Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Gani, J.
1971.
Recent work in epidemiology at Sheffield.
Advances in Applied Probability,
Vol. 3,
Issue. 2,
p.
204.
Gani, J.
1971.
Recent work in epidemiology at Sheffield.
Advances in Applied Probability,
Vol. 3,
Issue. 2,
p.
204.
Gani, J.
and
Jerwood, D.
1972.
The cost of a general stochastic epidemic.
Journal of Applied Probability,
Vol. 9,
Issue. 02,
p.
257.
Downton, F.
1972.
The area under the infectives trajectory of the general stochastic epidemic.
Journal of Applied Probability,
Vol. 9,
Issue. 2,
p.
414.
Downton, F.
1972.
The area under the infectives trajectory of the general stochastic epidemic.
Journal of Applied Probability,
Vol. 9,
Issue. 02,
p.
414.
Jerwood, D.
1974.
The cost of a carrier-borne epidemic.
Journal of Applied Probability,
Vol. 11,
Issue. 4,
p.
642.
Jerwood, D.
1974.
The cost of a carrier-borne epidemic.
Journal of Applied Probability,
Vol. 11,
Issue. 04,
p.
642.
Gani, J.
1975.
Ecole d’Eté de Probabilités de Saint-Flour IV—1974.
Vol. 480,
Issue. ,
p.
189.
Puri, Prem S.
1975.
Statistical Inference and Related Topics.
p.
235.
Lefevre, C.
1988.
A Markov Chain Model of Population Growth with an Application in Epidemiology.
Biometrical Journal,
Vol. 30,
Issue. 2,
p.
165.
Ball, Frank
and
Donnelly, Peter
1995.
Strong approximations for epidemic models.
Stochastic Processes and their Applications,
Vol. 55,
Issue. 1,
p.
1.
Gani, J.
and
Swift, R. J.
2008.
A Simple Approach to the Integrals under Three Stochastic Processes.
Journal of Statistical Theory and Practice,
Vol. 2,
Issue. 4,
p.
559.
Orsingher, Enzo
and
Polito, Federico
2013.
On the integral of fractional Poisson processes.
Statistics & Probability Letters,
Vol. 83,
Issue. 4,
p.
1006.
Khaluf, Yara
and
Dorigo, Marco
2016.
Modeling Robot Swarms Using Integrals of Birth-Death Processes.
ACM Transactions on Autonomous and Adaptive Systems,
Vol. 11,
Issue. 2,
p.
1.
Crawford, Forrest W.
Ho, Lam Si Tung
and
Suchard, Marc A.
2018.
Computational methods for birth‐death processes.
WIREs Computational Statistics,
Vol. 10,
Issue. 2,
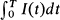 where T is the duration of the epidemic.
where T is the duration of the epidemic.