Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-11-25T04:06:21.374Z Has data issue: false hasContentIssue false
  • English
  • Français

The M/M/1 queue with mass exodus and mass arrivals when empty

Part of: Special processes Markov processes

Published online by Cambridge University Press:  14 July 2016

Anyue Chen  and
Eric Renshaw
Anyue Chen*
Affiliation:
University of Greenwich
Eric Renshaw*
Affiliation:
University of Strathclyde
*
∗Postal address: School of Computing and Mathematical Sciences, University of Greenwich, Wellington Street, Woolwich, London SE18 6PF, UK.
∗∗Postal address: Department of Statistics and Modelling Science, Livingstone Tower, University of Strathclyde, 26 Richmond Street, Glasgow Gl 1XH, UK.
Get access Rights & Permissions [Opens in a new window]

Abstract

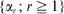An M/M/1 queue is subject to mass exodus at rate β and mass immigration at rate when idle. A general resolvent approach is used to derive occupation probabilities and high-order moments. This powerful technique is not only considerably easier to apply than a standard direct attack on the forward p.g.f. equation, but it also implicitly yields necessary and sufficient conditions for recurrence, positive recurrence and transience.

Keywords

M/M/1 QUEUECATASTROPHEMASS IMMIGRATIONQ-PROCESSRECURRENCETRANSIENCEIDLE PROBABILITYEQUILIBRIUM PROBABILITIESFACTORIAL MOMENTS

MSC classification

Primary: 60J35: Transition functions, generators and resolvents
Secondary: 60K25: Queueing theory
Type
Research Papers
Information
Journal of Applied Probability , Volume 34 , Issue 1 , March 1997 , pp. 192 - 207
Copyright
Copyright © Applied Probability Trust 1997 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abramowitz, M. and Stegun, A. I. (1965) Handbook of Mathematical Functions. Dover, New York.Google Scholar
Anderson, W. J. (1991) Continuous Time Markov Chains. Springer, Berlin.CrossRefGoogle Scholar
Asmussen, S. (1987) Applied Probability and Queues. Wiley, New York.Google Scholar
Chen, A. Y. and Renshaw, E. (1990) Markov branching processes with instantaneous immigration. Prob. Theory Rel. Fields 87, 209–240.CrossRefGoogle Scholar
Chen, A. Y. and Renshaw, E. (1993a) Existence and uniqueness criteria for conservative uni-instantaneous denumerable Markov processes. Prob. Theory Rel. Fields 94, 427–156.CrossRefGoogle Scholar
Chen, A. Y. and Renshaw, E. (1993b) Recurrence of Markov branching processes with immigration. Stoch. Proc. Appl. 45, 231–242.CrossRefGoogle Scholar
Chen, A. Y. and Renshaw, E. (1995) Markov branching processes regulated by large immigration and emigration. Stoch. Proc. Appl. 57, 339–359.CrossRefGoogle Scholar
Cox, D. R. and Miller, H. D. (1965) Theory of Stochastic Processes. Methuen, London.Google Scholar
Gross, D. and Harris, C. M. (1985) Fundamentals of Queueing Theory. Wiley, New York.Google Scholar
Hou, Z. T. and Guo, Q. F. (1988) Homogeneous Denumerable Markov Processes. Springer, Berlin.Google Scholar
Kleinrock, I. (1975) Queueing Systems. Vol. 1. Wiley, New York.Google Scholar
Parthasarathy, P. R. and Krishna Kumar, B. (1991) Density-dependent birth and death process with state-dependent immigration. Math. Comput. Modelling 15, 11–16.CrossRefGoogle Scholar
Renshaw, E. and Chen, A. Y. (1996) Birth-death processes with mass annihilation and state-dependent immigration. Stoch. Models (to appear).CrossRefGoogle Scholar
Saaty, T. L. (1961) Elements of Queueing Theory. McGraw Hill, New York.Google Scholar
Sharma, O. P. (1990) Markovian Queues. Ellis Horwood, New York.Google Scholar
Wang, T. K. and Yang, X. Q. (1992) Birth and Death Processes and Markov Chains. Springer, Berlin.Google Scholar
Yang, W. Q. (1990) The Construction Theory of Denumerable Markov Processes. Wiley, New York.Google Scholar