Published online by Cambridge University Press: 14 July 2016
A second guesser, G2, proposes to guess ‘smaller' or ‘larger' on each of n contests, i, according as 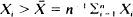 , or
, or 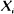 where Xi, i = 1, ···, n are the revealed guesses of the first guesser, G1. G2 wins contest i if his assertion about the size of the target quantity θi, is more accurate than that of G1, Xi, i = 1, ···, n. Otherwise G 1 is the winner. Laws of large numbers are derived for G2's win totals for arbitrary configurations of θi. Limiting distributions are obtained for the first guesser's win totals in two special cases, the first where the θi are highly concentrated and the second where they are widely dispersed. G2 enjoys an enormous advantage over G1 in the former case, as intuition would suggest.
where Xi, i = 1, ···, n are the revealed guesses of the first guesser, G1. G2 wins contest i if his assertion about the size of the target quantity θi, is more accurate than that of G1, Xi, i = 1, ···, n. Otherwise G 1 is the winner. Laws of large numbers are derived for G2's win totals for arbitrary configurations of θi. Limiting distributions are obtained for the first guesser's win totals in two special cases, the first where the θi are highly concentrated and the second where they are widely dispersed. G2 enjoys an enormous advantage over G1 in the former case, as intuition would suggest.
This research was supported by the National Science Foundation of the United States under grants NSF MCS-80-03568 and NSF MCS-782-4175.