Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Massé, Bruno
1999.
On the variance of the number of extreme points of a random convex hull.
Statistics & Probability Letters,
Vol. 44,
Issue. 2,
p.
123.
Massé, Bruno
2000.
On the LLN for the number of vertices of a random convex hull.
Advances in Applied Probability,
Vol. 32,
Issue. 3,
p.
675.


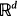 is investigated. We obtain, in particular, an explicit and distribution-independent bound.
is investigated. We obtain, in particular, an explicit and distribution-independent bound.