Published online by Cambridge University Press: 14 July 2016
The centred, periodic, stationary Gaussian process X(z), ≧ z ≧ 1 with covariances 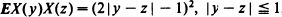 , appears when one studies the solutions of the vibrating string equation forced by noise, corresponding to the case of a finite string with the extremes tied together. The close relationship between this process and a Brownian bridge permits us to compute the distribution of the maximum excursion of the string at particular times.
, appears when one studies the solutions of the vibrating string equation forced by noise, corresponding to the case of a finite string with the extremes tied together. The close relationship between this process and a Brownian bridge permits us to compute the distribution of the maximum excursion of the string at particular times.