Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-26T13:43:07.795Z Has data issue: false hasContentIssue false
  • English
  • Français

Study of the Normal Variational Equation in an Homogeneous Field of Degree Five

Published online by Cambridge University Press:  12 April 2016

A. Makhlouf  and
N. Debbah
A. Makhlouf
Affiliation:
Institute of mathematics, BP 12 El-Hadhar, University of Annaba, Algeria
N. Debbah
Affiliation:
Institute of mathematics, BP 12 El-Hadhar, University of Annaba, Algeria

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We study the normal variational equation of degree five:

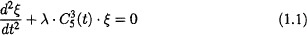
where λ is a parameter and C5(t) verifies

The solution C5(t) of (1.2) is the inverse function of

We prove that the pole t5 of C5(t) defined by 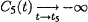is given by

where

Type
Extended Abstracts
Information
International Astronomical Union Colloquium , Volume 172: Impact of Modern Dynamics in Astronomy , 1999 , pp. 459 - 460
Copyright
Copyright © Kluwer 1999

References

Makhlouf, A. and Nahon, F.: 1991, On a family of Hill’s equation in the complex field, Celest. Mech., 52, 227–291.Google Scholar
Lainé, A.: Précis d’analyse mathématique, Tome 1, p. 213.Google Scholar
Yoshida, H.: 1986, Existence of exponentially unstable periodic solution and the non integrability of homogeneous Hamiltonian systems, Physica D, 21 Google Scholar
Yoshida, H.: 1987, A criterium for the non existence of an additional integral in systems with an homogeneous potential, Physica D, 29 Google Scholar