Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-23T16:02:20.906Z Has data issue: false hasContentIssue false
  • English
  • Français

Dynamical Instabilities in Stars

Published online by Cambridge University Press:  15 February 2018

P. Ledoux
P. Ledoux*
Affiliation:
Institut d’Astrophysique, Université de Liège

Summary

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The linear dynamical instability at the origin of convection in stars is reviewed and shown to depend essentially on the sign of 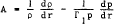which is the usual argument of convection criteria. The case of two or more superadiabatic regions separated by subadiabatic ones might well deserve more detailed attention.

Once this instability is partially removed by the setting in of convection its effects must be balanced by dissipation terms if a stationary state is to result. This yields the value of a Rayleigh number.

If energy generation is included in the non-conservative terms, possibilities are somewhat enriched including a case of dynamical instability in presence of A<0 (usually stable) but very small in absolute value.

Type
II. Linear Theory
Information
International Astronomical Union Colloquium , Volume 38: Problems of Stellar Convection , 1977 , pp. 87 - 102
Copyright
Copyright © 1976

References

Biermann, L. 1939, Z. Astrophys., 1838, 344 Google Scholar
Chandrasekhar, S. 1964, Astrophys. J., 13938, 664 Google Scholar
Cowling, T.G. 1941, Mon. Not. Roy. Astr. Soc., 10138, 367 CrossRefGoogle Scholar
Defouw, R.J. 1970, Astrophys. J., 16038, 659 Google Scholar
Deupree, R.G. 1974, Astrophys. J., 19438, 393 Google Scholar
Deupree, R.G. 1975a, Astrophys. J., 19838, 419 Google Scholar
Deupree, R.G. 1975b, Astrophys. J., 20138, 183 Google Scholar
Deupree, R.G. 1976, Astrophys. J., 20538, 286 Google Scholar
Goosens, M. and Smeyers, P. 1974, Astrophys. Space Sci., 2638, 137 Google Scholar
Grisvard, P., Souffrin, P. and Zerner, M. 1972, Astron. and Astrophys., 1738, 309 Google Scholar
Islam, J.N. 1970, Mon. Not. Roy. Astr. Soc., 15038, 237 Google Scholar
Kovetz, A. 1967, Z. Astrophys., 6638, 446 Google Scholar
Langer, R.E. 1959, Trans. Amer. Math. Soc., 9038, 113 Google Scholar
Lebovitz, N.R. 1965a, Astrophys. J., 14238, 229 Google Scholar
Lebovitz, N.R. 1965b, Astrophys. J., 14238, 1257 Google Scholar
Lebovitz, N.R. 1966, Astrophys. J., 14638, 946 Google Scholar
Ledoux, P. 1949, Contribution à l’étude de la structure interne des étoiles et de leur stabilité, Mém. Soc. Roy. Sci. Liège Coll. in -8°, 4°sér. T IX, Ch. III, sect. 4, 5, 6Google Scholar
Ledoux, P. et Smeyers, P. 1966, Compt. Rend. Acad. Sci. Paris, Sér.B., 26238, 841 Google Scholar
Ledoux, P. 1974, in Ledoux, P. et al (ed.) Stellar Instability and Evolution, IAU Symposium n°59, Part VI, p. 135 Google Scholar
Moulton, F.R. 1909, Astrophys. J., 2938, 257 Google Scholar
Nordlund, Å. 1976, Astron. and Astrophys., 5038, 23 Google Scholar
Olver, F.W.J. 1975, Phil. Trans. Roy. Soc. London A, 27838, 137 Google Scholar
Osaki, Y. 1975, Publ. Astron. Soc. Japan, 2738, 237 Google Scholar
Pekeris, C.L. 1938, Astrophys. J., 8838, 189 Google Scholar
Schatzman, E. 1946, Ann. Astrophys., 938, 199 Google Scholar
Scuflaire, R. 1974, Astron. and Astrophys., 3438, 449 Google Scholar
Shapley, H. 1914, Astrophys. J., 4038, 448 Google Scholar
Spiegel, E.A. 1971, Ann. Rev. Astron. Astrophys. 938, 323 Google Scholar
Spiegel, E.A. 1972, Ann. Rev. Astron. Astrophys., 1038, 261 Google Scholar
Tassoul, M. and Tassoul, J.L. 1968, Ann. Astrophys., 3138, 251 Google Scholar
Thomson, W. 1863, Phil. Trans. Roy. Soc. London, 15338, 612 Google Scholar
Thorne, K.S. 1966, Astrophys. J., 14438, 201 CrossRefGoogle Scholar