Article contents
TOPOLOGICAL 4-MANIFOLDS WITH 4-DIMENSIONAL FUNDAMENTAL GROUP
Published online by Cambridge University Press: 23 July 2021
Abstract
Let 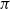 $\pi$ be a group satisfying the Farrell–Jones conjecture and assume that
$\pi$ be a group satisfying the Farrell–Jones conjecture and assume that  $B\pi$ is a 4-dimensional Poincaré duality space. We consider topological, closed, connected manifolds with fundamental group
$B\pi$ is a 4-dimensional Poincaré duality space. We consider topological, closed, connected manifolds with fundamental group 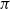 $\pi$ whose canonical map to
$\pi$ whose canonical map to 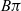 $B\pi$ has degree 1, and show that two such manifolds are s-cobordant if and only if their equivariant intersection forms are isometric and they have the same Kirby–Siebenmann invariant. If
$B\pi$ has degree 1, and show that two such manifolds are s-cobordant if and only if their equivariant intersection forms are isometric and they have the same Kirby–Siebenmann invariant. If  $\pi$ is good in the sense of Freedman, it follows that two such manifolds are homeomorphic if and only if they are homotopy equivalent and have the same Kirby–Siebenmann invariant. This shows rigidity in many cases that lie between aspherical 4-manifolds, where rigidity is expected by Borel’s conjecture, and simply connected manifolds where rigidity is a consequence of Freedman’s classification results.
$\pi$ is good in the sense of Freedman, it follows that two such manifolds are homeomorphic if and only if they are homotopy equivalent and have the same Kirby–Siebenmann invariant. This shows rigidity in many cases that lie between aspherical 4-manifolds, where rigidity is expected by Borel’s conjecture, and simply connected manifolds where rigidity is a consequence of Freedman’s classification results.
MSC classification
- Type
- Research Article
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press on behalf of Glasgow Mathematical Journal Trust
References
- 1
- Cited by


