Published online by Cambridge University Press: 18 May 2009
Let A be an n × n complex matrix and c = (c1… cn) єℂn. Define the c-numerical range of A to be the set 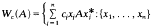 is an orthonormal set in
is an orthonormal set in 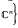 , where * denotes the conjugate transpose. Westwick [8[ proved that if c … cn are collinear, then Wc(A) is convex. (Poon [6] gave another proof.) But in general for n ≧3, Wc(A) may fail to be convex even for normal A (for example, see Marcus [4] or Lemma 3 in this note) though it is star-shaped (Tsing [7]). In the following, we shall assume that A is normal. Let W(A) = {diag UAU*: U is unitary}. Horn [3] proved that if the eigenvalues of A are collinear, then W(A) is convex. Au-Yeung and Sing [2] showed that the converse is also true. Marcus [4] further conjectured (and proved for n = 3) that if Wc(A) is convex for all cєℂn then the eigenvalues of A are collinear. Let λ = (λ1, …, λn єℂn. We denote by
, where * denotes the conjugate transpose. Westwick [8[ proved that if c … cn are collinear, then Wc(A) is convex. (Poon [6] gave another proof.) But in general for n ≧3, Wc(A) may fail to be convex even for normal A (for example, see Marcus [4] or Lemma 3 in this note) though it is star-shaped (Tsing [7]). In the following, we shall assume that A is normal. Let W(A) = {diag UAU*: U is unitary}. Horn [3] proved that if the eigenvalues of A are collinear, then W(A) is convex. Au-Yeung and Sing [2] showed that the converse is also true. Marcus [4] further conjectured (and proved for n = 3) that if Wc(A) is convex for all cєℂn then the eigenvalues of A are collinear. Let λ = (λ1, …, λn єℂn. We denote by  the vector λ1, …, λn and by [λ] the diagonal matrix with λ1, …, λn lying on its diagonal. Since, for any unitary matrix U,. Wc(A) = Wc (UAU*), the Marcus conjecture reduces to: if Wc([λ]) is convex for all c єℂn then λ1, … λn are collinear. For the case n = 3, Au-Yeung and Poon [1] gave a complete characterization on the convexity of the set Wc([λ]) in terms of the relative position of the points
the vector λ1, …, λn and by [λ] the diagonal matrix with λ1, …, λn lying on its diagonal. Since, for any unitary matrix U,. Wc(A) = Wc (UAU*), the Marcus conjecture reduces to: if Wc([λ]) is convex for all c єℂn then λ1, … λn are collinear. For the case n = 3, Au-Yeung and Poon [1] gave a complete characterization on the convexity of the set Wc([λ]) in terms of the relative position of the points  , where σ є S3 the permutation group of order 3. As an example they showed that if λ1, λ2, λ3 are not collinear, then
, where σ є S3 the permutation group of order 3. As an example they showed that if λ1, λ2, λ3 are not collinear, then  is not convex (Lemma 3 in this note gives another proof). We shall show that for the case n = 4,
is not convex (Lemma 3 in this note gives another proof). We shall show that for the case n = 4,  is not convex if λ1, λ2. λ3. λ4 are not collinear. Thus for n = 3, 4 the Marcus conjecture is answered and improved.
is not convex if λ1, λ2. λ3. λ4 are not collinear. Thus for n = 3, 4 the Marcus conjecture is answered and improved.