Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-23T02:40:13.405Z Has data issue: false hasContentIssue false
  • English
  • Français

ON BRANNAN'S COEFFICIENT CONJECTURE AND APPLICATIONS

Published online by Cambridge University Press:  01 January 2007

STEPHAN RUSCHEWEYH  and
LUIS SALINAS
STEPHAN RUSCHEWEYH
Affiliation:
Mathematisches Institut, Universität Würzburg, D-97074 Würzburg, Germany e-mail: [email protected]
LUIS SALINAS
Affiliation:
Departamento de Informática, Universidad Técnica F. Santa María, Valparaíso, Chile e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract.

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

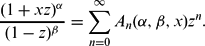D. Brannan's conjecture says that for 0 <α,β≤1, |x|=1, and n∈N one has |A2n−1(α,β,x)|≤|A2n−1(α,β,1)|, where We prove this for the case α=β, and also prove a differentiated version of the Brannan conjecture. This has applications to estimates for Gegenbauer polynomials and also to coefficient estimates for univalent functions in the unit disk that are ‘starlike with respect to a boundary point’. The latter application has previously been conjectured by H. Silverman and E. Silvia. The proofs make use of various properties of the Gauss hypergeometric function.

Keywords

Primary 30C50Secondary 33C0533C45
Type
Research Article
Information
Glasgow Mathematical Journal , Volume 49 , Issue 1 , January 2007 , pp. 45 - 52
Copyright
Copyright © Glasgow Mathematical Journal Trust 2007

References

REFERENCES

1.Abramowitz, M. and Stegun, I.A., editors, Handbook of mathematical functions (Dover, New York, 1970).Google Scholar
2.Aharonov, D. and Friedland, S., On an inequality connected with the coefficient conjecture for functions of bounded boundary rotation, Ann. Acad. Sci. Fenn., Ser. A1 Math. 524 (1972), 14 pp.Google Scholar
3.Barnard, R.W., Pearce, K. and Wheeler, W., On a coefficient conjecture of Brannan, Complex Variables 33 (1997), 51–61.Google Scholar
4.Brannan, D., {On coefficient problems for certain power series}, Symposium on Complex Analysis, Canterbury, 1973, eds. J.G. Clunie and W.K. Hayman, London Math. Soc. Lecture Notes Series {No. 12} (1974), 17–27.Google Scholar
5.Brannan, D., Clunie, J. G. and Kirwan, W. E., On the coefficient problem for functions of bounded rotation, Ann. Acad. Sci. Fenn., Ser. A1 Math. 523 (1973), 18 pp.Google Scholar
6.Geisler, R., Sternförmige Funktionen bezüglich eines Randpunktes, Diplomarbeit (Würzburg University, 2005).Google Scholar
7.Milcetich, J. G., On a coefficient conjecture of Brannan, J. Math. Anal. Appl. 139 (1989), 515–522.Google Scholar
8.Silverman, H. and Silvia, E., Subclasses of univalent functions starlike with respect to a boundary point, Houston J. Math. 16 (1990), 289–300.Google Scholar
9.Todorov, P., Three explicit formulas for the Taylor coefficients of , Abh. Math. Seminar Univ. Hamb. 65 (1995), 147–153.Google Scholar