Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-26T02:02:36.941Z Has data issue: false hasContentIssue false
  • English
  • Français

On approximating Lebesgue integrals by Riemann sums

Published online by Cambridge University Press:  18 May 2009

Szilárd GY. Révész  and
Imre Z. Ruzsa
Szilárd GY. Révész
Affiliation:
Mathematical Research Institute of the Hungarian Academy of Sciences, Budapest, Reáltanoda u. 13–15, POB 127, H-1364Hungary
Imre Z. Ruzsa
Affiliation:
Mathematical Research Institute of the Hungarian Academy of Sciences, Budapest, Reáltanoda u. 13–15, POB 127, H-1364Hungary
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

If f is a real function, periodic with period 1, we define

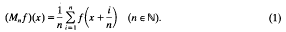
In the whole paper we write ∫ for , mE for the Lebesgue measure of E ∩ [0,1], where E ⊂ ℝ is any measurable set of period 1, and we also use XE for the characteristic function of the set E. Consistent with this, the meaning of ℒp is ℒp [0, 1]. For all real xwe have

if f is Riemann-integrable on [0, 1]. However,∫ f exists for all f ∈ ℒ1 and one would wish to extend the validity of (2). As easy examples show, (cf. [3], [7]), (2) does not hold for f ∈ ℒp in general if p < 2. Moreover, Rudin [4] showed that (2) may fail for all x even for the characteristic function of an open set, and so, to get a reasonable extension, it is natural to weaken (2) to

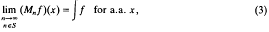
where S ⊂ ℕ is some “good” increasing subsequence of ℕ. Naturally, for different function classes ℱ ⊂ ℒ1 we get different meanings of being good. That is, we introduce the class of ℱ-good sequences as

Type
Research Article
Information
Glasgow Mathematical Journal , Volume 33 , Issue 2 , May 1991 , pp. 129 - 134
Copyright
Copyright © Glasgow Mathematical Journal Trust 1991

References

REFERENCES

1.Jessen, B., On the approximation of Lebesgue integrals by Riemann sums, Ann. of Math. 35 (1934), 248–251.CrossRefGoogle Scholar
2.Jessen, B., The theory of integration in a space of an infinite number of dimensions, Acta Math. 63 (1934), 249–323.CrossRefGoogle Scholar
3.Marczinkiewicz, J. and Zygmund, A., Mean values of trigonometrical polynomials, Fund. Math. 28 (1937), 131–166.CrossRefGoogle Scholar
4.Rudin, W., An arithmetic property of Riemann sums, Proc. Amer. Math. Soc. 15 (1964), 321–324.CrossRefGoogle Scholar
5.Salem, R., Sur les sommes Riemanniennes des fonctions sommables, Mat. Tidsskr. B 1948 (1948), 60–62.Google Scholar
6.Sauer, N., On the density of families of sets, J. Combin. Theory Ser. A. 13 (1972), 145–147.CrossRefGoogle Scholar
7.Ursell, H. D., On the behaviour of a certain sequence of functions derived from a given one, J. London Math. Soc. 12 (1937), 229–232.CrossRefGoogle Scholar