Hostname: page-component-586b7cd67f-2plfb Total loading time: 0 Render date: 2024-11-23T01:16:57.343Z Has data issue: false hasContentIssue false
  • English
  • Français

Numerical ranges of powers of hermitian elements

Published online by Cambridge University Press:  18 May 2009

M. J. Crabb  and
C. M. McGregor
M. J. Crabb
Affiliation:
University of Glasgow
C. M. McGregor
Affiliation:
University of Glasgow
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

An element k of a unital Banach algebra A is said to be Hermitian if its numerical range

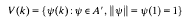
is contained in ℝ; equivalently, ∥eitk∥ = 1(t ∈ ℝ)—see Bonsall and Duncan [3] and [4]. Here we find the largest possible extent of V(kn), n ∈ ℕ, given V(k) ⊆ [−1, 1], and so ∥k∥ ≤ 1: previous knowledge is in Bollobás [2] and Crabb, Duncan and McGregor [7]. The largest possible sets all occur in a single example. Surprisingly, they all have straight line segments in their boundaries. The example is in [2] and [7], but here we give A. Browder's construction from [5], partly published in [6]. We are grateful to him for a copy of [5], and for discussions which led to the present work. We are also grateful to J. Duncan for useful discussions.

Type
Research Article
Information
Glasgow Mathematical Journal , Volume 28 , Issue 1 , January 1986 , pp. 37 - 45
Copyright
Copyright © Glasgow Mathematical Journal Trust 1986

References

REFERENCES

1.Boas, R. P., Entire functions (Academic Press, 1954).Google Scholar
2.Bollobás, B., The numerical range in Banach algebras and complex functions of exponential type, Bull. London Math. Soc. 3 (1971), 27–33.CrossRefGoogle Scholar
3.Bonsall, F. F. and Duncan, J., Numerical ranges of operators on normed spaces and elements of normed algebras. London Math. Soc. Lecture Notes 2 (Cambridge University Press, 1971).CrossRefGoogle Scholar
4.Bonsall, F. F. and Duncan, J., Numerical ranges II, London Math. Soc. Lecture Notes 10 (Cambridge University Press, 1973).CrossRefGoogle Scholar
5.Browder, A., States, Numerical ranges, etc., Proc. Brown Informal analysis Seminar, 1969.Google Scholar
6.Browder, A., On Bernstein's inequality and the norm of Hermitian operators, Amer. Math. Monthly 78 (1971), 871–873.CrossRefGoogle Scholar
7.Crabb, M. J., Duncan, J. and McGregor, C. M., Some extremal problems in the theory of numerical ranges, Acta Math. 128 (1972), 123–142.CrossRefGoogle Scholar
8.Halmos, P. R., A Hilbert Space Problem book, (Van Nostrand, 1967).Google Scholar
9.Hardy, G. H., A Course of Pure Mathematics, (Cambridge, ed. 5, 1928).Google Scholar
10.Luke, Y. L., Integrals of Bessel functions, (McGraw–Hill, 1962).Google Scholar