In [1, Corollary 5], Figiel gives an elegant demonstration that the modulus ofconvexity δ in real Banach space X is nondecreasing, where

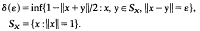
It is deduced from this that in fact δ(ɛ)/ɛ is nondecreasing [Proposition 3]. During the course of the proof [Lemma 4] it is stated that if v ∊ Sx is a local maximum on Sx of φ ∈Sx*, then v is a global maximum (φ(v) = 1). This is false; it could be that v is a global minimum. It is easy to construct such an example in R2 endowed with the maximum norm. What is true is that v is a global maximum of |φ|.