Hostname: page-component-78c5997874-xbtfd Total loading time: 0 Render date: 2024-11-19T10:37:31.294Z Has data issue: false hasContentIssue false
  • English
  • Français

A note on Cauchy's root test

Published online by Cambridge University Press:  18 May 2009

I. S. Murphy
I. S. Murphy
Affiliation:
University of Glasgow, Scotland
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Throughout this note we deal with a series Σan of positive terms. The following tests for the convergence of this series are well-known.

Test 1. (Ratio test). Let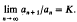Then, if K < 1, Σanconverges, while if K > 1, Σandiverges.

Test 2. (Root test). LetThen, if K < 1, Σanconverges, while if K > 1, Σandiverges.

Type
Research Article
Information
Glasgow Mathematical Journal , Volume 17 , Issue 2 , July 1976 , pp. 151 - 154
Copyright
Copyright © Glasgow Mathematical Journal Trust 1976

References

REFERENCES

1.Bromwich, T. J. I'A., Infinite series (Macmillan, 1908).Google Scholar
2.Knopp, K., Theory and application of infinite series (Blackie, 1928).Google Scholar
3.Phillips, E. G., A course of analysis (Cambridge, 1962).Google Scholar
4.Rankin, R. A., An introduction to mathematical analysis (Pergamon, 1963).Google Scholar