Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Jevtić, Miroljub
and
Karapetrović, Boban
2019.
Generalized Hilbert Matrices Acting on Spaces that are Close to the Hardy Space
$$H^1$$
H
1
and to the Space BMOA.
Complex Analysis and Operator Theory,
Vol. 13,
Issue. 5,
p.
2357.
Karapetrović, Boban
2021.
Hilbert Matrix and Its Norm on Weighted Bergman Spaces.
The Journal of Geometric Analysis,
Vol. 31,
Issue. 6,
p.
5909.
Lindström, M.
Miihkinen, S.
and
Norrbo, D.
2022.
Exact essential norm of generalized Hilbert matrix operators on classical analytic function spaces.
Advances in Mathematics,
Vol. 408,
Issue. ,
p.
108598.
Bralović, Dušica
and
Karapetrović, Boban
2022.
New Upper Bound for the Hilbert Matrix Norm on Negatively Indexed Weighted Bergman Spaces.
Bulletin of the Malaysian Mathematical Sciences Society,
Vol. 45,
Issue. 3,
p.
1183.
Karapetrović, Boban
2023.
Function Spaces, Theory and Applications.
Vol. 87,
Issue. ,
p.
245.
Dmitrović, Dušica
and
Karapetrović, Boban
2023.
On the Hilbert matrix norm on positively indexed weighted Bergman spaces.
Revista de la Real Academia de Ciencias Exactas, Físicas y Naturales. Serie A. Matemáticas,
Vol. 117,
Issue. 4,
Ye, Shanli
and
Feng, Guanghao
2023.
Norm of the Hilbert Matrix on Logarithmically Weighted Bergman Spaces.
Complex Analysis and Operator Theory,
Vol. 17,
Issue. 6,
Bao, Guanlong
Guo, Kunyu
Sun, Fangmei
and
Wang, Zipeng
2024.
Hankel Matrices Acting on the Dirichlet Space.
Journal of Fourier Analysis and Applications,
Vol. 30,
Issue. 5,
Dai, Jineng
2024.
On the norm of the Hilbert matrix operator on weighted Bergman spaces.
Journal of Functional Analysis,
Vol. 287,
Issue. 9,
p.
110587.
Norrbo, David
2025.
Norm estimates for the Hilbert matrix operator on weighted Bergman spaces.
Journal of Mathematical Analysis and Applications,
Vol. 548,
Issue. 2,
p.
129408.
Bellavita, C.
Daskalogiannis, V.
Miihkinen, S.
Norrbo, D.
Stylogiannis, G.
and
Virtanen, J.
2025.
Generalized Hilbert matrix operators acting on Bergman spaces.
Journal of Functional Analysis,
Vol. 288,
Issue. 9,
p.
110856.
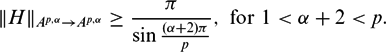 \begin{equation*}
\|H\|_{A^{p,\alpha}\rightarrow A^{p,\alpha}}\geq\frac{\pi}{\sin{\frac{(\alpha+2)\pi}{p}}}, \,\, \textnormal{for} \,\, 1<\alpha+2<p.
\end{equation*}
\begin{equation*}
\|H\|_{A^{p,\alpha}\rightarrow A^{p,\alpha}}\geq\frac{\pi}{\sin{\frac{(\alpha+2)\pi}{p}}}, \,\, \textnormal{for} \,\, 1<\alpha+2<p.
\end{equation*} $\frac{\pi}{\sin{\frac{(\alpha+2)\pi}{p}}}$, while if 2 ≤ α +2 < p < 2(α+2), upper bound for the norm ∥H∥Ap,α → Ap,α, better then known, is obtained.
$\frac{\pi}{\sin{\frac{(\alpha+2)\pi}{p}}}$, while if 2 ≤ α +2 < p < 2(α+2), upper bound for the norm ∥H∥Ap,α → Ap,α, better then known, is obtained.