Let f be an analytic function on a connected open set Ώ in the complex plane. Then, for given a, b ∈ Ώ, the equation

need not have a solution z ∈ Ώ. As a matter of fact, this would happen with each locally one-to-one analytic function which is not one-to-one on Ώ. But if we fix a a ∈ Ώ, then, for all b sufficiently close to a, (1) is solvable for z. This is an easy consequence of the Open Mapping Theorem applied to f'. For, assuming that f' is non-constant (otherwise, (1) holds for all a, b, z ∈Ώ), the Open Mapping Theorem tells us that f'(Ώ), the image under f' of Ώ, is an open neighbourhood of f'(a); so it is a direct consequence of the definition of f'(a) that there exists δ > 0 such that 0 < |b – a| < δ implies (f(b) – f(a))/(b – a) ∈f'(Ώ). A stronger statement has been obtained by J. M. Robertson [1, p. 329], who has shown that

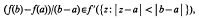
and, if f''(a) ≠ 0, then

.
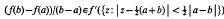