Hostname: page-component-78c5997874-ndw9j Total loading time: 0 Render date: 2024-11-19T10:22:04.970Z Has data issue: false hasContentIssue false
  • English
  • Français

The lattice of inverse subsemigroups of a reduced inverse semigroup†

Published online by Cambridge University Press:  18 May 2009

P. R. Jones
P. R. Jones
Affiliation:
Department of Mathematics, Monash University, Clayton, Victoria, Australia,3168
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

An inverse semigroup R is said to be reduced (or proper) if ℛ∩σ= i (where σ is the minimum group congruence on R). McAlister has shown ([3], [4]) that every reduced inverse semigroup is isomorphic with a “P-semigroup” P(G, 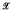, 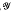), for some semilattice 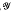, poset 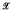containing 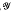as an ideal, and group G acting on 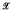by order-automorphisms; (and, conversely, every P-semigroup is reduced). In [4], he also found the morphisms between P-semigroups, in terms of morphisms between the respective groups, and between the respective posets.

Type
Research Article
Information
Glasgow Mathematical Journal , Volume 17 , Issue 2 , July 1976 , pp. 161 - 172
Copyright
Copyright © Glasgow Mathematical Journal Trust 1976

References

REFERENCES

1.Clifford, A. H. and Preston, G. B., The algebraic theory of semigroups (Providence, R.I., 1961, 1967).Google Scholar
2.Gluskin, L. M., Elementary generalized groups, Mat. Sb. 41 (83) (1957), 23–26 (Russian) (MR 19, #836).Google Scholar
3.McAlister, D. B., Groups, semilattices and inverse semigroups, Trans. Amer. Math. Soc. 192 (1974), 227–244.Google Scholar
4.McAlister, D. B., Groups, semilattices and inverse semigroups, II, Trans. Amer. Math. Soc. 196 (1974), 351–370.Google Scholar
5.McAlister, D. B. and McFadden, R., Zig-zag representations and inverse semigroups, J. Algebra 32 (1974), 178–206.Google Scholar
6.Munn, W. D., A class of irreducible matrix representations of an arbitrary inverse semigroup, Proc. Glasgow Math. Assoc. 5 (1961), 41–48.CrossRefGoogle Scholar
7.O'carroll, L., A note on free inverse semigroups, Proc. Edinburgh Math. Soc. 19 (1974), 17–24.CrossRefGoogle Scholar
8.Preston, G. B., Inverse semigroups, J. London Math. Soc. 29 (1954), 396–403.Google Scholar
9.Reilly, N. R. and Scheiblich, H. E., Congruences on regular semigroups, Pacific J. Math. 23 (1967), 349–360.Google Scholar