Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Kittaneh, Fuad
1986.
Inequalities for the Schattenp-norm. IV.
Communications In Mathematical Physics,
Vol. 106,
Issue. 4,
p.
581.
Kittaneh, Fuad
1986.
On zero-trace commutators.
Bulletin of the Australian Mathematical Society,
Vol. 34,
Issue. 1,
p.
119.
Kittaneh, Fuad
1986.
Inequalities for the Schattenp-norm. III.
Communications in Mathematical Physics,
Vol. 104,
Issue. 2,
p.
307.
Kittaneh, Fuad
1987.
Inequalities for the Schatten p-norm II.
Glasgow Mathematical Journal,
Vol. 29,
Issue. 1,
p.
99.
Howe, Roger
2012.
Mathematical Methods in Systems, Optimization, and Control.
p.
221.
Wang, Xiaoqian
Liu, Kefei
Yan, Jingwen
Risacher, Shannon L.
Saykin, Andrew J.
Shen, Li
and
Huang, Heng
2017.
Information Processing in Medical Imaging.
Vol. 10265,
Issue. ,
p.
198.
Aziznejad, Shayan
and
Unser, Michael
2021.
Duality Mapping for Schatten Matrix Norms.
Numerical Functional Analysis and Optimization,
Vol. 42,
Issue. 6,
p.
679.
Salvia, Raffaele
and
Giovannetti, Vittorio
2021.
On the distribution of the mean energy in the unitary orbit of quantum states.
Quantum,
Vol. 5,
Issue. ,
p.
514.
Cirio, Mauro
Luo, Si
Liang, Pengfei
Nori, Franco
and
Lambert, Neill
2024.
Modeling the unphysical pseudomode model with physical ensembles: Simulation, mitigation, and restructuring of non-Markovian quantum noise.
Physical Review Research,
Vol. 6,
Issue. 3,
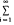 si(A)p <∞, we say that A is in the Schatten p-class Cp and ∥A∥p=
si(A)p <∞, we say that A is in the Schatten p-class Cp and ∥A∥p=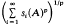 1/p is the p-norm of A. Hence, C1 is the trace class, C2 is the Hilbert–Schmidt class, and C∞ is the ideal of compact operators K(H).
1/p is the p-norm of A. Hence, C1 is the trace class, C2 is the Hilbert–Schmidt class, and C∞ is the ideal of compact operators K(H).