No CrossRef data available.
 $$\sum\limits_{l + km = n} {\sigma (l)\sigma (m)} $$ AND
$$\sum\limits_{l + km = n} {\sigma (l)\sigma (m)} $$ AND  $$\sum\limits_{al + bm = n} {\sigma (l)\sigma (m)} $$ FOR k = a · b = 21, 33, AND 35
$$\sum\limits_{al + bm = n} {\sigma (l)\sigma (m)} $$ FOR k = a · b = 21, 33, AND 35Published online by Cambridge University Press: 16 July 2021
The article focuses on the evaluation of convolution sums 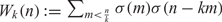 $${W_k}(n): = \mathop \sum \nolimits_{_{m < {n \over k}}} \sigma (m)\sigma (n - km)$$ involving the sum of divisor function
$${W_k}(n): = \mathop \sum \nolimits_{_{m < {n \over k}}} \sigma (m)\sigma (n - km)$$ involving the sum of divisor function  $$\sigma (n)$$ for k =21, 33, and 35. In this article, our aim is to obtain certain Eisenstein series of level 21 and use them to evaluate the convolution sums for level 21. We also make use of the existing Eisenstein series identities for level 33 and 35 in evaluating the convolution sums for level 33 and 35. Most of the convolution sums were evaluated using the theory of modular forms, whereas we have devised a technique which is free from the theory of modular forms. As an application, we determine a formula for the number of representations of a positive integer n by the octonary quadratic form
$$\sigma (n)$$ for k =21, 33, and 35. In this article, our aim is to obtain certain Eisenstein series of level 21 and use them to evaluate the convolution sums for level 21. We also make use of the existing Eisenstein series identities for level 33 and 35 in evaluating the convolution sums for level 33 and 35. Most of the convolution sums were evaluated using the theory of modular forms, whereas we have devised a technique which is free from the theory of modular forms. As an application, we determine a formula for the number of representations of a positive integer n by the octonary quadratic form 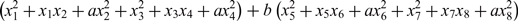 $$(x_1^2 + {x_1}{x_2} + ax_2^2 + x_3^2 + {x_3}{x_4} + ax_4^2) + b(x_5^2 + {x_5}{x_6} + ax_6^2 + x_7^2 + {x_7}{x_8} + ax_8^2)$$, for (a, b)=(1, 7), (1, 11), (2, 3), and (2, 5).
$$(x_1^2 + {x_1}{x_2} + ax_2^2 + x_3^2 + {x_3}{x_4} + ax_4^2) + b(x_5^2 + {x_5}{x_6} + ax_6^2 + x_7^2 + {x_7}{x_8} + ax_8^2)$$, for (a, b)=(1, 7), (1, 11), (2, 3), and (2, 5).