Hostname: page-component-6bf8c574d5-xtvcr Total loading time: 0 Render date: 2025-02-16T19:51:28.887Z Has data issue: false hasContentIssue false
  • English
  • Français

An extension of the van Hemmen–Ando norm inequality

Part of: General theory of linear operators Linear spaces and algebras of operators Special classes of linear operators

Published online by Cambridge University Press:  03 August 2022

Hamed Najafi
Hamed Najafi*
Affiliation:
Department of Pure Mathematics, Ferdowsi University of Mashhad, P.O. Box 1159, Mashhad 91775, Iran E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Let 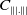$C_{\||.\||}$ be an ideal of compact operators with symmetric norm 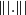$\||.\||$ . In this paper, we extend the van Hemmen–Ando norm inequality for arbitrary bounded operators as follows: if f is an operator monotone function on $[0,\infty)$ and S and T are bounded operators in $\mathbb{B}(\mathscr{H}\;\,)$ such that 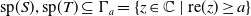${\rm{sp}}(S),{\rm{sp}}(T) \subseteq \Gamma_a=\{z\in \mathbb{C} \ | \ {\rm{re}}(z)\geq a\}$ , then

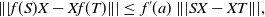\begin{equation*}\||f(S)X-Xf(T)\|| \leq\;f'(a) \ \||SX-XT\||,\end{equation*}
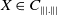for each $X\in C_{\||.\||}$ . In particular, if 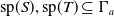${\rm{sp}}(S), {\rm{sp}}(T) \subseteq \Gamma_a$ , then
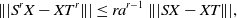\begin{equation*}\||S^r X-XT^r\|| \leq r a^{r-1} \ \||SX-XT\||,\end{equation*}
for each $X\in C_{\||.\||}$ and for each 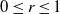$0\leq r\leq 1$ .

MSC classification

Primary: 47A56: Functions whose values are linear operators (operator and matrix valued functions, etc., including analytic and meromorphic ones)
Secondary: 47B47: Commutators, derivations, elementary operators, etc. 47L20: Operator ideals
Type
Research Article
Information
Glasgow Mathematical Journal , Volume 65 , Issue 1 , January 2023 , pp. 121 - 127
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press on behalf of Glasgow Mathematical Journal Trust

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aleksandrov, A. B. and Peller, V. V., Functions of perturbed unbounded self-adjoint operators. Operator Bernstein type inequalities, Indiana Univ. Math. J. 59 (4) (2010), 1451–1490.Google Scholar
Bendat, J. and Sherman, S., Monotone and convex operator functions, Trans. Am. Math. Soc. 79 (1955), 58–71.CrossRefGoogle Scholar
Bhatia, R., First and second order perturbation bounds for the operator absolute value, Linear Algebra Appl. 208 (1994), 367–376.Google Scholar
Gohberg, I. C. and Krein, M. G., Introduction to the theory of linear non-selfadjoint operators in Hilbert spaces (Nauka, Moscow, 1965).Google Scholar
Hansen, F., The fast track to Loewner’s theorem, Linear Algebra Appl. 438 (2013), 4557–4571.CrossRefGoogle Scholar
Hiai, F., Linear operators , Banach Center Publications, vol. 38 (Polish Academy of Sciences, Warszawa, 1997), 119–181 Google Scholar
Hiai, F. and Kosaki, H., Means of Hilbert space operators , Lecture Notes in Mathematics, vol. 1820 (Springer-Verlag, Berlin, 2003).Google Scholar
Kissin, E., Potapov, D., Shulman, V. and Sukochev, F., Operator smoothness in Schatten norms for functions of several variables: Lipschitz conditions, differentiability and unbounded derivations, Proc. London Math. Soc. 108 (3) (2014), 327–349.Google Scholar
Kissin, E. and Shulman, V. S., On fully operator Lipschitz functions, J. Funct. Anal. 253 (2007), 711–728.Google Scholar
Kittaneh, F. and Kosaki, H., Inequalities for the Schatten p-norm V, Publ. Res. Inst. Math. Sci. 23 (1986), 433–443.Google Scholar
Löwner, K., Über monotone Matrix funktionen, Math. Z. 38 (1934), 177–216.Google Scholar
Najafi, H., Some operator inequalities for Hermitian Banach $*$ -algebras, Math. Scand. (preprint).Google Scholar
Simon, B., Trace ideals and their applications (AMS, 2005).Google Scholar
Skripka, A. and Tomskova, A., Multilinear operator integrals: theory and applications, Lecture Notes in Mathematics, vol. 2250 (Springer International Publishing, 2019).Google Scholar
van Hemmen, J. L. and Ando, T., An inequality for trace ideals, Commun. Math. Phys. 76 (1980), 143–148.Google Scholar