Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-23T07:44:45.621Z Has data issue: false hasContentIssue false
  • English
  • Français

The reduction in fixation probability caused by substitutions at linked loci

Published online by Cambridge University Press:  14 April 2009

N. H. Barton
N. H. Barton
Affiliation:
Institute of Cell, Animal and Population Biology, University of Edinburgh, Kings' Buildings, Edinburgh EH9 3JT, UK
Rights & Permissions [Opens in a new window]

Summary

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

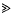The probability of fixation of a mutation with selective advantage s will be reduced by substitutions at other loci. The effect of a single substitution, with selective advantage , can be approximated as a sudden reduction in the frequency of the favourable allele, by a fraction w = 1 −(s/S)r/s (where r is the recombination rate). An expression for the effect of a given sequence of such catastrophes is derived. This also applies to the ecological prxoblem of finding the probability that a small population will survive, despite occasional disasters. It is shown that if substitutions occur at a rate Δ, and are scattered randomly over a genetic map of length R, then an allele is unlikely to be fixed if its advantage is less than a critical value, Scrit = (π2/6)(2ΔS/(Rlog(S/s))). This threshold depends primarily on the variance in fitness per unit map length dueto substitutions, var(W)/R = 2ΔS/R. With no recombination, the fixation probability can be calculated for a finite population. If Δ > s, it is of the same order as for a neutral allele ( ≈ Δ/(2N(Δ−s))), whilst if 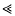, fixation probability is much higher than for a neutral allele, but much lower than in the absence of hitch-hiking 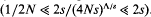. These results suggest that hitch-hiking may substantially impede the accumulation of weakly favoured adaptations.

Type
Research Article
Information
Genetics Research , Volume 64 , Issue 3 , December 1994 , pp. 199 - 208
Copyright
Copyright © Cambridge University Press 1994

References

Abramowitz, M. & Stegun, I. A. (1965). Handbook of Mathematical Functions. New York: Dover.Google Scholar
Aquadro, C. F. & Begun, D. J. (1993). Evidence for and implications of genetic hitch-hiking in the Drosophila genome. In Mechanics of Molecular Evolution (ed. Takahata, N. and Clark, A.G.), pp. 159–178. Sunderland, MA: Sinauer Press.Google Scholar
Barton, N. H. (1994). Linkage and the limits to natural selection. Genetics (in the press).Google Scholar
Birky, C. W. & Walsh, J. B. (1988). Effects of linkage on rates of molecular evolution. Proceedings of the National Academy of Science (USA) 85, 6414–6418.CrossRefGoogle ScholarPubMed
Charlesworth, B., Morgan, M. T. & Charlesworth, D. (1993). The effect of deleterious mutations on neutral molecular variation. Genetics 134, 1289–1303.CrossRefGoogle ScholarPubMed
Charlesworth, B. (1994). The effect of background selection against deleterious mutations on weakly selected, linked variants. Genetical Research 63, 213–228.CrossRefGoogle ScholarPubMed
Crow, J. F. (1970). Genetic loads and the cost of natural selection. In Mathematical Topics in Population Genetics (ed. Kojima, K. I.), pp. 128–177. Berlin: Springer-Verlag.CrossRefGoogle Scholar
Crow, J. F. & Kimura, M. (1965). Evolution in sexual and asexual populations. American Naturalist 99, 439–450.CrossRefGoogle Scholar
Crow, J. F. & Kimura, M. (1970). An Introduction to Population Genetics Theory. New York: Harper and Row.Google Scholar
Felsenstein, J. (1974). The evolutionary advantage of recombination. Genetics 78, 737–756.CrossRefGoogle ScholarPubMed
Felsenstein, J. (1988). Sex and the evolution of recombination. In The Evolution of Sex (ed. Michod, R. E. and Levin, B. R.), pp. 74–86. Sunderland, Massachussetts: Sinauer Press.Google Scholar
Fisher, R. A. (1930). The Genetical Theory of Natural Selection. Oxford: Oxford University Press.CrossRefGoogle Scholar
Gillespie, J. H. (1992). The Causes of Molecular Evolution. Oxford: Oxford University Press.CrossRefGoogle Scholar
Haldane, J. B. S. (1957). The cost of natural selection. Journal of Genetics 55, 511–524.CrossRefGoogle Scholar
Hill, W. G. (1966). The effect of linkage on limits to artificial selection. Genetical Research 8, 269–294.CrossRefGoogle ScholarPubMed
Hudson, R. R., Kreitman, M. & Aguade, M. (1987). A test of neutral molecular evolution based on nucleotide data. Genetics 114, 93–110.Google Scholar
Kaplan, N. L., Hudson, N. L. & Langley, C. H. (1989). The hitch-hiking effect revisited. Genetics 123, 887–899.CrossRefGoogle Scholar
Keightley, P. D. (1991). Genetic variance and fixation probabilities at quantitative trait loci in mutation selection balance. Genetical Research 58, 139–144.CrossRefGoogle Scholar
Mangel, M. & Tier, C. (1993). Dynamics of metapopulations with demographic stochasticity and environmental catastrophes. Theoretical Population Biology 44, 1–31.CrossRefGoogle Scholar
Smith, J. Maynard (1971). What use is sex? Journal of Theoretical Biology 30, 319–335.CrossRefGoogle ScholarPubMed
Smith, J. Maynard & Haigh, J. (1974). The hitch-hiking effect of a favourable gene. Genetical Research 23, 23–35.CrossRefGoogle ScholarPubMed
Muller, H. J. (1932). Some genetic aspects of sex. American Naturalist 66, 118–138.CrossRefGoogle Scholar
Ohta, T. & Kimura, M. (1970). Development of associative overdominance through linkage disequilibrium in finite populations. Genetical Research 16, 165–177.CrossRefGoogle ScholarPubMed
Stephan, W., Wiehe, T. H. & Lenz, M. (1992). The effect of strongly selected substitutions on neutral polymorphism: analytical results based on diffusion theory. Theoretical Population Biology 41, 237–254.CrossRefGoogle Scholar