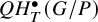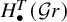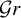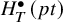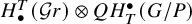1 Introduction
Let G be a simple simply-connected complex algebraic group. The quantum (resp. affine) Schubert calculus studies the algebra structure on the T-equivariant quantum cohomology
![]() $QH^{\bullet }_T(G/B)$
of the complete flag variety
$QH^{\bullet }_T(G/B)$
of the complete flag variety
![]() $G/B$
(resp. the T-equivariant Pontryagin homology
$G/B$
(resp. the T-equivariant Pontryagin homology
![]() $H^T_{\bullet }(\mathcal {G}r)$
of the affine Grassmannian
$H^T_{\bullet }(\mathcal {G}r)$
of the affine Grassmannian
![]() $\mathcal {G}r$
of G) in terms of the quantum Schubert classes
$\mathcal {G}r$
of G) in terms of the quantum Schubert classes
![]() $\{q^{\beta }\sigma _v\}_{(\beta ,v)\in \operatorname {\mathrm {Eff}}\times W}$
(resp. the affine Schubert classes
$\{q^{\beta }\sigma _v\}_{(\beta ,v)\in \operatorname {\mathrm {Eff}}\times W}$
(resp. the affine Schubert classes
![]() $\{\xi _{wt_{\lambda }}\}_{wt_{\lambda }\in W_{af}^-}$
). An unpublished result of Dale Peterson, announced during the lecturesFootnote
1
he gave at MIT in 1997, states that these two calculi are equivalent:
$\{\xi _{wt_{\lambda }}\}_{wt_{\lambda }\in W_{af}^-}$
). An unpublished result of Dale Peterson, announced during the lecturesFootnote
1
he gave at MIT in 1997, states that these two calculi are equivalent:
Theorem 1.1. The map
 $$\begin{align*}\begin{array}{ccccc} \Phi&:&H^T_{-\bullet}(\mathcal{G}r)&\rightarrow &QH^{\bullet}_T(G/B)[q_i^{-1}|~i\in I]\\ & & \xi_{wt_{\lambda}} & \mapsto & q^{\lambda}\sigma_{w} \end{array} \end{align*}$$
$$\begin{align*}\begin{array}{ccccc} \Phi&:&H^T_{-\bullet}(\mathcal{G}r)&\rightarrow &QH^{\bullet}_T(G/B)[q_i^{-1}|~i\in I]\\ & & \xi_{wt_{\lambda}} & \mapsto & q^{\lambda}\sigma_{w} \end{array} \end{align*}$$
is a graded homomorphism of
![]() $H_T^{\bullet }(\operatorname {\mathrm {pt}})$
-algebras.
$H_T^{\bullet }(\operatorname {\mathrm {pt}})$
-algebras.
A published proof, given by Lam and Shimozono [Reference Lam and Shimozono16], is algebraic and combinatorial. In this paper, we present a geometric proof by taking
![]() $\Phi $
to be the algebro-geometric and T-equivariant version of a map constructed by Savelyev [Reference Savelyev26] who generalized Seidel representations [Reference Seidel29] from 0-cycles in
$\Phi $
to be the algebro-geometric and T-equivariant version of a map constructed by Savelyev [Reference Savelyev26] who generalized Seidel representations [Reference Seidel29] from 0-cycles in
![]() $\mathcal {G}r$
to higher dimensional ones, and showing this map to have the desired form.
$\mathcal {G}r$
to higher dimensional ones, and showing this map to have the desired form.
In the same paper, Lam and Shimozono also proved the following:
Theorem 1.2. A parabolic version of Theorem 1.1 holds.
We will prove Theorem 1.2 as well. Since even stating it requires a substantial number of Lie-theoretic notations, we postpone the statement to Section 4.4, where we prove the Borel and parabolic cases simultaneously.
Remark 1.3. Savelyev has already computed his map partially. In [Reference Savelyev28], he showed that his map defined for
![]() $\mathbb {P}^n$
is nonzero on each generator of
$\mathbb {P}^n$
is nonzero on each generator of
![]() $\pi _*(\Omega SU(n+1))\otimes \mathbb {Q}$
which has degree
$\pi _*(\Omega SU(n+1))\otimes \mathbb {Q}$
which has degree
![]() $<2n$
. In [Reference Savelyev27], he proved that for any
$<2n$
. In [Reference Savelyev27], he proved that for any
![]() $wt_{\lambda }\in W_{af}^-$
such that w is the longest element of W, his map defined for
$wt_{\lambda }\in W_{af}^-$
such that w is the longest element of W, his map defined for
![]() $G/B$
sends
$G/B$
sends
![]() $\xi _{wt_{\lambda }}$
to
$\xi _{wt_{\lambda }}$
to
![]() $q^{\lambda }\sigma _{w}$
plus some higher terms with respect to an action functional on the space of sections of Hamiltonian fibrations.
$q^{\lambda }\sigma _{w}$
plus some higher terms with respect to an action functional on the space of sections of Hamiltonian fibrations.
Remark 1.4. The proof of Theorem 1.1 given by Lam and Shimozono relies on the equivariant quantum Chevalley formula [Reference Mihalcea21], which is the T-equivariant generalization of another unpublished result of Peterson proved by Fulton and Woodward [Reference Fulton and Woodward9]. Although we do not apply this formula directly, we do apply the key idea of the proof: the transverse property between the Schubert cells and the opposite Schubert cells in
![]() $G/P$
which implies that the moduli spaces for all two-pointed Gromov-Witten invariants are simultaneously regular and T-equivariant, allowing us to count the elements of their zero-dimensional components easily.
$G/P$
which implies that the moduli spaces for all two-pointed Gromov-Witten invariants are simultaneously regular and T-equivariant, allowing us to count the elements of their zero-dimensional components easily.
Remark 1.5. Unlike the proof by Lam and Shimozono, our proof of Theorem 1.2 is independent of Peterson-Woodward’s comparison formula [Reference Woodward30], which expresses explicitly the Schubert structure constants for
![]() $QH^{\bullet }(G/P)$
in terms of those for
$QH^{\bullet }(G/P)$
in terms of those for
![]() $QH^{\bullet }(G/B)$
. In fact, our work provides an alternative proof of this formula because it can be derived directly from Theorem 1.2 as shown by Huang and Li [Reference Huang and Li12, Proposition 2.10].
$QH^{\bullet }(G/B)$
. In fact, our work provides an alternative proof of this formula because it can be derived directly from Theorem 1.2 as shown by Huang and Li [Reference Huang and Li12, Proposition 2.10].
2 Preliminaries
2.1 Some notations
Let G be a simple simply-connected complex algebraic group and
![]() $T\subset G$
a maximal torus. Put
$T\subset G$
a maximal torus. Put
![]() $\mathfrak {g}:=\operatorname {\mathrm {Lie}}(G)$
and
$\mathfrak {g}:=\operatorname {\mathrm {Lie}}(G)$
and
![]() $\mathfrak {t}:=\operatorname {\mathrm {Lie}}(T)$
. Denote by R the set of roots associated to the pair
$\mathfrak {t}:=\operatorname {\mathrm {Lie}}(T)$
. Denote by R the set of roots associated to the pair
![]() $(\mathfrak {g},\mathfrak {t})$
. We have the root space decomposition
$(\mathfrak {g},\mathfrak {t})$
. We have the root space decomposition
where each
![]() $\mathfrak {g}_{\alpha }$
is a one-dimensional eigenspace with respect to the adjoint action of
$\mathfrak {g}_{\alpha }$
is a one-dimensional eigenspace with respect to the adjoint action of
![]() $\mathfrak {t}$
. Denote by W the Weyl group. Fix a fundamental system
$\mathfrak {t}$
. Denote by W the Weyl group. Fix a fundamental system
![]() $\{\alpha _i\}_{i\in I}$
of R, where
$\{\alpha _i\}_{i\in I}$
of R, where
![]() $I:=\{1,\ldots ,r\}$
. Denote by
$I:=\{1,\ldots ,r\}$
. Denote by
![]() $R^+\subset R$
the set of positive roots spanned by the
$R^+\subset R$
the set of positive roots spanned by the
![]() $\alpha _i$
’s. We have two particular Borel subgroups
$\alpha _i$
’s. We have two particular Borel subgroups
![]() $B^-$
and
$B^-$
and
![]() $B^+$
of G containing T with their Lie algebras equal to
$B^+$
of G containing T with their Lie algebras equal to
![]() $\mathfrak {t}\oplus \bigoplus _{\alpha \in -R^+}\mathfrak {g}_{\alpha }$
and
$\mathfrak {t}\oplus \bigoplus _{\alpha \in -R^+}\mathfrak {g}_{\alpha }$
and
![]() $\mathfrak {t}\oplus \bigoplus _{\alpha \in R^+}\mathfrak {g}_{\alpha }$
, respectively.
$\mathfrak {t}\oplus \bigoplus _{\alpha \in R^+}\mathfrak {g}_{\alpha }$
, respectively.
Let
![]() $W_{af}:=W\ltimes Q^{\vee }$
be the affine Weyl group where
$W_{af}:=W\ltimes Q^{\vee }$
be the affine Weyl group where
![]() $Q^{\vee }:=\sum _{\alpha \in R}\mathbb {Z}\cdot \alpha ^{\vee }\subset \mathfrak {t}$
is the lattice spanned by the coroots. Elements of
$Q^{\vee }:=\sum _{\alpha \in R}\mathbb {Z}\cdot \alpha ^{\vee }\subset \mathfrak {t}$
is the lattice spanned by the coroots. Elements of
![]() $W_{af}$
are denoted by
$W_{af}$
are denoted by
![]() $wt_{\lambda }$
with
$wt_{\lambda }$
with
![]() $w\in W$
and
$w\in W$
and
![]() $\lambda \in Q^{\vee }$
(where
$\lambda \in Q^{\vee }$
(where
![]() $t_{\lambda }$
means the translation
$t_{\lambda }$
means the translation
![]() $x\mapsto x+\lambda $
). Denote by
$x\mapsto x+\lambda $
). Denote by
![]() $W_{af}^-$
the set of minimal length coset representatives in
$W_{af}^-$
the set of minimal length coset representatives in
![]() $W_{af}/W$
. It is easy to see that the map
$W_{af}/W$
. It is easy to see that the map
![]() $W_{af}^-\rightarrow Q^{\vee }$
defined by
$W_{af}^-\rightarrow Q^{\vee }$
defined by
![]() $wt_{\lambda }\mapsto w(\lambda )$
is bijective.
$wt_{\lambda }\mapsto w(\lambda )$
is bijective.
2.2 Flag varieties
Let P be a parabolic subgroup of G containing
![]() $B^+$
. Define
$B^+$
. Define
![]() $R^+_P\subseteq R^+$
to be the subset such that
$R^+_P\subseteq R^+$
to be the subset such that
 $$\begin{align*}\operatorname{\mathrm{Lie}}(P)=\operatorname{\mathrm{Lie}}(B^+)\oplus\bigoplus_{\alpha\in -R_P^+}\mathfrak{g}_{\alpha}\end{align*}$$
$$\begin{align*}\operatorname{\mathrm{Lie}}(P)=\operatorname{\mathrm{Lie}}(B^+)\oplus\bigoplus_{\alpha\in -R_P^+}\mathfrak{g}_{\alpha}\end{align*}$$
and
![]() $R_P:=R^+_P\cup (-R^+_P)$
. Let
$R_P:=R^+_P\cup (-R^+_P)$
. Let
![]() $I_P\subseteq I$
be the set of
$I_P\subseteq I$
be the set of
![]() $i\in I$
such that
$i\in I$
such that
![]() $\alpha _i\in R_P^+$
. Define
$\alpha _i\in R_P^+$
. Define
![]() $Q^{\vee }_P:=\sum _{\alpha \in R^+_P}\mathbb {Z}\cdot \alpha ^{\vee }\subseteq Q^{\vee }$
. Denote by
$Q^{\vee }_P:=\sum _{\alpha \in R^+_P}\mathbb {Z}\cdot \alpha ^{\vee }\subseteq Q^{\vee }$
. Denote by
![]() $W_P\subseteq W$
the subgroup generated by the simple reflections
$W_P\subseteq W$
the subgroup generated by the simple reflections
![]() $s_{\alpha _i}$
with
$s_{\alpha _i}$
with
![]() $i\in I_P$
and by
$i\in I_P$
and by
![]() $W^P$
the set of minimal length coset representatives in
$W^P$
the set of minimal length coset representatives in
![]() $W/W_P$
. For any
$W/W_P$
. For any
![]() $v\in W^P$
, define
$v\in W^P$
, define
![]() $y_v:=\dot {v}P\in G/P$
, where
$y_v:=\dot {v}P\in G/P$
, where
![]() $\dot {v}\in N(T)$
is any representative of v. Then
$\dot {v}\in N(T)$
is any representative of v. Then
![]() $\{y_v\}_{v\in W^P}$
is the set of T-fixed points of
$\{y_v\}_{v\in W^P}$
is the set of T-fixed points of
![]() $G/P$
.
$G/P$
.
The
![]() $B^-$
-orbits
$B^-$
-orbits
![]() $B^{-}\cdot y_v\subseteq G/P,~v\in W^P$
are called the Schubert cells, and the
$B^{-}\cdot y_v\subseteq G/P,~v\in W^P$
are called the Schubert cells, and the
![]() $B^+$
-orbits
$B^+$
-orbits
![]() $B^+\cdot y_v\subseteq G/P,~v\in W^P$
are called the opposite Schubert cells. Define the (opposite) Schubert classes
$B^+\cdot y_v\subseteq G/P,~v\in W^P$
are called the opposite Schubert cells. Define the (opposite) Schubert classes
 $$ \begin{align*} \sigma_v:=&~ \operatorname{\mathrm{PD}} \left[~\overline{B^{-}\cdot y_v}~\right]\in H_T^{2\ell(v)}(G/P)\\ \sigma^v:=&~ \operatorname{\mathrm{PD}} \left[~\overline{B^{+}\cdot y_v}~\right]\in H_T^{\dim_{\mathbb{R}}(G/P)-2\ell(v)}(G/P). \end{align*} $$
$$ \begin{align*} \sigma_v:=&~ \operatorname{\mathrm{PD}} \left[~\overline{B^{-}\cdot y_v}~\right]\in H_T^{2\ell(v)}(G/P)\\ \sigma^v:=&~ \operatorname{\mathrm{PD}} \left[~\overline{B^{+}\cdot y_v}~\right]\in H_T^{\dim_{\mathbb{R}}(G/P)-2\ell(v)}(G/P). \end{align*} $$
Then
![]() $\{\sigma _v\}_{v\in W^P}$
and
$\{\sigma _v\}_{v\in W^P}$
and
![]() $\{\sigma ^v\}_{v\in W^P}$
are
$\{\sigma ^v\}_{v\in W^P}$
are
![]() $H_T^{\bullet }(\operatorname {\mathrm {pt}})$
-bases of
$H_T^{\bullet }(\operatorname {\mathrm {pt}})$
-bases of
![]() $H_T^{\bullet }(G/P)$
.
$H_T^{\bullet }(G/P)$
.
The following well-known fact is crucial to us.
Lemma 2.1. Every Schubert cell intersects every opposite Schubert cell transversely. In particular,
![]() $\{\sigma _v\}_{v\in W^P}$
and
$\{\sigma _v\}_{v\in W^P}$
and
![]() $\{\sigma ^v\}_{v\in W^P}$
are dual to each other with respect to
$\{\sigma ^v\}_{v\in W^P}$
are dual to each other with respect to
![]() $\int _{G/P}-\cup -$
.
$\int _{G/P}-\cup -$
.
Proof. See, for example, [Reference Fulton and Woodward9, Section 7].
It is also well known that the closures of (resp. opposite) Schubert cells have
![]() $B^-$
-equivariant (resp.
$B^-$
-equivariant (resp.
![]() $B^+$
-equivariant) resolutions (e.g., the Bott-Samelson-Demazure-Hansen resolutions). See, for example, [Reference Brion4, Section 2] for the construction.
$B^+$
-equivariant) resolutions (e.g., the Bott-Samelson-Demazure-Hansen resolutions). See, for example, [Reference Brion4, Section 2] for the construction.
Definition 2.2. For each
![]() $v\in W^P$
, fix a
$v\in W^P$
, fix a
![]() $B^+$
-equivariant morphism
$B^+$
-equivariant morphism
which is the composition of a resolution
![]() $\Gamma _v\rightarrow \overline {B^+\cdot y_v}$
and the inclusion
$\Gamma _v\rightarrow \overline {B^+\cdot y_v}$
and the inclusion
![]() $\overline {B^+\cdot y_v}\hookrightarrow G/P$
.
$\overline {B^+\cdot y_v}\hookrightarrow G/P$
.
We now recall the T-equivariant quantum cohomology of
![]() $G/P$
. See, for example, [Reference Cox and Katz5, Reference Fulton and Pandharipande8, Reference Kontsevich and Manin15] for more details. There are isomorphisms
$G/P$
. See, for example, [Reference Cox and Katz5, Reference Fulton and Pandharipande8, Reference Kontsevich and Manin15] for more details. There are isomorphisms
where the first is defined as the dual of the composition of three isomorphisms:
Here,
![]() $L_{\rho }$
is the line bundle
$L_{\rho }$
is the line bundle
![]() $G\times _P \mathbb {C}_{-\rho }$
, where
$G\times _P \mathbb {C}_{-\rho }$
, where
![]() $\mathbb {C}_{-\rho }$
is the one-dimensional representation of weight
$\mathbb {C}_{-\rho }$
is the one-dimensional representation of weight
![]() $-\rho $
on which P acts by forgetting the semi-simple and unipotent parts. Denote by
$-\rho $
on which P acts by forgetting the semi-simple and unipotent parts. Denote by
![]() $\operatorname {\mathrm {Eff}}\subset H_2(G/P)$
the semi-group of effective curve classes in
$\operatorname {\mathrm {Eff}}\subset H_2(G/P)$
the semi-group of effective curve classes in
![]() $G/P$
. Under (2.1),
$G/P$
. Under (2.1),
![]() $\operatorname {\mathrm {Eff}}$
corresponds to the semi-subgroup of
$\operatorname {\mathrm {Eff}}$
corresponds to the semi-subgroup of
![]() $Q^{\vee }/Q^{\vee }_P$
generated by
$Q^{\vee }/Q^{\vee }_P$
generated by
![]() $\alpha ^{\vee }_i$
with
$\alpha ^{\vee }_i$
with
![]() $i\in I\setminus I_P$
.
$i\in I\setminus I_P$
.
Define the T-equivariant quantum cohomology of
![]() $G/P$
$G/P$
We grade
![]() $QH_T^{\bullet }(G/P)$
by declaring each
$QH_T^{\bullet }(G/P)$
by declaring each
![]() $q_i$
to have degree
$q_i$
to have degree
![]() $2\sum _{\alpha \in R^+\setminus R^+_P}\alpha (\alpha ^{\vee }_i)$
. The T-equivariant quantum cup product
$2\sum _{\alpha \in R^+\setminus R^+_P}\alpha (\alpha ^{\vee }_i)$
. The T-equivariant quantum cup product
![]() $\star $
is a deformation of the T-equivariant cup product, defined by
$\star $
is a deformation of the T-equivariant cup product, defined by
 $$\begin{align*}\sigma_u\star \sigma_v := \sum_{w\in W^P}\sum_{\mathbf{d}} \left(\prod_{i\in I\setminus I_P} q_i^{d_i}\right) \left(\int_{\overline{\mathcal{M}}_{0,3}(G/P,\beta_{\mathbf{d}})} \operatorname{\mathrm{ev}}_1^*\sigma_u\cup \operatorname{\mathrm{ev}}_2^*\sigma_v\cup \operatorname{\mathrm{ev}}_3^*\sigma^w \right) \sigma_w,\end{align*}$$
$$\begin{align*}\sigma_u\star \sigma_v := \sum_{w\in W^P}\sum_{\mathbf{d}} \left(\prod_{i\in I\setminus I_P} q_i^{d_i}\right) \left(\int_{\overline{\mathcal{M}}_{0,3}(G/P,\beta_{\mathbf{d}})} \operatorname{\mathrm{ev}}_1^*\sigma_u\cup \operatorname{\mathrm{ev}}_2^*\sigma_v\cup \operatorname{\mathrm{ev}}_3^*\sigma^w \right) \sigma_w,\end{align*}$$
where
-
1.
 $\mathbf {d}=\{d_i\}_{i\in I\setminus I_P}$
runs over the set of
$\mathbf {d}=\{d_i\}_{i\in I\setminus I_P}$
runs over the set of
 $(I\setminus I_P)$
-tuples of non-negative integers;
$(I\setminus I_P)$
-tuples of non-negative integers; -
2.
 $\beta _{\mathbf {d}}\in \operatorname {\mathrm {Eff}}$
corresponds to
$\beta _{\mathbf {d}}\in \operatorname {\mathrm {Eff}}$
corresponds to
 $\sum _{i\in I\setminus I_P}d_i\alpha ^{\vee }_i$
via the isomorphism (2.1);
$\sum _{i\in I\setminus I_P}d_i\alpha ^{\vee }_i$
via the isomorphism (2.1); -
3.
 $\overline {\mathcal {M}}_{0,3}(G/P,\beta _{\mathbf {d}})$
is the moduli of genus-zero stable maps to
$\overline {\mathcal {M}}_{0,3}(G/P,\beta _{\mathbf {d}})$
is the moduli of genus-zero stable maps to
 $G/P$
of degree
$G/P$
of degree
 $\beta _{\mathbf {d}}$
with three marked points and are the evaluation morphisms at these marked points; and
$\beta _{\mathbf {d}}$
with three marked points and are the evaluation morphisms at these marked points; and $$\begin{align*}\operatorname{\mathrm{ev}}_1,\operatorname{\mathrm{ev}}_2,\operatorname{\mathrm{ev}}_3:\overline{\mathcal{M}}_{0,3}(G/P,\beta_{\mathbf{d}})\rightarrow G/P\end{align*}$$
$$\begin{align*}\operatorname{\mathrm{ev}}_1,\operatorname{\mathrm{ev}}_2,\operatorname{\mathrm{ev}}_3:\overline{\mathcal{M}}_{0,3}(G/P,\beta_{\mathbf{d}})\rightarrow G/P\end{align*}$$
-
4. the integral
 $\int _{\overline {\mathcal {M}}_{0,3}(G/P,\beta _{\mathbf {d}})}$
is the T-equivariant integral.
$\int _{\overline {\mathcal {M}}_{0,3}(G/P,\beta _{\mathbf {d}})}$
is the T-equivariant integral.
Then
![]() $(QH_T^{\bullet }(G/P),\star )$
is a graded commutative
$(QH_T^{\bullet }(G/P),\star )$
is a graded commutative
![]() $H_T^{\bullet }(\operatorname {\mathrm {pt}})$
-algebra.
$H_T^{\bullet }(\operatorname {\mathrm {pt}})$
-algebra.
2.3 Affine Grassmannian
The affine Grassmannian
![]() $\mathcal {G}r$
of G is by definition (see, for example, [Reference Zhu31, Section 1.2]) the functor
$\mathcal {G}r$
of G is by definition (see, for example, [Reference Zhu31, Section 1.2]) the functor
 $$\begin{align*}\begin{array}{cccc} & AffSch_{\mathbb{C}} &\rightarrow& Sets\\ &\operatorname{\mathrm{Spec}} R&\mapsto & \left\{\begin{array}{l} \text{isomorphism classes of }(\mathcal{E}^o,\nu^o)\text{ where}\\[.2em] \mathcal{E}^o\text{ is a }G\text{-torsor over }\operatorname{\mathrm{Spec}} R[[z]], \\[.2em] \nu^o:\mathcal{E}^o|_{\operatorname{\mathrm{Spec}} R((z))}\xrightarrow{\sim}\operatorname{\mathrm{Spec}} R((z))\times G\\[.2em] \text{is a trivialization} \end{array} \right\} \end{array}. \end{align*}$$
$$\begin{align*}\begin{array}{cccc} & AffSch_{\mathbb{C}} &\rightarrow& Sets\\ &\operatorname{\mathrm{Spec}} R&\mapsto & \left\{\begin{array}{l} \text{isomorphism classes of }(\mathcal{E}^o,\nu^o)\text{ where}\\[.2em] \mathcal{E}^o\text{ is a }G\text{-torsor over }\operatorname{\mathrm{Spec}} R[[z]], \\[.2em] \nu^o:\mathcal{E}^o|_{\operatorname{\mathrm{Spec}} R((z))}\xrightarrow{\sim}\operatorname{\mathrm{Spec}} R((z))\times G\\[.2em] \text{is a trivialization} \end{array} \right\} \end{array}. \end{align*}$$
(We use the notation
![]() $(\mathcal {E}^o,\nu ^o)$
instead of a more natural one
$(\mathcal {E}^o,\nu ^o)$
instead of a more natural one
![]() $(\mathcal {E},\nu )$
because the latter is reserved for G-torsors over
$(\mathcal {E},\nu )$
because the latter is reserved for G-torsors over
![]() $\mathbb {P}^1$
.) It is well known that
$\mathbb {P}^1$
.) It is well known that
![]() $\mathcal {G}r$
is represented by an Ind-projective Ind-scheme. See, for example, [Reference Zhu31, Theorem 1.2.2]. By Beauville-Laszlo’s theorem [Reference Beauville and Laszlo2],
$\mathcal {G}r$
is represented by an Ind-projective Ind-scheme. See, for example, [Reference Zhu31, Theorem 1.2.2]. By Beauville-Laszlo’s theorem [Reference Beauville and Laszlo2],
![]() $\mathcal {G}r$
also represents the subfunctor
$\mathcal {G}r$
also represents the subfunctor
 $$\begin{align*}\operatorname{\mathrm{Spec}} R\mapsto \left\{\begin{array}{l} \text{isomorphism classes of }(\mathcal{E}^o,\nu^o)\text{ where}\\[.2em] \mathcal{E}^o\text{ is a }G\text{-torsor over }\operatorname{\mathrm{Spec}} R[z], \\[.2em] \nu^o:\mathcal{E}^o|_{\operatorname{\mathrm{Spec}} R[z,z^{-1}]}\xrightarrow{\sim}\operatorname{\mathrm{Spec}} R[z,z^{-1}]\times G\\[.2em] \text{is a trivialization} \end{array} \right\}. \end{align*}$$
$$\begin{align*}\operatorname{\mathrm{Spec}} R\mapsto \left\{\begin{array}{l} \text{isomorphism classes of }(\mathcal{E}^o,\nu^o)\text{ where}\\[.2em] \mathcal{E}^o\text{ is a }G\text{-torsor over }\operatorname{\mathrm{Spec}} R[z], \\[.2em] \nu^o:\mathcal{E}^o|_{\operatorname{\mathrm{Spec}} R[z,z^{-1}]}\xrightarrow{\sim}\operatorname{\mathrm{Spec}} R[z,z^{-1}]\times G\\[.2em] \text{is a trivialization} \end{array} \right\}. \end{align*}$$
Let
![]() $\mu \in Q^{\vee }$
. Denote by
$\mu \in Q^{\vee }$
. Denote by
![]() $t^{\mu }$
the
$t^{\mu }$
the
![]() $\operatorname {\mathrm {Spec}}\mathbb {C}$
-point of
$\operatorname {\mathrm {Spec}}\mathbb {C}$
-point of
![]() $\mathcal {G}r$
represented by the trivial G-torsor with trivialization
$\mathcal {G}r$
represented by the trivial G-torsor with trivialization
![]() $(z,g)\mapsto (z,\mu (z)g)$
. One checks easily that it is a T-fixed point of
$(z,g)\mapsto (z,\mu (z)g)$
. One checks easily that it is a T-fixed point of
![]() $\mathcal {G}r$
. It is known that if G is simply-connected, every T-fixed point of
$\mathcal {G}r$
. It is known that if G is simply-connected, every T-fixed point of
![]() $\mathcal {G}r$
is of this form. Define
$\mathcal {G}r$
is of this form. Define
![]() $\mathcal {B}:=\operatorname {\mathrm {ev}}_{z=0}^{-1}(B^-)$
, where
$\mathcal {B}:=\operatorname {\mathrm {ev}}_{z=0}^{-1}(B^-)$
, where
![]() $\operatorname {\mathrm {ev}}_{z=0}:G(\mathbb {C}[[z]])\rightarrow G$
is the evaluation map at
$\operatorname {\mathrm {ev}}_{z=0}:G(\mathbb {C}[[z]])\rightarrow G$
is the evaluation map at
![]() $z=0$
. For any
$z=0$
. For any
![]() $\mu \in Q^{\vee }$
, the orbit
$\mu \in Q^{\vee }$
, the orbit
![]() $\mathcal {B}\cdot t^{\mu }$
is isomorphic to an affine space, and we call it an affine Schubert cell. In this paper, it is more convenient to index affine Schubert cells by
$\mathcal {B}\cdot t^{\mu }$
is isomorphic to an affine space, and we call it an affine Schubert cell. In this paper, it is more convenient to index affine Schubert cells by
![]() $W_{af}^-$
instead of
$W_{af}^-$
instead of
![]() $Q^{\vee }$
. (See Section 2.1 for the definition of
$Q^{\vee }$
. (See Section 2.1 for the definition of
![]() $W_{af}^-$
.) For any
$W_{af}^-$
.) For any
![]() $wt_{\lambda }\in W_{af}^-$
, we define the affine Schubert class
$wt_{\lambda }\in W_{af}^-$
, we define the affine Schubert class
Then
![]() $\{\xi _{wt_{\lambda }}\}_{wt_{\lambda }\in W_{af}^-}$
is an
$\{\xi _{wt_{\lambda }}\}_{wt_{\lambda }\in W_{af}^-}$
is an
![]() $H_T^{\bullet }(\operatorname {\mathrm {pt}})$
-basis of the T-equivariant homology
$H_T^{\bullet }(\operatorname {\mathrm {pt}})$
-basis of the T-equivariant homology
![]() $H^T_{\bullet }(\mathcal {G}r)$
of
$H^T_{\bullet }(\mathcal {G}r)$
of
![]() $\mathcal {G}r$
.
$\mathcal {G}r$
.
Denote by
![]() $\mathcal {L} G$
the loop group functor
$\mathcal {L} G$
the loop group functor
![]() $\operatorname {\mathrm {Spec}} R\mapsto G(R((z)))$
. We have a natural group action
$\operatorname {\mathrm {Spec}} R\mapsto G(R((z)))$
. We have a natural group action
defined by
for any
![]() $\varphi \in G(R((z)))$
and
$\varphi \in G(R((z)))$
and
![]() $\operatorname {\mathrm {Spec}} R$
-point
$\operatorname {\mathrm {Spec}} R$
-point
![]() $[(\mathcal {E}^o,\nu ^o)]$
of
$[(\mathcal {E}^o,\nu ^o)]$
of
![]() $\mathcal {G}r$
, where
$\mathcal {G}r$
, where
![]() $\varphi \cdot \nu ^o(p):=(x,\varphi (z)g)\in \operatorname {\mathrm {Spec}} R((z))\times G$
for
$\varphi \cdot \nu ^o(p):=(x,\varphi (z)g)\in \operatorname {\mathrm {Spec}} R((z))\times G$
for
![]() $\nu ^o(p)=(x,g)$
.
$\nu ^o(p)=(x,g)$
.
For any
![]() $\alpha \in R$
and
$\alpha \in R$
and
![]() $k\in \mathbb {Z}$
, denote by
$k\in \mathbb {Z}$
, denote by
![]() $U_{\alpha ,k}\subset \mathcal {L} G$
the affine root group
$U_{\alpha ,k}\subset \mathcal {L} G$
the affine root group
![]() $\exp (z^k\mathfrak {g}_{\alpha })\simeq \mathbb {G}_a$
.
$\exp (z^k\mathfrak {g}_{\alpha })\simeq \mathbb {G}_a$
.
Definition 2.3. Let H be a subgroup of G. A morphism
![]() $f:\Gamma \rightarrow \mathcal {G}r$
from a variety
$f:\Gamma \rightarrow \mathcal {G}r$
from a variety
![]() $\Gamma $
to
$\Gamma $
to
![]() $\mathcal {G}r$
is said to be H-good if
$\mathcal {G}r$
is said to be H-good if
![]() $\Gamma $
has an algebraic H-action and an algebraic
$\Gamma $
has an algebraic H-action and an algebraic
![]() $U_{\alpha ,k}$
-action for each
$U_{\alpha ,k}$
-action for each
![]() $\alpha \in R$
and
$\alpha \in R$
and
![]() $k>0$
such that f is equivariant with respect to these group actions.
$k>0$
such that f is equivariant with respect to these group actions.
Lemma 2.4. For any
![]() $wt_{\lambda }\in W_{af}^-$
, there exists a
$wt_{\lambda }\in W_{af}^-$
, there exists a
![]() $B^-$
-good morphism
$B^-$
-good morphism
![]() $f:\Gamma \rightarrow \mathcal {G}r$
which is the composition of a resolution
$f:\Gamma \rightarrow \mathcal {G}r$
which is the composition of a resolution
![]() $\Gamma \rightarrow \overline {\mathcal {B}\cdot t^{w(\lambda )}}$
and the inclusion
$\Gamma \rightarrow \overline {\mathcal {B}\cdot t^{w(\lambda )}}$
and the inclusion
![]() $\overline {\mathcal {B}\cdot t^{w(\lambda )}}\hookrightarrow \mathcal {G}r$
.
$\overline {\mathcal {B}\cdot t^{w(\lambda )}}\hookrightarrow \mathcal {G}r$
.
Proof. Notice that for
![]() $\mathcal {H}=B^-$
or
$\mathcal {H}=B^-$
or
![]() $U_{\alpha ,k}$
with
$U_{\alpha ,k}$
with
![]() $\alpha \in R$
and
$\alpha \in R$
and
![]() $k>0$
, the
$k>0$
, the
![]() $\mathcal {H}$
-action on
$\mathcal {H}$
-action on
![]() $\mathcal {G}r$
induces an
$\mathcal {G}r$
induces an
![]() $\mathcal {H}$
-action on
$\mathcal {H}$
-action on
![]() $\overline {\mathcal {B}\cdot t^{w(\lambda )}}$
such that the inclusion
$\overline {\mathcal {B}\cdot t^{w(\lambda )}}$
such that the inclusion
![]() $\iota :\overline {\mathcal {B}\cdot t^{w(\lambda )}}\hookrightarrow \mathcal {G}r$
is
$\iota :\overline {\mathcal {B}\cdot t^{w(\lambda )}}\hookrightarrow \mathcal {G}r$
is
![]() $\mathcal {H}$
-equivariant.
$\mathcal {H}$
-equivariant.
By [Reference Kollár14, Proposition 3.9.1 & Theorem 3.26], there exists a resolution
![]() $r:\Gamma \rightarrow \overline {\mathcal {B}\cdot t^{w(\lambda )}}$
such that every algebraic group action on
$r:\Gamma \rightarrow \overline {\mathcal {B}\cdot t^{w(\lambda )}}$
such that every algebraic group action on
![]() $\overline {\mathcal {B}\cdot t^{w(\lambda )}}$
lifts to an algebraic group action on
$\overline {\mathcal {B}\cdot t^{w(\lambda )}}$
lifts to an algebraic group action on
![]() $\Gamma $
. It follows that the composition
$\Gamma $
. It follows that the composition
![]() $f:=\iota \circ r$
is a
$f:=\iota \circ r$
is a
![]() $B^-$
-good morphism.
$B^-$
-good morphism.
Alternatively, one can take a Bott-Samelson-Demazure-Hansen resolution of
![]() $\overline {\mathcal {B}\cdot t^{w(\lambda )}}$
. See, for example, [Reference Pappas and Rapoport24, Section 8] for the construction.
$\overline {\mathcal {B}\cdot t^{w(\lambda )}}$
. See, for example, [Reference Pappas and Rapoport24, Section 8] for the construction.
Definition 2.5. For each
![]() $wt_{\lambda }\in W_{af}^-$
, fix a
$wt_{\lambda }\in W_{af}^-$
, fix a
![]() $B^-$
-good morphism
$B^-$
-good morphism
which is the composition of a resolution
![]() $\Gamma _{wt_{\lambda }}\rightarrow \overline {\mathcal {B}\cdot t^{w(\lambda )}}$
and the inclusion
$\Gamma _{wt_{\lambda }}\rightarrow \overline {\mathcal {B}\cdot t^{w(\lambda )}}$
and the inclusion
![]() $\overline {\mathcal {B}\cdot t^{w(\lambda )}}\hookrightarrow \mathcal {G}r$
.
$\overline {\mathcal {B}\cdot t^{w(\lambda )}}\hookrightarrow \mathcal {G}r$
.
Let K be a maximal compact subgroup of G such that
![]() $T_K:=T\cap K$
is a maximal torus of K. Let
$T_K:=T\cap K$
is a maximal torus of K. Let
![]() $\Omega _{pol} K$
be the space of polynomial based loops in K. It is well known that the canonical map
$\Omega _{pol} K$
be the space of polynomial based loops in K. It is well known that the canonical map
![]() $\Omega _{pol} K\rightarrow \mathcal {G}r$
is a
$\Omega _{pol} K\rightarrow \mathcal {G}r$
is a
![]() $T_K$
-equivariant homeomorphism. See [Reference Zhu31, Theorem 1.6.1] for an exposition of the proof of this result and the references cited therein – namely, [Reference Nadler22, Section 4] and [Reference Pressley and Segal25, Section 8.3]. Notice that
$T_K$
-equivariant homeomorphism. See [Reference Zhu31, Theorem 1.6.1] for an exposition of the proof of this result and the references cited therein – namely, [Reference Nadler22, Section 4] and [Reference Pressley and Segal25, Section 8.3]. Notice that
![]() $\Omega _{pol} K$
is a group. Its group structure thus induces an
$\Omega _{pol} K$
is a group. Its group structure thus induces an
![]() $H_T^{\bullet }(\operatorname {\mathrm {pt}})$
-algebra structure on
$H_T^{\bullet }(\operatorname {\mathrm {pt}})$
-algebra structure on
![]() $H^T_{\bullet }(\mathcal {G}r)$
. It is called the Pontryagin product. By definition, we have
$H^T_{\bullet }(\mathcal {G}r)$
. It is called the Pontryagin product. By definition, we have
Since
![]() $\{[t^{\mu }]\}_{\mu \in Q^{\vee }}$
is a basis of
$\{[t^{\mu }]\}_{\mu \in Q^{\vee }}$
is a basis of
![]() $H^T_{\bullet }(\mathcal {G}r)\otimes _{H_T^{\bullet }(\operatorname {\mathrm {pt}})}\operatorname {\mathrm {Frac}}(H_T^{\bullet }(\operatorname {\mathrm {pt}}))$
, these equalities determine the Pontryagin product completely.
$H^T_{\bullet }(\mathcal {G}r)\otimes _{H_T^{\bullet }(\operatorname {\mathrm {pt}})}\operatorname {\mathrm {Frac}}(H_T^{\bullet }(\operatorname {\mathrm {pt}}))$
, these equalities determine the Pontryagin product completely.
3 The Savelyev-Seidel homomorphism
3.1
 $G/P$
-bundles
$G/P$
-bundles
Let
![]() $f:\Gamma \rightarrow \mathcal {G}r$
be a morphism where
$f:\Gamma \rightarrow \mathcal {G}r$
be a morphism where
![]() $\Gamma $
is a variety. It is represented by a pair
$\Gamma $
is a variety. It is represented by a pair
![]() $(\mathcal {E}_f^o,\nu _f^o)$
where
$(\mathcal {E}_f^o,\nu _f^o)$
where
![]() $\mathcal {E}_f^o$
is a G-torsor over
$\mathcal {E}_f^o$
is a G-torsor over
![]() $\mathbb {A}^1_z\times \Gamma $
and
$\mathbb {A}^1_z\times \Gamma $
and
![]() $\nu _f^o:\mathcal {E}_f^o|_{(\mathbb {A}^1_z\setminus 0)\times \Gamma }\xrightarrow {\sim } (\mathbb {A}^1_z\setminus 0)\times \Gamma \times G$
is a trivialization. To see this, take a covering
$\nu _f^o:\mathcal {E}_f^o|_{(\mathbb {A}^1_z\setminus 0)\times \Gamma }\xrightarrow {\sim } (\mathbb {A}^1_z\setminus 0)\times \Gamma \times G$
is a trivialization. To see this, take a covering
![]() $\{U_i\}_i$
of
$\{U_i\}_i$
of
![]() $\Gamma $
by affine open subsets. By the definition of
$\Gamma $
by affine open subsets. By the definition of
![]() $\mathcal {G}r$
, each
$\mathcal {G}r$
, each
![]() $f|_{U_i}$
is represented by a pair
$f|_{U_i}$
is represented by a pair
![]() $(\mathcal {E}^o_{f|_{U_i}},\nu ^o_{f|_{U_i}})$
. Since in general, every pair
$(\mathcal {E}^o_{f|_{U_i}},\nu ^o_{f|_{U_i}})$
. Since in general, every pair
![]() $(\mathcal {E}^o,\nu ^o)$
has no nontrivial automorphism (essentially due to the trivialization
$(\mathcal {E}^o,\nu ^o)$
has no nontrivial automorphism (essentially due to the trivialization
![]() $\nu ^o$
), it follows that we can glue
$\nu ^o$
), it follows that we can glue
![]() $(\mathcal {E}^o_{f|_{U_i}},\nu ^o_{f|_{U_i}})$
to form the desired pair
$(\mathcal {E}^o_{f|_{U_i}},\nu ^o_{f|_{U_i}})$
to form the desired pair
![]() $(\mathcal {E}_f^o,\nu _f^o)$
.
$(\mathcal {E}_f^o,\nu _f^o)$
.
Identify
![]() $\mathbb {A}^1_z$
with
$\mathbb {A}^1_z$
with
![]() $\mathbb {P}^1\setminus \infty $
. Glue
$\mathbb {P}^1\setminus \infty $
. Glue
![]() $\mathcal {E}_f^o$
and
$\mathcal {E}_f^o$
and
![]() $(\mathbb {P}^1\setminus 0)\times \Gamma \times G$
using
$(\mathbb {P}^1\setminus 0)\times \Gamma \times G$
using
![]() $\nu _f^o$
. The resulting variety is a G-torsor over
$\nu _f^o$
. The resulting variety is a G-torsor over
![]() $\mathbb {P}^1\times \Gamma $
with a trivialization over
$\mathbb {P}^1\times \Gamma $
with a trivialization over
![]() $(\mathbb {P}^1\setminus 0)\times \Gamma $
. We denote the G-torsor by
$(\mathbb {P}^1\setminus 0)\times \Gamma $
. We denote the G-torsor by
![]() $\mathcal {E}_f$
and the trivialization by
$\mathcal {E}_f$
and the trivialization by
![]() $\nu _f$
.
$\nu _f$
.
Remark 3.1. There is a parallel story in the analytic category. In [Reference Pressley and Segal25], Pressley and Segal defined
![]() $\mathcal {G}r$
to be the based loop group
$\mathcal {G}r$
to be the based loop group
![]() $\Omega K$
with respect to various topologies and showed [Reference Pressley and Segal25, Theorem 8.10.2] that there is a bijective correspondence between the set of holomorphic maps
$\Omega K$
with respect to various topologies and showed [Reference Pressley and Segal25, Theorem 8.10.2] that there is a bijective correspondence between the set of holomorphic maps
![]() $f:\Gamma \rightarrow \mathcal {G}r$
and the set of isomorphism classes of holomorphic principal G-bundles over
$f:\Gamma \rightarrow \mathcal {G}r$
and the set of isomorphism classes of holomorphic principal G-bundles over
![]() $\mathbb {P}^1\times \Gamma $
with trivializations over
$\mathbb {P}^1\times \Gamma $
with trivializations over
![]() $(\mathbb {P}^1\setminus \{|z|\leqslant 1\})\times \Gamma $
.
$(\mathbb {P}^1\setminus \{|z|\leqslant 1\})\times \Gamma $
.
Lemma 3.2. The associated fiber bundle
exists as a variety. The canonical projection
is smooth and projective. In particular,
![]() $\mathcal {E}_{f}(G/P)$
is smooth (resp. projective) if
$\mathcal {E}_{f}(G/P)$
is smooth (resp. projective) if
![]() $\Gamma $
is.
$\Gamma $
is.
Proof. By the existence of a G-linearized ample line bundle on
![]() $G/P$
and the descent theory for quasi-coherent sheaves,
$G/P$
and the descent theory for quasi-coherent sheaves,
![]() $\mathcal {E}_{f}(G/P)$
exists as a scheme. In fact, it is a closed subscheme of a projective bundle over
$\mathcal {E}_{f}(G/P)$
exists as a scheme. In fact, it is a closed subscheme of a projective bundle over
![]() $\mathbb {P}^1\times \Gamma $
. In particular,
$\mathbb {P}^1\times \Gamma $
. In particular,
![]() $\mathcal {E}_{f}(G/P)$
is separated and of finite type over
$\mathcal {E}_{f}(G/P)$
is separated and of finite type over
![]() $\mathbb {C}$
, and
$\mathbb {C}$
, and
![]() $\pi _f$
is projective. Observe that
$\pi _f$
is projective. Observe that
![]() $\mathcal {E}_{f}(G/P)$
becomes a trivial
$\mathcal {E}_{f}(G/P)$
becomes a trivial
![]() $G/P$
-bundle after a faithfully flat base change. This implies that
$G/P$
-bundle after a faithfully flat base change. This implies that
![]() $\mathcal {E}_{f}(G/P)$
is reduced, as it is the flat image of a reduced scheme, and that
$\mathcal {E}_{f}(G/P)$
is reduced, as it is the flat image of a reduced scheme, and that
![]() $\pi _f$
is smooth, as it becomes so after a faithfully flat base change. Finally,
$\pi _f$
is smooth, as it becomes so after a faithfully flat base change. Finally,
![]() $\mathcal {E}_{f}(G/P)$
is irreducible because
$\mathcal {E}_{f}(G/P)$
is irreducible because
![]() $\pi _f$
is smooth and has irreducible base and geometric fibers.
$\pi _f$
is smooth and has irreducible base and geometric fibers.
Let
![]() $\rho \in (Q^{\vee }/Q^{\vee }_P)^*$
. Recall the G-linearized line bundle
$\rho \in (Q^{\vee }/Q^{\vee }_P)^*$
. Recall the G-linearized line bundle
![]() $L_{\rho }:=G\times _P\mathbb {C}_{-\rho }$
on
$L_{\rho }:=G\times _P\mathbb {C}_{-\rho }$
on
![]() $G/P$
. Let
$G/P$
. Let
![]() $\operatorname {\mathrm {pr}}_2:\mathcal {E}_f\times G/P\rightarrow G/P$
denote the canonical projection. Then
$\operatorname {\mathrm {pr}}_2:\mathcal {E}_f\times G/P\rightarrow G/P$
denote the canonical projection. Then
![]() $\operatorname {\mathrm {pr}}_2^*L_{\rho }$
is naturally a G-linearized line bundle on
$\operatorname {\mathrm {pr}}_2^*L_{\rho }$
is naturally a G-linearized line bundle on
![]() $\mathcal {E}_f\times G/P$
with respect to the diagonal G-action, and hence, it descends to a line bundle on
$\mathcal {E}_f\times G/P$
with respect to the diagonal G-action, and hence, it descends to a line bundle on
![]() $\mathcal {E}_{f}(G/P)$
which we denote by
$\mathcal {E}_{f}(G/P)$
which we denote by
![]() $\mathcal {L}_{\rho }$
. It has a property that its restriction to every fiber of
$\mathcal {L}_{\rho }$
. It has a property that its restriction to every fiber of
![]() $\pi _f$
is isomorphic to
$\pi _f$
is isomorphic to
![]() $L_{\rho }$
.
$L_{\rho }$
.
Definition 3.3. We call
![]() $\beta \in H_2(\mathcal {E}_{f}(G/P))$
a section class of
$\beta \in H_2(\mathcal {E}_{f}(G/P))$
a section class of
![]() $\mathcal {E}_{f}(G/P)$
if
$\mathcal {E}_{f}(G/P)$
if
![]() $(\pi _f)_*\beta = [\mathbb {P}^1\times \gamma _0]$
for some
$(\pi _f)_*\beta = [\mathbb {P}^1\times \gamma _0]$
for some
![]() $\gamma _0\in \Gamma $
.
$\gamma _0\in \Gamma $
.
Definition 3.4. Define a function
characterized by the property that for any
![]() $\rho \in (Q^{\vee }/Q^{\vee }_P)^*$
,
$\rho \in (Q^{\vee }/Q^{\vee }_P)^*$
,
Let H be a subgroup of G. Suppose
![]() $\Gamma $
has an H-action. Then we have an obvious H-action on
$\Gamma $
has an H-action. Then we have an obvious H-action on
![]() $(\mathbb {P}^1\setminus 0)\times \Gamma \times G$
:
$(\mathbb {P}^1\setminus 0)\times \Gamma \times G$
:
Lemma 3.5. Suppose f is H-equivariant. Then the H-action on
![]() $\mathcal {E}_{f}|_{(\mathbb {P}^1\setminus 0)\times \Gamma }$
defined by (3.1) via
$\mathcal {E}_{f}|_{(\mathbb {P}^1\setminus 0)\times \Gamma }$
defined by (3.1) via
![]() $\nu _f$
extends to
$\nu _f$
extends to
![]() $\mathcal {E}_f$
and hence defines an H-action on
$\mathcal {E}_f$
and hence defines an H-action on
![]() $\mathcal {E}_{f}(G/P)$
.
$\mathcal {E}_{f}(G/P)$
.
Proof. Denote by
the action morphism and the canonical projection, respectively. Consider the following two
![]() $H\times \Gamma $
-points of
$H\times \Gamma $
-points of
![]() $\mathcal {G}r$
:
$\mathcal {G}r$
:
where
![]() $\widetilde {\nu }_f^o$
is the trivialization of
$\widetilde {\nu }_f^o$
is the trivialization of
![]() $(\operatorname {\mathrm {id}}_{\mathbb {A}^1_z}\times \operatorname {\mathrm {pr}}_{\Gamma })^*\mathcal {E}_f^o|_{(\mathbb {A}^1_z\setminus 0)\times H\times \Gamma }\simeq H\times \mathcal {E}_f^o|_{(\mathbb {A}^1_z\setminus 0)\times \Gamma }$
defined by
$(\operatorname {\mathrm {id}}_{\mathbb {A}^1_z}\times \operatorname {\mathrm {pr}}_{\Gamma })^*\mathcal {E}_f^o|_{(\mathbb {A}^1_z\setminus 0)\times H\times \Gamma }\simeq H\times \mathcal {E}_f^o|_{(\mathbb {A}^1_z\setminus 0)\times \Gamma }$
defined by
By the assumption that f is H-equivariant, these two
![]() $H\times \Gamma $
-points are equal. In other words, there exists an isomorphism between the underlying G-torsors which is compatible with the underlying trivializations. Therefore, the composition
$H\times \Gamma $
-points are equal. In other words, there exists an isomorphism between the underlying G-torsors which is compatible with the underlying trivializations. Therefore, the composition
gives the desired extension, where the last arrow is the canonical projection.
3.2 Moduli of sections
Let
![]() $f:\Gamma \rightarrow \mathcal {G}r$
be a morphism where
$f:\Gamma \rightarrow \mathcal {G}r$
be a morphism where
![]() $\Gamma $
is a smooth projective variety. Then
$\Gamma $
is a smooth projective variety. Then
![]() $\mathcal {E}_{f}(G/P)$
is a smooth projective variety by Lemma 3.2. The subvariety
$\mathcal {E}_{f}(G/P)$
is a smooth projective variety by Lemma 3.2. The subvariety
![]() $D_{f,\infty }:=\pi _f^{-1}(\infty \times \Gamma )$
is a smooth divisor of
$D_{f,\infty }:=\pi _f^{-1}(\infty \times \Gamma )$
is a smooth divisor of
![]() $\mathcal {E}_{f}(G/P)$
and is identified with
$\mathcal {E}_{f}(G/P)$
and is identified with
![]() $\Gamma \times G/P$
via the trivialization
$\Gamma \times G/P$
via the trivialization
![]() $\nu _f$
. Denote by
$\nu _f$
. Denote by
![]() $\iota _{f,\infty }:D_{f,\infty }\hookrightarrow \mathcal {E}_{f}(G/P)$
the inclusion.
$\iota _{f,\infty }:D_{f,\infty }\hookrightarrow \mathcal {E}_{f}(G/P)$
the inclusion.
Definition 3.6. Let
![]() $\eta \in Q^{\vee }/Q^{\vee }_P$
.
$\eta \in Q^{\vee }/Q^{\vee }_P$
.
-
1. Define
where $$\begin{align*}\overline{\mathcal{M}}(f,\eta):= \bigcup_{\beta} ~\overline{\mathcal{M}}_{0,1}(\mathcal{E}_{f}(G/P),\beta)\times_{(\operatorname{\mathrm{ev}}_1,\iota_{f,\infty})} D_{f,\infty},\end{align*}$$
$$\begin{align*}\overline{\mathcal{M}}(f,\eta):= \bigcup_{\beta} ~\overline{\mathcal{M}}_{0,1}(\mathcal{E}_{f}(G/P),\beta)\times_{(\operatorname{\mathrm{ev}}_1,\iota_{f,\infty})} D_{f,\infty},\end{align*}$$
 $\beta $
runs over all section classes of
$\beta $
runs over all section classes of
 $\mathcal {E}_{f}(G/P)$
such that
$\mathcal {E}_{f}(G/P)$
such that
 $c(\beta )=\eta $
(c is defined in Definition 3.4).
$c(\beta )=\eta $
(c is defined in Definition 3.4).
-
2. Define
to be the composition $$\begin{align*}\operatorname{\mathrm{ev}}:\overline{\mathcal{M}}(f,\eta)\rightarrow G/P\end{align*}$$
of the morphism induced by
$$\begin{align*}\operatorname{\mathrm{ev}}:\overline{\mathcal{M}}(f,\eta)\rightarrow G/P\end{align*}$$
of the morphism induced by $$\begin{align*}\overline{\mathcal{M}}(f,\eta)\rightarrow D_{f,\infty}\simeq \Gamma\times G/P \rightarrow G/P\end{align*}$$
$$\begin{align*}\overline{\mathcal{M}}(f,\eta)\rightarrow D_{f,\infty}\simeq \Gamma\times G/P \rightarrow G/P\end{align*}$$
 $\operatorname {\mathrm {ev}}_1$
, the isomorphism induced by
$\operatorname {\mathrm {ev}}_1$
, the isomorphism induced by
 $\nu _f$
and the canonical projection.
$\nu _f$
and the canonical projection.
Lemma 3.7. The virtual dimension of
![]() $\overline {\mathcal {M}}(f,\eta )$
is equal to
$\overline {\mathcal {M}}(f,\eta )$
is equal to
![]() $\dim \Gamma +\dim G/P +\sum _{\alpha \in R^+\setminus R^+_P}\alpha (\eta )$
.
$\dim \Gamma +\dim G/P +\sum _{\alpha \in R^+\setminus R^+_P}\alpha (\eta )$
.
Proof. Denote by
![]() $\operatorname {\mathrm {vdim}}\overline {\mathcal {M}}(f,\eta )$
the virtual dimension of
$\operatorname {\mathrm {vdim}}\overline {\mathcal {M}}(f,\eta )$
the virtual dimension of
![]() $\overline {\mathcal {M}}(f,\eta )$
. Let
$\overline {\mathcal {M}}(f,\eta )$
. Let
![]() $\beta $
be a section class of
$\beta $
be a section class of
![]() $\mathcal {E}_{f}(G/P)$
such that
$\mathcal {E}_{f}(G/P)$
such that
![]() $c(\beta )=\eta $
. We have
$c(\beta )=\eta $
. We have
Since
![]() $\mathcal {E}_{f}(G/P)$
is a
$\mathcal {E}_{f}(G/P)$
is a
![]() $G/P$
-bundle over
$G/P$
-bundle over
![]() $\mathbb {P}^1\times \Gamma $
and
$\mathbb {P}^1\times \Gamma $
and
![]() $\beta $
is a section class, the equality (3.2) can be simplified to
$\beta $
is a section class, the equality (3.2) can be simplified to
where
![]() $\mathcal {T}^{vert}_{\pi _f}$
is the vertical tangent bundle of
$\mathcal {T}^{vert}_{\pi _f}$
is the vertical tangent bundle of
![]() $\pi _f$
.
$\pi _f$
.
It remains to show
![]() $\langle \beta ,c_1(\mathcal {T}^{vert}_{\pi _f})\rangle =\sum _{\alpha \in R^+\setminus R_P^+}\alpha (\eta )$
. Recall
$\langle \beta ,c_1(\mathcal {T}^{vert}_{\pi _f})\rangle =\sum _{\alpha \in R^+\setminus R_P^+}\alpha (\eta )$
. Recall
![]() $\bigwedge ^{top}\mathcal {T}_{G/P}\simeq L_{\rho _P}$
as G-linearized line bundles, where
$\bigwedge ^{top}\mathcal {T}_{G/P}\simeq L_{\rho _P}$
as G-linearized line bundles, where
![]() $\rho _P:=\sum _{\alpha \in R^+\setminus R_P^+}\alpha $
. It follows that
$\rho _P:=\sum _{\alpha \in R^+\setminus R_P^+}\alpha $
. It follows that
![]() $\bigwedge ^{top}\mathcal {T}^{vert}_{\pi _f}\simeq \mathcal {L}_{\rho _P}$
, and hence,
$\bigwedge ^{top}\mathcal {T}^{vert}_{\pi _f}\simeq \mathcal {L}_{\rho _P}$
, and hence,
 $$\begin{align*}\langle\beta,c_1(\mathcal{T}^{vert}_{\pi_f})\rangle=\langle\beta,c_1({\textstyle \bigwedge^{top}}\mathcal{T}^{vert}_{\pi_f})\rangle=\langle\beta,c_1(\mathcal{L}_{\rho_P})\rangle=\langle\eta,\rho_P\rangle=\sum_{\alpha\in R^+\setminus R_P^+}\alpha(\eta).\\[-34pt]\end{align*}$$
$$\begin{align*}\langle\beta,c_1(\mathcal{T}^{vert}_{\pi_f})\rangle=\langle\beta,c_1({\textstyle \bigwedge^{top}}\mathcal{T}^{vert}_{\pi_f})\rangle=\langle\beta,c_1(\mathcal{L}_{\rho_P})\rangle=\langle\eta,\rho_P\rangle=\sum_{\alpha\in R^+\setminus R_P^+}\alpha(\eta).\\[-34pt]\end{align*}$$
Lemma 3.8. Let H be a subgroup of G. Suppose
![]() $\Gamma $
has an H-action and f is H-equivariant. Then for any
$\Gamma $
has an H-action and f is H-equivariant. Then for any
![]() $\eta \in Q^{\vee }/Q^{\vee }_P$
, the stack
$\eta \in Q^{\vee }/Q^{\vee }_P$
, the stack
![]() $\overline {\mathcal {M}}(f,\eta )$
has a natural H-action such that
$\overline {\mathcal {M}}(f,\eta )$
has a natural H-action such that
![]() $\operatorname {\mathrm {ev}}$
is H-equivariant.
$\operatorname {\mathrm {ev}}$
is H-equivariant.
Proof. This follows immediately from Lemma 3.5.
Definition 3.9.
-
1. For any
 $wt_{\lambda }\in W_{af}^-$
, define
$wt_{\lambda }\in W_{af}^-$
, define
 $\overline {\mathcal {M}}(wt_{\lambda },\eta )$
to be the moduli space
$\overline {\mathcal {M}}(wt_{\lambda },\eta )$
to be the moduli space
 $\overline {\mathcal {M}}(f,\eta )$
in Definition 3.6 by taking
$\overline {\mathcal {M}}(f,\eta )$
in Definition 3.6 by taking
 $f=f_{\mathcal {G}r,wt_{\lambda }}$
(Definition 2.5).
$f=f_{\mathcal {G}r,wt_{\lambda }}$
(Definition 2.5). -
2. For any
 $\mu \in Q^{\vee }$
, define
$\mu \in Q^{\vee }$
, define
 $\overline {\mathcal {M}}(\mu ,\eta )$
to be the moduli space
$\overline {\mathcal {M}}(\mu ,\eta )$
to be the moduli space
 $\overline {\mathcal {M}}(f,\eta )$
in Definition 3.6 by taking
$\overline {\mathcal {M}}(f,\eta )$
in Definition 3.6 by taking
 $f= t^{\mu }$
(point map).
$f= t^{\mu }$
(point map).
By Lemma 3.8,
![]() $\overline {\mathcal {M}}(wt_{\lambda },\eta )$
(resp.
$\overline {\mathcal {M}}(wt_{\lambda },\eta )$
(resp.
![]() $\overline {\mathcal {M}}(\mu ,\eta )$
) has a natural
$\overline {\mathcal {M}}(\mu ,\eta )$
) has a natural
![]() $B^-$
-action (resp. T-action) such that
$B^-$
-action (resp. T-action) such that
![]() $\operatorname {\mathrm {ev}}$
is
$\operatorname {\mathrm {ev}}$
is
![]() $B^-$
-equivariant (resp. T-equivariant).
$B^-$
-equivariant (resp. T-equivariant).
3.3 Construction of the Savelyev-Seidel homomorphism
Definition 3.10. Define an
![]() $H_T^{\bullet }(\operatorname {\mathrm {pt}})$
-linear map
$H_T^{\bullet }(\operatorname {\mathrm {pt}})$
-linear map
 $$\begin{align*}\begin{array}{cccc} \Phi_{SS}: & H^T_{-\bullet}(\mathcal{G}r) &\rightarrow& QH_T^{\bullet}(G/P)[q_i^{-1}|~i\in I\setminus I_P]\\ &\xi_{wt_{\lambda}} &\mapsto & \displaystyle \sum_{v\in W^P}\sum_{\eta\in Q^{\vee}/Q^{\vee}_P} q^{\eta} \left(\int_{[\overline{\mathcal{M}}(wt_{\lambda},\eta)]^{vir}}\operatorname{\mathrm{ev}}^*\sigma^v\right) \sigma_v \end{array}. \end{align*}$$
$$\begin{align*}\begin{array}{cccc} \Phi_{SS}: & H^T_{-\bullet}(\mathcal{G}r) &\rightarrow& QH_T^{\bullet}(G/P)[q_i^{-1}|~i\in I\setminus I_P]\\ &\xi_{wt_{\lambda}} &\mapsto & \displaystyle \sum_{v\in W^P}\sum_{\eta\in Q^{\vee}/Q^{\vee}_P} q^{\eta} \left(\int_{[\overline{\mathcal{M}}(wt_{\lambda},\eta)]^{vir}}\operatorname{\mathrm{ev}}^*\sigma^v\right) \sigma_v \end{array}. \end{align*}$$
Remark 3.11. At this stage, we should take the coefficient ring to be
![]() $\mathbb {Q}$
. But we will prove at the end that we can actually take it to be
$\mathbb {Q}$
. But we will prove at the end that we can actually take it to be
![]() $\mathbb {Z}$
. See Theorem 4.9.
$\mathbb {Z}$
. See Theorem 4.9.
Proposition 3.12.
![]() $\Phi _{SS}$
is a graded homomorphism of
$\Phi _{SS}$
is a graded homomorphism of
![]() $H_T^{\bullet }(\operatorname {\mathrm {pt}})$
-algebras.
$H_T^{\bullet }(\operatorname {\mathrm {pt}})$
-algebras.
Proof. Let us first show that
![]() $\Phi _{SS}$
is graded. Let
$\Phi _{SS}$
is graded. Let
![]() $wt_{\lambda }\in W_{af}^-$
. Then
$wt_{\lambda }\in W_{af}^-$
. Then
![]() $\xi _{wt_{\lambda }}$
has degree
$\xi _{wt_{\lambda }}$
has degree
![]() $-2\ell (wt_{\lambda })$
in
$-2\ell (wt_{\lambda })$
in
![]() $H^T_{-\bullet }(\mathcal {G}r)$
. By Lemma 3.7, the integral
$H^T_{-\bullet }(\mathcal {G}r)$
. By Lemma 3.7, the integral
![]() $\int _{[\overline {\mathcal {M}}(wt_{\lambda },\eta )]^{vir}}\operatorname {\mathrm {ev}}^*\sigma ^v$
is nonzero only if
$\int _{[\overline {\mathcal {M}}(wt_{\lambda },\eta )]^{vir}}\operatorname {\mathrm {ev}}^*\sigma ^v$
is nonzero only if
 $$\begin{align*}\dim G/P -\ell(v)=\ell(wt_{\lambda})+\dim G/P +\sum_{\alpha\in R^+\setminus R^+_P}\alpha(\eta) .\end{align*}$$
$$\begin{align*}\dim G/P -\ell(v)=\ell(wt_{\lambda})+\dim G/P +\sum_{\alpha\in R^+\setminus R^+_P}\alpha(\eta) .\end{align*}$$
By definition,
![]() $q^{\eta }$
has degree
$q^{\eta }$
has degree
![]() $2\sum _{\alpha \in R^+\setminus R^+_P}\alpha (\eta )$
. This shows that
$2\sum _{\alpha \in R^+\setminus R^+_P}\alpha (\eta )$
. This shows that
![]() $\Phi _{SS}(\xi _{wt_{\lambda }})$
has degree
$\Phi _{SS}(\xi _{wt_{\lambda }})$
has degree
![]() $-2\ell (wt_{\lambda })$
, as desired.
$-2\ell (wt_{\lambda })$
, as desired.
It remains to show that
![]() $\Phi _{SS}$
is an algebra homomorphism. Define a
$\Phi _{SS}$
is an algebra homomorphism. Define a
![]() $\operatorname {\mathrm {Frac}}(H_T^{\bullet }(\operatorname {\mathrm {pt}}))$
-linear map
$\operatorname {\mathrm {Frac}}(H_T^{\bullet }(\operatorname {\mathrm {pt}}))$
-linear map
 $$ \begin{align} \begin{array}{cccc} \Phi_{SS}': & H^T_{-\bullet}(\mathcal{G}r)_{loc} &\rightarrow& QH_T^{\bullet}(G/P)[q_i^{-1}|~i\in I\setminus I_P]_{loc}\\ &[t^{\mu}] &\mapsto & \displaystyle \sum_{v\in W^P}\sum_{\eta\in Q^{\vee}/Q^{\vee}_P} q^{\eta} \left(\int_{[\overline{\mathcal{M}}(\mu,\eta)]^{vir}}\operatorname{\mathrm{ev}}^*\sigma^v\right) \sigma_v, \end{array} \end{align} $$
$$ \begin{align} \begin{array}{cccc} \Phi_{SS}': & H^T_{-\bullet}(\mathcal{G}r)_{loc} &\rightarrow& QH_T^{\bullet}(G/P)[q_i^{-1}|~i\in I\setminus I_P]_{loc}\\ &[t^{\mu}] &\mapsto & \displaystyle \sum_{v\in W^P}\sum_{\eta\in Q^{\vee}/Q^{\vee}_P} q^{\eta} \left(\int_{[\overline{\mathcal{M}}(\mu,\eta)]^{vir}}\operatorname{\mathrm{ev}}^*\sigma^v\right) \sigma_v, \end{array} \end{align} $$
where the subscript
![]() $loc$
denotes the localization
$loc$
denotes the localization
![]() $-\otimes _{H_T^{\bullet }(\operatorname {\mathrm {pt}})}\operatorname {\mathrm {Frac}}(H_T^{\bullet }(\operatorname {\mathrm {pt}}))$
. By (2.3) and Lemma 3.14 below, it suffices to show
$-\otimes _{H_T^{\bullet }(\operatorname {\mathrm {pt}})}\operatorname {\mathrm {Frac}}(H_T^{\bullet }(\operatorname {\mathrm {pt}}))$
. By (2.3) and Lemma 3.14 below, it suffices to show
for any
![]() $wt_{\lambda }\in W_{af}^-$
. Put
$wt_{\lambda }\in W_{af}^-$
. Put
![]() $\Gamma :=\Gamma _{wt_{\lambda }}$
, the source of
$\Gamma :=\Gamma _{wt_{\lambda }}$
, the source of
![]() $f_{\mathcal {G}r,wt_{\lambda }}$
. To simplify the exposition, assume
$f_{\mathcal {G}r,wt_{\lambda }}$
. To simplify the exposition, assume
![]() $\Gamma ^T$
is discrete.Footnote
2
By the classical localization formula and the assumption that
$\Gamma ^T$
is discrete.Footnote
2
By the classical localization formula and the assumption that
![]() $f_{\mathcal {G}r,wt_{\lambda }}$
is the composition of a T-equivariant resolution
$f_{\mathcal {G}r,wt_{\lambda }}$
is the composition of a T-equivariant resolution
![]() $\Gamma \rightarrow \overline {\mathcal {B}\cdot t^{w(\lambda )}}$
and the inclusion
$\Gamma \rightarrow \overline {\mathcal {B}\cdot t^{w(\lambda )}}$
and the inclusion
![]() $\overline {\mathcal {B}\cdot t^{w(\lambda )}}\hookrightarrow \mathcal {G}r$
, we have
$\overline {\mathcal {B}\cdot t^{w(\lambda )}}\hookrightarrow \mathcal {G}r$
, we have
 $$\begin{align*}\xi_{wt_{\lambda}} = (f_{\mathcal{G}r,wt_{\lambda}})_*[\Gamma]= \sum_{\gamma\in\Gamma^T} \frac{1}{e^T(T_{\gamma}\Gamma)} [t^{\mu_{\gamma}}],\end{align*}$$
$$\begin{align*}\xi_{wt_{\lambda}} = (f_{\mathcal{G}r,wt_{\lambda}})_*[\Gamma]= \sum_{\gamma\in\Gamma^T} \frac{1}{e^T(T_{\gamma}\Gamma)} [t^{\mu_{\gamma}}],\end{align*}$$
where
![]() $\mu _{\gamma }\in Q^{\vee }$
satisfies
$\mu _{\gamma }\in Q^{\vee }$
satisfies
![]() $f_{\mathcal {G}r,wt_{\lambda }}\circ \gamma =t^{\mu _{\gamma }}$
. (Here,
$f_{\mathcal {G}r,wt_{\lambda }}\circ \gamma =t^{\mu _{\gamma }}$
. (Here,
![]() $\gamma $
and
$\gamma $
and
![]() $t^{\mu _{\gamma }}$
are viewed as morphisms from
$t^{\mu _{\gamma }}$
are viewed as morphisms from
![]() $\operatorname {\mathrm {Spec}}\mathbb {C}$
to
$\operatorname {\mathrm {Spec}}\mathbb {C}$
to
![]() $\Gamma $
and
$\Gamma $
and
![]() $\mathcal {G}r$
, respectively.) Put
$\mathcal {G}r$
, respectively.) Put
![]() $\overline {\mathcal {M}}:=\overline {\mathcal {M}}(wt_{\lambda },\eta )$
and
$\overline {\mathcal {M}}:=\overline {\mathcal {M}}(wt_{\lambda },\eta )$
and
![]() $\overline {\mathcal {M}}_{\gamma }:=\overline {\mathcal {M}}(f_{\mathcal {G}r,wt_{\lambda }}\circ \gamma ,\eta )\simeq \overline {\mathcal {M}}(\mu _{\gamma },\eta )$
. Let
$\overline {\mathcal {M}}_{\gamma }:=\overline {\mathcal {M}}(f_{\mathcal {G}r,wt_{\lambda }}\circ \gamma ,\eta )\simeq \overline {\mathcal {M}}(\mu _{\gamma },\eta )$
. Let
![]() $\{F_{\gamma ,j}\}_{j\in J_{\gamma }}$
be the set of components of the fixed-point substack
$\{F_{\gamma ,j}\}_{j\in J_{\gamma }}$
be the set of components of the fixed-point substack
![]() $\overline {\mathcal {M}}_{\gamma }^T$
. We have
$\overline {\mathcal {M}}_{\gamma }^T$
. We have
 $$\begin{align*}\overline{\mathcal{M}}^T=\bigcup_{\gamma\in \Gamma^T}\overline{\mathcal{M}}_{\gamma}^T=\bigcup_{\gamma\in\Gamma^T}\bigcup_{j\in J_{\gamma}} F_{\gamma,j}.\end{align*}$$
$$\begin{align*}\overline{\mathcal{M}}^T=\bigcup_{\gamma\in \Gamma^T}\overline{\mathcal{M}}_{\gamma}^T=\bigcup_{\gamma\in\Gamma^T}\bigcup_{j\in J_{\gamma}} F_{\gamma,j}.\end{align*}$$
Applying the virtual localization formula [Reference Graber and Pandharipande11] twice, we get
 $$\begin{align*}[\overline{\mathcal{M}}]^{vir} = \sum_{\gamma\in \Gamma^T} \sum_{j\in J_{\gamma}} \frac{[F_{\gamma,j}]^{vir}}{e^T(N^{vir}_{F_{\gamma,j}/\overline{\mathcal{M}}})} = \sum_{\gamma\in \Gamma^T} \frac{1}{e^T(T_{\gamma} \Gamma)}\left( \sum_{j\in J_{\gamma}} \frac{[F_{\gamma,j}]^{vir}}{e^T(N^{vir}_{F_{\gamma,j}/\overline{\mathcal{M}}_{\gamma}})} \right) = \sum_{\gamma\in \Gamma^T} \frac{1}{e^T(T_{\gamma} \Gamma)} [\overline{\mathcal{M}}_{\gamma}]^{vir}.\end{align*}$$
$$\begin{align*}[\overline{\mathcal{M}}]^{vir} = \sum_{\gamma\in \Gamma^T} \sum_{j\in J_{\gamma}} \frac{[F_{\gamma,j}]^{vir}}{e^T(N^{vir}_{F_{\gamma,j}/\overline{\mathcal{M}}})} = \sum_{\gamma\in \Gamma^T} \frac{1}{e^T(T_{\gamma} \Gamma)}\left( \sum_{j\in J_{\gamma}} \frac{[F_{\gamma,j}]^{vir}}{e^T(N^{vir}_{F_{\gamma,j}/\overline{\mathcal{M}}_{\gamma}})} \right) = \sum_{\gamma\in \Gamma^T} \frac{1}{e^T(T_{\gamma} \Gamma)} [\overline{\mathcal{M}}_{\gamma}]^{vir}.\end{align*}$$
It follows that
 $$ \begin{align*} \Phi_{SS}(\xi_{wt_{\lambda}}) &= \sum_{v\in W^P}\sum_{\eta\in Q^{\vee}/Q^{\vee}_P} q^{\eta} \left(\int_{[\overline{\mathcal{M}}(wt_{\lambda},\eta)]^{vir}}\operatorname{\mathrm{ev}}^*\sigma^v\right) \sigma_v\\ &= \sum_{v\in W^P}\sum_{\eta\in Q^{\vee}/Q^{\vee}_P} \sum_{\gamma\in\Gamma^T} \frac{q^{\eta}}{ e^T(T_{\gamma}\Gamma)} \left(\int_{[\overline{\mathcal{M}}_{\gamma}]^{vir}}\operatorname{\mathrm{ev}}^*\sigma^v\right) \sigma_v\\ &= \sum_{\gamma\in\Gamma^T} \frac{1}{ e^T(T_{\gamma}\Gamma)}\sum_{v\in W^P}\sum_{\eta\in Q^{\vee}/Q^{\vee}_P} q^{\eta} \left(\int_{[\overline{\mathcal{M}}(\mu_{\gamma},\eta)]^{vir}}\operatorname{\mathrm{ev}}^*\sigma^v\right) \sigma_v\\ &= \sum_{\gamma\in\Gamma^T} \frac{1}{ e^T(T_{\gamma}\Gamma)} \Phi_{SS}'([t^{\mu_{\gamma}}])\\ &= \Phi_{SS}'\left( \sum_{\gamma\in\Gamma^T} \frac{1}{e^T(T_{\gamma}\Gamma)} [t^{\mu_{\gamma}}] \right) = \Phi_{SS}'(\xi_{wt_{\lambda}}), \end{align*} $$
$$ \begin{align*} \Phi_{SS}(\xi_{wt_{\lambda}}) &= \sum_{v\in W^P}\sum_{\eta\in Q^{\vee}/Q^{\vee}_P} q^{\eta} \left(\int_{[\overline{\mathcal{M}}(wt_{\lambda},\eta)]^{vir}}\operatorname{\mathrm{ev}}^*\sigma^v\right) \sigma_v\\ &= \sum_{v\in W^P}\sum_{\eta\in Q^{\vee}/Q^{\vee}_P} \sum_{\gamma\in\Gamma^T} \frac{q^{\eta}}{ e^T(T_{\gamma}\Gamma)} \left(\int_{[\overline{\mathcal{M}}_{\gamma}]^{vir}}\operatorname{\mathrm{ev}}^*\sigma^v\right) \sigma_v\\ &= \sum_{\gamma\in\Gamma^T} \frac{1}{ e^T(T_{\gamma}\Gamma)}\sum_{v\in W^P}\sum_{\eta\in Q^{\vee}/Q^{\vee}_P} q^{\eta} \left(\int_{[\overline{\mathcal{M}}(\mu_{\gamma},\eta)]^{vir}}\operatorname{\mathrm{ev}}^*\sigma^v\right) \sigma_v\\ &= \sum_{\gamma\in\Gamma^T} \frac{1}{ e^T(T_{\gamma}\Gamma)} \Phi_{SS}'([t^{\mu_{\gamma}}])\\ &= \Phi_{SS}'\left( \sum_{\gamma\in\Gamma^T} \frac{1}{e^T(T_{\gamma}\Gamma)} [t^{\mu_{\gamma}}] \right) = \Phi_{SS}'(\xi_{wt_{\lambda}}), \end{align*} $$
as desired.
Remark 3.13. In the proof of Proposition 3.12, we have used the fact that
![]() $\pi _*[X]=[Y]\in H^T_{BM,\dim _{\mathbb {R}}Y}(Y)$
for any T-equivariant proper birational morphism
$\pi _*[X]=[Y]\in H^T_{BM,\dim _{\mathbb {R}}Y}(Y)$
for any T-equivariant proper birational morphism
![]() $\pi :X\rightarrow Y$
between possibly singular T-varieties over
$\pi :X\rightarrow Y$
between possibly singular T-varieties over
![]() $\mathbb {C}$
. For reader’s convenience, we provide a proof.
$\mathbb {C}$
. For reader’s convenience, we provide a proof.
Let us first deal with the non-equivariant case. Since
![]() $\pi $
is proper, the pushforward map
$\pi $
is proper, the pushforward map
![]() $\pi _*:A_{\bullet }(X)\rightarrow A_{\bullet }(Y)$
between Chow groups exists. Since
$\pi _*:A_{\bullet }(X)\rightarrow A_{\bullet }(Y)$
between Chow groups exists. Since
![]() $\pi $
is birational, it has degree one, and hence,
$\pi $
is birational, it has degree one, and hence,
![]() $\pi _*[X]=[Y]$
, where
$\pi _*[X]=[Y]$
, where
![]() $[X]\in A_n(X)$
and
$[X]\in A_n(X)$
and
![]() $[Y]\in A_n(Y)$
(
$[Y]\in A_n(Y)$
(
![]() $n:=\dim _{\mathbb {C}}X=\dim _{\mathbb {C}}Y$
) are the fundamental cycles. The desired equality (in Borel-Moore homology) now follows from this equality, the existence of the cycle map
$n:=\dim _{\mathbb {C}}X=\dim _{\mathbb {C}}Y$
) are the fundamental cycles. The desired equality (in Borel-Moore homology) now follows from this equality, the existence of the cycle map
![]() $c\ell :A_{\bullet }(-)\rightarrow H_{BM,2\bullet }(-)$
and the fact that
$c\ell :A_{\bullet }(-)\rightarrow H_{BM,2\bullet }(-)$
and the fact that
![]() $c\ell $
commutes with
$c\ell $
commutes with
![]() $\pi _*$
. See [Reference Anderson and Fulton1, Chapter 17] or [Reference Fulton7] for more details.
$\pi _*$
. See [Reference Anderson and Fulton1, Chapter 17] or [Reference Fulton7] for more details.
For the equivariant case, apply the above result to the morphism
![]() $X\times ^T U\rightarrow Y\times ^T U$
for a suitable finite dimensional approximation
$X\times ^T U\rightarrow Y\times ^T U$
for a suitable finite dimensional approximation
![]() $U\rightarrow U/T$
of the classifying bundle
$U\rightarrow U/T$
of the classifying bundle
![]() $ET\rightarrow BT$
. See [Reference Edidin and Graham6, Section 2.2] for more details.
$ET\rightarrow BT$
. See [Reference Edidin and Graham6, Section 2.2] for more details.
Lemma 3.14. The map
![]() $\Phi _{SS}'$
defined in (3.3) satisfies
$\Phi _{SS}'$
defined in (3.3) satisfies
for any
![]() $\mu _1, \mu _2\in Q^{\vee }$
.
$\mu _1, \mu _2\in Q^{\vee }$
.
Proof. Notice that each
![]() $\Phi _{SS}'([t^{\mu }])$
is a T-equivariant Seidel element. Seidel elements are originally introduced by Seidel in [Reference Seidel29]. Their T-equivariant generalizations are introduced in [Reference Braverman, Maulik and Okounkov3, Reference Iritani13, Reference Maulik and Okounkov20, Reference Okounkov and Pandharipande23] in algebraic geometry and in [Reference González, Mak and Pomerleano10, Reference Liebenschutz-Jones19] in symplectic geometry.
$\Phi _{SS}'([t^{\mu }])$
is a T-equivariant Seidel element. Seidel elements are originally introduced by Seidel in [Reference Seidel29]. Their T-equivariant generalizations are introduced in [Reference Braverman, Maulik and Okounkov3, Reference Iritani13, Reference Maulik and Okounkov20, Reference Okounkov and Pandharipande23] in algebraic geometry and in [Reference González, Mak and Pomerleano10, Reference Liebenschutz-Jones19] in symplectic geometry.
Consider the one
![]() $S_{\mu }(0):=S_{\mu }(\tau )|_{\tau = 0}$
defined by Iritani in [Reference Iritani13, Definition 3.17]. (More precisely, what he defined are T-equivariant big Seidel elements. Since we are dealing with T-equivariant small Seidel elements, we put
$S_{\mu }(0):=S_{\mu }(\tau )|_{\tau = 0}$
defined by Iritani in [Reference Iritani13, Definition 3.17]. (More precisely, what he defined are T-equivariant big Seidel elements. Since we are dealing with T-equivariant small Seidel elements, we put
![]() $\tau =0$
.) In terms of our notations, we have
$\tau =0$
.) In terms of our notations, we have
 $$\begin{align*}S_{\mu}(0):= \sum_{v\in W^P}\sum_{\eta\in Q^{\vee}/Q^{\vee}_P} q^{\eta - c([u^{min}_{\mu}])} \left(\int_{[\overline{\mathcal{M}}(\mu,\eta)]^{vir}}\operatorname{\mathrm{ev}}^*\sigma^v\right) \sigma_v= q^{-c([u^{min}_{\mu}])}\Phi_{SS}'([t^{\mu}]),\end{align*}$$
$$\begin{align*}S_{\mu}(0):= \sum_{v\in W^P}\sum_{\eta\in Q^{\vee}/Q^{\vee}_P} q^{\eta - c([u^{min}_{\mu}])} \left(\int_{[\overline{\mathcal{M}}(\mu,\eta)]^{vir}}\operatorname{\mathrm{ev}}^*\sigma^v\right) \sigma_v= q^{-c([u^{min}_{\mu}])}\Phi_{SS}'([t^{\mu}]),\end{align*}$$
where
![]() $u^{min}_{\mu }$
is a minimal section of
$u^{min}_{\mu }$
is a minimal section of
![]() $\mathcal {E}_{t^{\mu }}(G/P)$
which is defined between Lemma 3.5 and Lemma 3.6 in op. cit. and c is the function defined in Definition 3.4 in the present paper.
$\mathcal {E}_{t^{\mu }}(G/P)$
which is defined between Lemma 3.5 and Lemma 3.6 in op. cit. and c is the function defined in Definition 3.4 in the present paper.
By the discussion following [Reference Iritani13, Definition 3.17], we have
where
![]() $[u^{min}_{\mu _1}]\#[u^{min}_{\mu _2}]$
is the section class of
$[u^{min}_{\mu _1}]\#[u^{min}_{\mu _2}]$
is the section class of
![]() $\mathcal {E}_{t^{\mu _1+\mu _2}}(G/P)$
obtained by gluing the sections
$\mathcal {E}_{t^{\mu _1+\mu _2}}(G/P)$
obtained by gluing the sections
![]() $u^{min}_{\mu _1}$
and
$u^{min}_{\mu _1}$
and
![]() $u^{min}_{\mu _2}$
through the following ‘degeneration’ (see the proof of [Reference Iritani13, Corollary 3.16])
$u^{min}_{\mu _2}$
through the following ‘degeneration’ (see the proof of [Reference Iritani13, Corollary 3.16])
Here, the
![]() $\mathbb {G}_m\times \mathbb {G}_m$
-action is defined by
$\mathbb {G}_m\times \mathbb {G}_m$
-action is defined by
(We call
![]() $\mathcal {E}_{\mu _1,\mu _2}$
a degeneration because it is a
$\mathcal {E}_{\mu _1,\mu _2}$
a degeneration because it is a
![]() $G/P$
-bundle over
$G/P$
-bundle over
![]() $\mathbb {P}^1\times \mathbb {P}^1$
and satisfies
$\mathbb {P}^1\times \mathbb {P}^1$
and satisfies
where
![]() $\Delta \subset \mathbb {P}^1\times \mathbb {P}^1$
is the diagonal.)
$\Delta \subset \mathbb {P}^1\times \mathbb {P}^1$
is the diagonal.)
The equality (3.4) will be proved if we can show
This follows from the observation that for each
![]() $\rho \in (Q^{\vee }/Q^{\vee }_P)^*$
, the line bundle
$\rho \in (Q^{\vee }/Q^{\vee }_P)^*$
, the line bundle
on
![]() $\mathcal {E}_{\mu _1,\mu _2}$
restricts to
$\mathcal {E}_{\mu _1,\mu _2}$
restricts to
![]() $\mathcal {L}_{\rho }$
over
$\mathcal {L}_{\rho }$
over
![]() $\mathcal {E}_{\mu _1,\mu _2}|_{\mathbb {P}^1\times [1:0]} $
,
$\mathcal {E}_{\mu _1,\mu _2}|_{\mathbb {P}^1\times [1:0]} $
,
![]() $\mathcal {E}_{\mu _1,\mu _2}|_{\mathbb {P}^1\times [0:1]} $
and
$\mathcal {E}_{\mu _1,\mu _2}|_{\mathbb {P}^1\times [0:1]} $
and
![]() $\mathcal {E}_{\mu _1,\mu _2}|_{\Delta }$
.
$\mathcal {E}_{\mu _1,\mu _2}|_{\Delta }$
.
Remark 3.15. The author of the present paper did not know Iritani’s result until he read a paper of González, Mak and Pomerleano [Reference González, Mak and Pomerleano10]. In the original version, Lemma 3.14 was proved using Li’s degeneration formula [Reference Li17, Reference Li18]. The degeneration used was essentially the fiber bundle
![]() $\mathcal {E}_{\mu _1,\mu _2}$
constructed by Iritani. Notice, however, that Iritani’s proof does not rely on the degeneration formula but virtual localization formula.
$\mathcal {E}_{\mu _1,\mu _2}$
constructed by Iritani. Notice, however, that Iritani’s proof does not rely on the degeneration formula but virtual localization formula.
4 Proof of main result
4.1 T-invariant sections
Let
![]() $\mu \in Q^{\vee }$
. Recall
$\mu \in Q^{\vee }$
. Recall
![]() $\mathcal {E}_{t^{\mu }}(G/P)$
is the
$\mathcal {E}_{t^{\mu }}(G/P)$
is the
![]() $G/P$
-bundle
$G/P$
-bundle
![]() $\mathcal {E}_{f}(G/P)$
where we take
$\mathcal {E}_{f}(G/P)$
where we take
![]() $f=t^{\mu }$
. By definition, we have
$f=t^{\mu }$
. By definition, we have
Every
![]() $v\in W^P$
gives rise to a T-invariant section
$v\in W^P$
gives rise to a T-invariant section
![]() $u_{\mu ,v}$
of
$u_{\mu ,v}$
of
![]() $\mathcal {E}_{t^{\mu }}(G/P)$
defined by
$\mathcal {E}_{t^{\mu }}(G/P)$
defined by
It is easy to see that all T-invariant sections of
![]() $\mathcal {E}_{t^{\mu }}(G/P)$
arise in this way.
$\mathcal {E}_{t^{\mu }}(G/P)$
arise in this way.
Let
![]() $v\in W^P$
. By linearizing the G-action on
$v\in W^P$
. By linearizing the G-action on
![]() $G/P$
at
$G/P$
at
![]() $y_v$
, we obtain an isomorphism
$y_v$
, we obtain an isomorphism
 $$\begin{align*}T_{y_v}(G/P)\simeq \bigoplus_{\alpha\in -v(R^+\setminus R_P^+)}\mathfrak{g}_{\alpha}\end{align*}$$
$$\begin{align*}T_{y_v}(G/P)\simeq \bigoplus_{\alpha\in -v(R^+\setminus R_P^+)}\mathfrak{g}_{\alpha}\end{align*}$$
of T-modules.
Lemma 4.1. Let
![]() $\mathcal {T}^{vert}$
be the vertical tangent bundle of the
$\mathcal {T}^{vert}$
be the vertical tangent bundle of the
![]() $G/P$
-bundle
$G/P$
-bundle
![]() $\mathcal {E}_{t^{\mu }}(G/P)\rightarrow \mathbb {P}^1$
. Then
$\mathcal {E}_{t^{\mu }}(G/P)\rightarrow \mathbb {P}^1$
. Then
![]() $u_{\mu ,v}^*\mathcal {T}^{vert}$
is defined by the transition matrix
$u_{\mu ,v}^*\mathcal {T}^{vert}$
is defined by the transition matrix
 $$\begin{align*}A(z):= \sum_{\alpha\in -v(R^+\setminus R_P^+)} z^{\alpha(\mu)}\operatorname{\mathrm{id}}_{\mathfrak{g}_{\alpha}}\in\operatorname{\mathrm{End}}(T_{y_v}(G/P))[z,z^{-1}]. \end{align*}$$
$$\begin{align*}A(z):= \sum_{\alpha\in -v(R^+\setminus R_P^+)} z^{\alpha(\mu)}\operatorname{\mathrm{id}}_{\mathfrak{g}_{\alpha}}\in\operatorname{\mathrm{End}}(T_{y_v}(G/P))[z,z^{-1}]. \end{align*}$$
In particular, we have
 $$\begin{align*}u_{\mu,v}^*\mathcal{T}^{vert}\simeq \bigoplus_{\alpha\in -v(R^+\setminus R_P^+)}\mathcal{O}_{\mathbb{P}^1}(-\alpha(\mu)).\end{align*}$$
$$\begin{align*}u_{\mu,v}^*\mathcal{T}^{vert}\simeq \bigoplus_{\alpha\in -v(R^+\setminus R_P^+)}\mathcal{O}_{\mathbb{P}^1}(-\alpha(\mu)).\end{align*}$$
Proof. This follows from the explicit construction (4.1) of
![]() $\mathcal {E}_{t^{\mu }}(G/P)$
.
$\mathcal {E}_{t^{\mu }}(G/P)$
.
Recall the function c defined in Definition 3.4.
Lemma 4.2. For any
![]() $\mu \in Q^{\vee }$
and
$\mu \in Q^{\vee }$
and
![]() $v\in W^P$
, we have
$v\in W^P$
, we have
![]() $c([u_{\mu ,v}]) = v^{-1}(\mu ) + Q^{\vee }_P\in Q^{\vee }/Q^{\vee }_P$
.
$c([u_{\mu ,v}]) = v^{-1}(\mu ) + Q^{\vee }_P\in Q^{\vee }/Q^{\vee }_P$
.
Proof. Write
![]() $c([u_{\mu ,v}]) = \eta + Q^{\vee }_P$
. Let
$c([u_{\mu ,v}]) = \eta + Q^{\vee }_P$
. Let
![]() $\rho \in (Q^{\vee }/Q^{\vee }_P)^*$
. By definition,
$\rho \in (Q^{\vee }/Q^{\vee }_P)^*$
. By definition,
![]() $\rho (\eta )$
is the degree of the line bundle
$\rho (\eta )$
is the degree of the line bundle
![]() $u_{\mu ,v}^*\mathcal {L}_{\rho }$
. From the explicit construction (4.1) of
$u_{\mu ,v}^*\mathcal {L}_{\rho }$
. From the explicit construction (4.1) of
![]() $\mathcal {E}_{t^{\mu }}(G/P)$
and the definition of
$\mathcal {E}_{t^{\mu }}(G/P)$
and the definition of
![]() $\mathcal {L}_{\rho }$
, we see that
$\mathcal {L}_{\rho }$
, we see that
![]() $u_{\mu ,v}^*\mathcal {L}_{\rho }$
is defined by the transition matrix
$u_{\mu ,v}^*\mathcal {L}_{\rho }$
is defined by the transition matrix
![]() $-\rho (v^{-1}(\mu ))$
. It follows that the degree is equal to
$-\rho (v^{-1}(\mu ))$
. It follows that the degree is equal to
![]() $\rho (v^{-1}(\mu ))$
. Since
$\rho (v^{-1}(\mu ))$
. Since
![]() $\rho $
is arbitrary, the result follows.
$\rho $
is arbitrary, the result follows.
4.2 Regularity of the moduli
Recall the key reason for
![]() $\overline {\mathcal {M}}_{0,n}(G/P,\beta )$
to be regular is that
$\overline {\mathcal {M}}_{0,n}(G/P,\beta )$
to be regular is that
![]() $G/P$
is convex; that is,
$G/P$
is convex; that is,
for any morphism
![]() $u:C\rightarrow G/P$
, where C is a genus zero nodal curve. Surprisingly,
$u:C\rightarrow G/P$
, where C is a genus zero nodal curve. Surprisingly,
![]() $\mathcal {E}_{f_{\mathcal {G}r,wt_{\lambda }}}(G/P)$
also satisfies this property, provided the morphisms in question represent section classes. The goal of this subsection is to prove this fact. First, we show that it suffices to verify the analogue of (4.2) for a smaller class of u. In what follows, C always denotes a genus zero nodal curve.
$\mathcal {E}_{f_{\mathcal {G}r,wt_{\lambda }}}(G/P)$
also satisfies this property, provided the morphisms in question represent section classes. The goal of this subsection is to prove this fact. First, we show that it suffices to verify the analogue of (4.2) for a smaller class of u. In what follows, C always denotes a genus zero nodal curve.
Definition 4.3. Let X be a variety with a T-action. A morphism
![]() $u:C\rightarrow X$
is said to be T-invariant if for any
$u:C\rightarrow X$
is said to be T-invariant if for any
![]() $t\in T$
, there exists an automorphism
$t\in T$
, there exists an automorphism
![]() $\phi :C\rightarrow C$
such that
$\phi :C\rightarrow C$
such that
![]() $t\cdot u=u\circ \phi $
.
$t\cdot u=u\circ \phi $
.
Lemma 4.4. Let X be a smooth projective variety with a T-action and
![]() $\beta \in H_2(X)$
. Suppose for any T-invariant morphism
$\beta \in H_2(X)$
. Suppose for any T-invariant morphism
![]() $u:C\rightarrow X$
representing
$u:C\rightarrow X$
representing
![]() $\beta $
, we have
$\beta $
, we have
![]() $H^1(C;u^*\mathcal {T}_X)=0$
. Then the same is true for any morphism representing
$H^1(C;u^*\mathcal {T}_X)=0$
. Then the same is true for any morphism representing
![]() $\beta $
.
$\beta $
.
Proof. For a given morphism, choose
![]() $n\in \mathbb {Z}_{\geqslant 0}$
such that it becomes stable after adding n marked points to its domain. Let
$n\in \mathbb {Z}_{\geqslant 0}$
such that it becomes stable after adding n marked points to its domain. Let
![]() $\overline {M}:=\overline {M}_{0,n}(X,\beta )$
be the coarse moduli space of stable maps to X with n marked points and representing
$\overline {M}:=\overline {M}_{0,n}(X,\beta )$
be the coarse moduli space of stable maps to X with n marked points and representing
![]() $\beta $
. This space is constructed and proved to be projective in [Reference Fulton and Pandharipande8, Theorem 1]. Denote by V the set of
$\beta $
. This space is constructed and proved to be projective in [Reference Fulton and Pandharipande8, Theorem 1]. Denote by V the set of
![]() $[u]\in \overline {M}$
such that
$[u]\in \overline {M}$
such that
![]() $H^1(C;u^*\mathcal {T}_X)=0$
. We have to prove
$H^1(C;u^*\mathcal {T}_X)=0$
. We have to prove
![]() $\overline {M}=V$
. Notice that T preserves V, and hence, its complement
$\overline {M}=V$
. Notice that T preserves V, and hence, its complement
![]() $\overline {M}\setminus V$
. Let us assume for a while V is open so that
$\overline {M}\setminus V$
. Let us assume for a while V is open so that
![]() $\overline {M}\setminus V$
is closed. Suppose
$\overline {M}\setminus V$
is closed. Suppose
![]() $\overline {M}\setminus V\ne \emptyset $
. By Borel fixed-point theorem,
$\overline {M}\setminus V\ne \emptyset $
. By Borel fixed-point theorem,
![]() $\overline {M}\setminus V$
contains a T-fixed point
$\overline {M}\setminus V$
contains a T-fixed point
![]() $[u_0]$
. Then
$[u_0]$
. Then
![]() $u_0$
is T-invariant and
$u_0$
is T-invariant and
![]() $H^1(C_0;u_0^*\mathcal {T}_X)\ne 0$
, in contradiction to our assumption stated in the lemma. Therefore,
$H^1(C_0;u_0^*\mathcal {T}_X)\ne 0$
, in contradiction to our assumption stated in the lemma. Therefore,
![]() $\overline {M}=V$
, as desired.
$\overline {M}=V$
, as desired.
It remains to verify that V is open. Recall [Reference Fulton and Pandharipande8, Section 3 & 4]
![]() $\overline {M}$
is a union of open subschemes, each of which is a finite group quotient of the fine moduli U of stable maps to X with stable domains, representing
$\overline {M}$
is a union of open subschemes, each of which is a finite group quotient of the fine moduli U of stable maps to X with stable domains, representing
![]() $\beta $
and satisfying a condition depending on a fixed set of generic Cartier divisors on X. For each U, consider its universal family
$\beta $
and satisfying a condition depending on a fixed set of generic Cartier divisors on X. For each U, consider its universal family
![]() $\pi :\mathcal {C}\rightarrow U$
and evaluation map
$\pi :\mathcal {C}\rightarrow U$
and evaluation map
![]() $\operatorname {\mathrm {ev}}:\mathcal {C}\rightarrow X$
. Since
$\operatorname {\mathrm {ev}}:\mathcal {C}\rightarrow X$
. Since
![]() $\pi $
is flat and
$\pi $
is flat and
![]() $\operatorname {\mathrm {ev}}^*\mathcal {T}_X$
is locally free, the set
$\operatorname {\mathrm {ev}}^*\mathcal {T}_X$
is locally free, the set
![]() $U'$
of
$U'$
of
![]() $x\in U$
for which
$x\in U$
for which
![]() $H^1(\mathcal {C}_x;\operatorname {\mathrm {ev}}^*\mathcal {T}_X|_{\mathcal {C}_x})=0$
is open, by the semi-continuity theorem. Then
$H^1(\mathcal {C}_x;\operatorname {\mathrm {ev}}^*\mathcal {T}_X|_{\mathcal {C}_x})=0$
is open, by the semi-continuity theorem. Then
![]() $U'$
descends to an open subset
$U'$
descends to an open subset
![]() $U"$
of V. The proof is complete by varying U and taking the union of
$U"$
of V. The proof is complete by varying U and taking the union of
![]() $U"$
.
$U"$
.
Proposition 4.5. Let
![]() $\Gamma $
be a smooth projective variety and
$\Gamma $
be a smooth projective variety and
![]() $f:\Gamma \rightarrow \mathcal {G}r$
a morphism which is T-good (see Definition 2.3). Then for any morphism
$f:\Gamma \rightarrow \mathcal {G}r$
a morphism which is T-good (see Definition 2.3). Then for any morphism
![]() $u:C\rightarrow \mathcal {E}_{f}(G/P)$
which represents a section class of
$u:C\rightarrow \mathcal {E}_{f}(G/P)$
which represents a section class of
![]() $\mathcal {E}_{f}(G/P)$
, we have
$\mathcal {E}_{f}(G/P)$
, we have
![]() $H^1(C;u^*\mathcal {T}_{\mathcal {E}_{f}(G/P)})=0$
.
$H^1(C;u^*\mathcal {T}_{\mathcal {E}_{f}(G/P)})=0$
.
Proof. Since f is T-good,
![]() $\mathcal {E}_{f}(G/P)$
has a T-action by Lemma 3.5, and hence, by Lemma 4.4, we may assume u is T-invariant.
$\mathcal {E}_{f}(G/P)$
has a T-action by Lemma 3.5, and hence, by Lemma 4.4, we may assume u is T-invariant.
Consider the composition
Since u represents a section class, we have
![]() $(\operatorname {\mathrm {pr}}_{\Gamma }\circ \pi _f\circ u)_*[C]=0$
. But
$(\operatorname {\mathrm {pr}}_{\Gamma }\circ \pi _f\circ u)_*[C]=0$
. But
![]() $\Gamma $
is projective so
$\Gamma $
is projective so
![]() $\operatorname {\mathrm {pr}}_{\Gamma }\circ \pi _f\circ u$
is constant, and hence, there exists a factorization
$\operatorname {\mathrm {pr}}_{\Gamma }\circ \pi _f\circ u$
is constant, and hence, there exists a factorization
for some morphisms
![]() $\gamma :\operatorname {\mathrm {Spec}}\mathbb {C}\rightarrow \Gamma $
and
$\gamma :\operatorname {\mathrm {Spec}}\mathbb {C}\rightarrow \Gamma $
and
![]() $u':C\rightarrow \mathcal {E}_{f\circ \gamma }(G/P)$
where
$u':C\rightarrow \mathcal {E}_{f\circ \gamma }(G/P)$
where
![]() $\iota $
is the canonical inclusion.
$\iota $
is the canonical inclusion.
Consider next the composition
Since u represents a section class, we have
![]() $(\operatorname {\mathrm {pr}}_{\mathbb {P}^1}\circ \pi _{f\circ \gamma }\circ u')_*[C]=[\mathbb {P}^1]$
. It follows that we can write
$(\operatorname {\mathrm {pr}}_{\mathbb {P}^1}\circ \pi _{f\circ \gamma }\circ u')_*[C]=[\mathbb {P}^1]$
. It follows that we can write
![]() $C=C_0\cup C_1$
, where
$C=C_0\cup C_1$
, where
![]() $C_0\simeq \mathbb {P}^1$
is an irreducible component of C and
$C_0\simeq \mathbb {P}^1$
is an irreducible component of C and
![]() $C_1$
is the union of the other irreducible components, such that
$C_1$
is the union of the other irreducible components, such that
![]() $u'|_{C_0}$
is a section of
$u'|_{C_0}$
is a section of
![]() $\mathcal {E}_{f\circ \gamma }(G/P)$
after reparametrizing
$\mathcal {E}_{f\circ \gamma }(G/P)$
after reparametrizing
![]() $C_0$
and
$C_0$
and
![]() $u'|_{C_1}$
factors through a finite union of the fibers of
$u'|_{C_1}$
factors through a finite union of the fibers of
![]() $\pi _{f\circ \gamma }$
.
$\pi _{f\circ \gamma }$
.
Let us first deal with the case where
![]() $C_1$
is absent. In what follows, we will identify
$C_1$
is absent. In what follows, we will identify
![]() $C_0$
with
$C_0$
with
![]() $\mathbb {P}^1$
and assume
$\mathbb {P}^1$
and assume
![]() $u'=u'|_{C_0=\mathbb {P}^1}$
is a section of
$u'=u'|_{C_0=\mathbb {P}^1}$
is a section of
![]() $\mathcal {E}_{f\circ \gamma }(G/P)$
. Define
$\mathcal {E}_{f\circ \gamma }(G/P)$
. Define
![]() $\mathcal {F}:=u^*\mathcal {T}^{vert}_{\operatorname {\mathrm {pr}}_{\mathbb {P}^1}\circ \pi _f}$
, where
$\mathcal {F}:=u^*\mathcal {T}^{vert}_{\operatorname {\mathrm {pr}}_{\mathbb {P}^1}\circ \pi _f}$
, where
![]() $\mathcal {T}^{vert}_{\operatorname {\mathrm {pr}}_{\mathbb {P}^1}\circ \pi _f}$
is the vertical tangent bundle of the fiber bundle:
$\mathcal {T}^{vert}_{\operatorname {\mathrm {pr}}_{\mathbb {P}^1}\circ \pi _f}$
is the vertical tangent bundle of the fiber bundle:
Since
![]() $H^1(\mathbb {P}^1;\mathcal {T}_{\mathbb {P}^1})=0$
, it suffices to verify
$H^1(\mathbb {P}^1;\mathcal {T}_{\mathbb {P}^1})=0$
, it suffices to verify
![]() $H^1(\mathbb {P}^1;\mathcal {F})=0$
. Define
$H^1(\mathbb {P}^1;\mathcal {F})=0$
. Define
![]() $\mathcal {F}':= u^*\mathcal {T}^{vert}_{\pi _f}$
, where
$\mathcal {F}':= u^*\mathcal {T}^{vert}_{\pi _f}$
, where
![]() $\mathcal {T}^{vert}_{\pi _f}$
is the vertical tangent bundle of
$\mathcal {T}^{vert}_{\pi _f}$
is the vertical tangent bundle of
![]() $\pi _f$
. We have an exact sequence of coherent sheaves over
$\pi _f$
. We have an exact sequence of coherent sheaves over
![]() $C_0=\mathbb {P}^1$
:
$C_0=\mathbb {P}^1$
:
where the morphism
![]() $\mathcal {F}\rightarrow T_{\gamma }\Gamma \otimes _{\mathbb {C}}\mathcal {O}_{\mathbb {P}^1}$
is given by the projection. By looking at the associated long exact sequence, it suffices to show
$\mathcal {F}\rightarrow T_{\gamma }\Gamma \otimes _{\mathbb {C}}\mathcal {O}_{\mathbb {P}^1}$
is given by the projection. By looking at the associated long exact sequence, it suffices to show
Let us look at
![]() $\mathcal {F}'$
closely. Since u is T-invariant, we have
$\mathcal {F}'$
closely. Since u is T-invariant, we have
![]() $\gamma \in \Gamma ^T$
, and so
$\gamma \in \Gamma ^T$
, and so
![]() $f\circ \gamma =t^{\mu }$
for some
$f\circ \gamma =t^{\mu }$
for some
![]() $\mu \in Q^{\vee }$
. By the discussion in Section 4.1, we have
$\mu \in Q^{\vee }$
. By the discussion in Section 4.1, we have
![]() $u'=u_{\mu ,v}$
for some
$u'=u_{\mu ,v}$
for some
![]() $v\in W^P$
(after identifying
$v\in W^P$
(after identifying
![]() $\mathcal {E}_{f\circ \gamma }(G/P)$
with
$\mathcal {E}_{f\circ \gamma }(G/P)$
with
![]() $\mathcal {E}_{t^{\mu }}(G/P)$
). Put
$\mathcal {E}_{t^{\mu }}(G/P)$
). Put
![]() $R_v:=-v(R^+\setminus R^+_P)$
. Then by Lemma 4.1,
$R_v:=-v(R^+\setminus R^+_P)$
. Then by Lemma 4.1,
![]() $\mathcal {F}'$
is defined by the transition matrix
$\mathcal {F}'$
is defined by the transition matrix
and, in particular,
![]() $\mathcal {F}'\simeq \bigoplus _{\alpha \in R_v}\mathcal {O}_{\mathbb {P}^1}(-\alpha (\mu ))$
. (Recall we have identified
$\mathcal {F}'\simeq \bigoplus _{\alpha \in R_v}\mathcal {O}_{\mathbb {P}^1}(-\alpha (\mu ))$
. (Recall we have identified
![]() $T_{y_v}(G/P)$
with
$T_{y_v}(G/P)$
with
![]() $\bigoplus _{\alpha \in R_v}\mathfrak {g}_{\alpha }$
via the linearization of the G-action on
$\bigoplus _{\alpha \in R_v}\mathfrak {g}_{\alpha }$
via the linearization of the G-action on
![]() $G/P$
at
$G/P$
at
![]() $y_v$
.) Since for any
$y_v$
.) Since for any
![]() $m\in \mathbb {Z}$
$m\in \mathbb {Z}$
it follows that
Let us now look at
![]() $\mathcal {F}$
. By (4.3),
$\mathcal {F}$
. By (4.3),
![]() $\mathcal {F}$
is defined by a transition matrix of the form
$\mathcal {F}$
is defined by a transition matrix of the form
 $$\begin{align*}\begin{bmatrix} A(z)& B(z)\\ 0&\operatorname{\mathrm{id}} \end{bmatrix} \end{align*}$$
$$\begin{align*}\begin{bmatrix} A(z)& B(z)\\ 0&\operatorname{\mathrm{id}} \end{bmatrix} \end{align*}$$
for some
![]() $B(z)\in \operatorname {\mathrm {Hom}}(T_{\gamma }\Gamma ,T_{y_v}(G/P))[z,z^{-1}]$
. It follows that every element of
$B(z)\in \operatorname {\mathrm {Hom}}(T_{\gamma }\Gamma ,T_{y_v}(G/P))[z,z^{-1}]$
. It follows that every element of
![]() $H^0(\mathbb {P}^1;\mathcal {F})$
is given by a pair of polynomial maps
$H^0(\mathbb {P}^1;\mathcal {F})$
is given by a pair of polynomial maps
such that the Laurent polynomials
are polynomials in
![]() $z^{-1}$
. It is clear that
$z^{-1}$
. It is clear that
![]() $u_2(z)\equiv \zeta $
for some constant
$u_2(z)\equiv \zeta $
for some constant
![]() $\zeta \in T_{\gamma }\Gamma $
. Write
$\zeta \in T_{\gamma }\Gamma $
. Write
![]() $u_1(z)=\sum _{\alpha \in R_v} u_{1,\alpha }(z)$
, where
$u_1(z)=\sum _{\alpha \in R_v} u_{1,\alpha }(z)$
, where
![]() $u_{1,\alpha }:\mathbb {A}^1\rightarrow \mathfrak {g}_{\alpha }$
; and
$u_{1,\alpha }:\mathbb {A}^1\rightarrow \mathfrak {g}_{\alpha }$
; and
![]() $B(z)=\sum _{\alpha \in R_v}\sum _{k\in \mathbb {Z}}z^k B_{\alpha ,k}$
where
$B(z)=\sum _{\alpha \in R_v}\sum _{k\in \mathbb {Z}}z^k B_{\alpha ,k}$
where
![]() $B_{\alpha ,k}:T_{\gamma }\Gamma \rightarrow \mathfrak {g}_{\alpha }$
is linear. The above condition for
$B_{\alpha ,k}:T_{\gamma }\Gamma \rightarrow \mathfrak {g}_{\alpha }$
is linear. The above condition for
![]() $A(z)u_1(z)+B(z)u_2(z)$
is equivalent, given
$A(z)u_1(z)+B(z)u_2(z)$
is equivalent, given
![]() $u_2(z)\equiv \zeta $
, to the one that for any
$u_2(z)\equiv \zeta $
, to the one that for any
![]() $\alpha \in R_v$
, the Laurent polynomial
$\alpha \in R_v$
, the Laurent polynomial
is a polynomial in
![]() $z^{-1}$
. Since
$z^{-1}$
. Since
![]() $z^kB_{\alpha ,k}(\zeta )$
cannot cancel any term from
$z^kB_{\alpha ,k}(\zeta )$
cannot cancel any term from
![]() $z^{\alpha (\mu )}u_{1,\alpha }(z)$
for any k such that
$z^{\alpha (\mu )}u_{1,\alpha }(z)$
for any k such that
![]() $\alpha (\mu )>k$
, the above condition for (4.7) implies that for any
$\alpha (\mu )>k$
, the above condition for (4.7) implies that for any
![]() $\alpha \in R_v$
and
$\alpha \in R_v$
and
![]() $\alpha (\mu )>k>0$
, we have
$\alpha (\mu )>k>0$
, we have
![]() $B_{\alpha ,k}(\zeta )=0$
.
$B_{\alpha ,k}(\zeta )=0$
.
Define h to be the composition
 $$ \begin{align} T_{\gamma}\Gamma\xrightarrow{B(z)} T_{y_v}(G/P)[z,z^{-1}]\simeq \bigoplus_{\substack{\alpha\in R_v\\ k\in\mathbb{Z}}}z^k\mathfrak{g}_{\alpha}\rightarrow \bigoplus_{\substack{ \alpha\in R_v\\ \alpha(\mu)>k>0}}z^k\mathfrak{g}_{\alpha}, \end{align} $$
$$ \begin{align} T_{\gamma}\Gamma\xrightarrow{B(z)} T_{y_v}(G/P)[z,z^{-1}]\simeq \bigoplus_{\substack{\alpha\in R_v\\ k\in\mathbb{Z}}}z^k\mathfrak{g}_{\alpha}\rightarrow \bigoplus_{\substack{ \alpha\in R_v\\ \alpha(\mu)>k>0}}z^k\mathfrak{g}_{\alpha}, \end{align} $$
where the last arrow is the canonical projection. The discussion in the last paragraph implies that the composition
 $$ \begin{align} H^0(\mathbb{P}^1;\mathcal{F})\rightarrow T_{\gamma}\Gamma\xrightarrow{h} \bigoplus_{\substack{ \alpha\in R_v\\ \alpha(\mu)>k>0}}z^k\mathfrak{g}_{\alpha} \end{align} $$
$$ \begin{align} H^0(\mathbb{P}^1;\mathcal{F})\rightarrow T_{\gamma}\Gamma\xrightarrow{h} \bigoplus_{\substack{ \alpha\in R_v\\ \alpha(\mu)>k>0}}z^k\mathfrak{g}_{\alpha} \end{align} $$
is zero. By Lemma 4.6 below, which says that h is surjective, we have
But the LHS of (4.10) is equal to
![]() $\dim H^1(\mathbb {P}^1;\mathcal {F}')$
by (4.6). This gives inequality (4.4). Hence, the proof for the case where
$\dim H^1(\mathbb {P}^1;\mathcal {F}')$
by (4.6). This gives inequality (4.4). Hence, the proof for the case where
![]() $C_1$
is absent is complete.
$C_1$
is absent is complete.
Finally, we deal with the general case. By the normalization sequence (e.g., [Reference Cox and Katz5]), it suffices to show
-
1.
 $H^1(C_0; u^*\mathcal {T}_{\mathcal {E}_{f}(G/P)}|_{C_0})=H^1(C_1; u^*\mathcal {T}_{\mathcal {E}_{f}(G/P)}|_{C_1})=0$
; and
$H^1(C_0; u^*\mathcal {T}_{\mathcal {E}_{f}(G/P)}|_{C_0})=H^1(C_1; u^*\mathcal {T}_{\mathcal {E}_{f}(G/P)}|_{C_1})=0$
; and -
2. the evaluation map
 $H^0(C_1; u^*\mathcal {T}_{\mathcal {E}_{f}(G/P)}|_{C_1})\rightarrow \bigoplus _i T_{u(p_i)}\mathcal {E}_{f}(G/P)$
at the intersection points
$H^0(C_1; u^*\mathcal {T}_{\mathcal {E}_{f}(G/P)}|_{C_1})\rightarrow \bigoplus _i T_{u(p_i)}\mathcal {E}_{f}(G/P)$
at the intersection points
 $\{p_i\}$
of
$\{p_i\}$
of
 $C_0$
and
$C_0$
and
 $C_1$
is surjective.
$C_1$
is surjective.
We have proved
![]() $H^1(C_0; u^*\mathcal {T}_{\mathcal {E}_{f}(G/P)}|_{C_0})=0$
. Observe that
$H^1(C_0; u^*\mathcal {T}_{\mathcal {E}_{f}(G/P)}|_{C_0})=0$
. Observe that
![]() $u^*\mathcal {T}_{\mathcal {E}_{f}(G/P)}|_{C_1}$
is an extension of a trivial bundle by
$u^*\mathcal {T}_{\mathcal {E}_{f}(G/P)}|_{C_1}$
is an extension of a trivial bundle by
![]() $(u|_{C_1})^*\mathcal {T}^{vert}_{\pi _f}$
. The rest of the statements then follow from the well-known fact that
$(u|_{C_1})^*\mathcal {T}^{vert}_{\pi _f}$
. The rest of the statements then follow from the well-known fact that
![]() $\mathcal {T}_{G/P}$
is globally generated. The proof of Proposition 4.5 is complete.
$\mathcal {T}_{G/P}$
is globally generated. The proof of Proposition 4.5 is complete.
Lemma 4.6. The map h defined in (4.8) is surjective.
Proof. Let
![]() $\alpha \in R_v$
and
$\alpha \in R_v$
and
![]() $\alpha (\mu )>k>0$
. Pick a nonzero vector
$\alpha (\mu )>k>0$
. Pick a nonzero vector
![]() $X_{\alpha }\in \mathfrak {g}_{\alpha }$
. Define
$X_{\alpha }\in \mathfrak {g}_{\alpha }$
. Define
![]() $r_{\alpha ,k}:\mathbb {A}^1_s\rightarrow \Gamma $
by
$r_{\alpha ,k}:\mathbb {A}^1_s\rightarrow \Gamma $
by
![]() $s\mapsto \exp (sz^kX_{\alpha })\cdot \gamma $
where the action is the given
$s\mapsto \exp (sz^kX_{\alpha })\cdot \gamma $
where the action is the given
![]() $U_{\alpha ,k}$
-action on
$U_{\alpha ,k}$
-action on
![]() $\Gamma $
. The surjectivity of h follows if we can show that h sends
$\Gamma $
. The surjectivity of h follows if we can show that h sends
![]() $v:= D_{s=0}r_{\alpha ,k}(1)\in T_{\gamma }\Gamma $
to
$v:= D_{s=0}r_{\alpha ,k}(1)\in T_{\gamma }\Gamma $
to
![]() $z^kX_{\alpha }\in z^k\mathfrak {g}_{\alpha }$
.
$z^kX_{\alpha }\in z^k\mathfrak {g}_{\alpha }$
.
Consider the
![]() $G/P$
-bundle
$G/P$
-bundle
![]() $\mathcal {E}_{f\circ r_{\alpha ,k}}(G/P)$
over
$\mathcal {E}_{f\circ r_{\alpha ,k}}(G/P)$
over
![]() $\mathbb {P}^1\times \mathbb {A}^1_s$
. Notice that u naturally factors through a morphism
$\mathbb {P}^1\times \mathbb {A}^1_s$
. Notice that u naturally factors through a morphism
![]() $u":C_0=\mathbb {P}^1\rightarrow \mathcal {E}_{f\circ r_{\alpha ,k}}(G/P)$
. Since f is T-good and in particular
$u":C_0=\mathbb {P}^1\rightarrow \mathcal {E}_{f\circ r_{\alpha ,k}}(G/P)$
. Since f is T-good and in particular
![]() $U_{\alpha ,k}$
-equivariant,
$U_{\alpha ,k}$
-equivariant,
![]() $f\circ r_{\alpha ,k}$
is equal to the morphism
$f\circ r_{\alpha ,k}$
is equal to the morphism
![]() $s\mapsto \exp (sz^kX_{\alpha })\cdot t^{\mu }$
. By the definition of the
$s\mapsto \exp (sz^kX_{\alpha })\cdot t^{\mu }$
. By the definition of the
![]() $U_{\alpha ,k}$
-action on
$U_{\alpha ,k}$
-action on
![]() $\mathcal {G}r$
(see (2.2)), we have
$\mathcal {G}r$
(see (2.2)), we have
From this explicit construction, we see that the vector bundle
![]() $(u")^*\mathcal {T}^{vert}_{\operatorname {\mathrm {pr}}_{\mathbb {P}^1}\circ \pi _{f\circ r_{\alpha ,k}}}$
is defined by a transition matrix of the form
$(u")^*\mathcal {T}^{vert}_{\operatorname {\mathrm {pr}}_{\mathbb {P}^1}\circ \pi _{f\circ r_{\alpha ,k}}}$
is defined by a transition matrix of the form
 $$\begin{align*}\begin{bmatrix} A(z)& z^kX_{\alpha}\\ 0&\operatorname{\mathrm{id}} \end{bmatrix}, \end{align*}$$
$$\begin{align*}\begin{bmatrix} A(z)& z^kX_{\alpha}\\ 0&\operatorname{\mathrm{id}} \end{bmatrix}, \end{align*}$$
where
![]() $A(z)$
is the same as the one defined in (4.5). Since the transition matrix
$A(z)$
is the same as the one defined in (4.5). Since the transition matrix
 $$\begin{align*}\begin{bmatrix} A(z)& B(z)v\\ 0&\operatorname{\mathrm{id}} \end{bmatrix} \end{align*}$$
$$\begin{align*}\begin{bmatrix} A(z)& B(z)v\\ 0&\operatorname{\mathrm{id}} \end{bmatrix} \end{align*}$$
also defines the same vector bundle, these two matrices differ by a gauge transformation. A straightforward computation shows that the difference
![]() $B(z)v-z^kX_{\alpha }$
lies in the sum of
$B(z)v-z^kX_{\alpha }$
lies in the sum of
![]() $z^{k'}\mathfrak {g}_{\alpha '}$
with
$z^{k'}\mathfrak {g}_{\alpha '}$
with
![]() $\alpha '\in R_v$
and
$\alpha '\in R_v$
and
![]() $k'\leqslant 0$
or
$k'\leqslant 0$
or
![]() $k' \geqslant \alpha'(\mu)$
. Since
$k' \geqslant \alpha'(\mu)$
. Since
![]() $\alpha (\mu )>k>0$
, we have
$\alpha (\mu )>k>0$
, we have
![]() $h(v)=z^kX_{\alpha }$
, as desired.
$h(v)=z^kX_{\alpha }$
, as desired.
Let
![]() $wt_{\lambda }\in W_{af}^-$
. Recall
$wt_{\lambda }\in W_{af}^-$
. Recall
is the
![]() $B^-$
-good morphism fixed in Definition 2.5. Clearly, it is T-good. It follows that the condition in Proposition 4.5 is satisfied, and hence,
$B^-$
-good morphism fixed in Definition 2.5. Clearly, it is T-good. It follows that the condition in Proposition 4.5 is satisfied, and hence,
![]() $\overline {\mathcal {M}}(wt_{\lambda },\eta )$
is regular for any
$\overline {\mathcal {M}}(wt_{\lambda },\eta )$
is regular for any
![]() $\eta \in Q^{\vee }/Q^{\vee }_P$
. Moreover, since
$\eta \in Q^{\vee }/Q^{\vee }_P$
. Moreover, since
![]() $f_{\mathcal {G}r,wt_{\lambda }}$
is
$f_{\mathcal {G}r,wt_{\lambda }}$
is
![]() $B^-$
-equivariant, it follows that by Lemma 3.8,
$B^-$
-equivariant, it follows that by Lemma 3.8,
![]() $\overline {\mathcal {M}}(wt_{\lambda },\eta )$
has a
$\overline {\mathcal {M}}(wt_{\lambda },\eta )$
has a
![]() $B^-$
-action and
$B^-$
-action and
![]() $\operatorname {\mathrm {ev}}:\overline {\mathcal {M}}(wt_{\lambda },\eta )\rightarrow G/P$
is
$\operatorname {\mathrm {ev}}:\overline {\mathcal {M}}(wt_{\lambda },\eta )\rightarrow G/P$
is
![]() $B^-$
-equivariant.
$B^-$
-equivariant.
Now let
![]() $v\in W^P$
. Recall
$v\in W^P$
. Recall
is the
![]() $B^+$
-equivariant morphism fixed in Definition 2.2. By Lemma 2.1,
$B^+$
-equivariant morphism fixed in Definition 2.2. By Lemma 2.1,
![]() $f_{G/P,v}$
is transverse to
$f_{G/P,v}$
is transverse to
![]() $\operatorname {\mathrm {ev}}:\overline {\mathcal {M}}(wt_{\lambda },\eta )\rightarrow G/P$
(i.e., the sum of the images of the tangent maps of these morphisms is equal to the tangent space of the common target). It follows that the stack
$\operatorname {\mathrm {ev}}:\overline {\mathcal {M}}(wt_{\lambda },\eta )\rightarrow G/P$
(i.e., the sum of the images of the tangent maps of these morphisms is equal to the tangent space of the common target). It follows that the stack
is regular. Notice that there is still a T-action on
![]() $\overline {\mathcal {M}}(wt_{\lambda },v,\eta )$
, since
$\overline {\mathcal {M}}(wt_{\lambda },v,\eta )$
, since
![]() $T=B^-\cap B^+$
.
$T=B^-\cap B^+$
.
Lemma 4.7. Suppose
![]() $\overline {\mathcal {M}}(wt_{\lambda },v,\eta )\ne \emptyset $
. The dimension of
$\overline {\mathcal {M}}(wt_{\lambda },v,\eta )\ne \emptyset $
. The dimension of
![]() $\overline {\mathcal {M}}(wt_{\lambda },v,\eta )$
is equal to
$\overline {\mathcal {M}}(wt_{\lambda },v,\eta )$
is equal to
![]() $\ell (wt_{\lambda })+\ell (v)+\sum _{\alpha \in R^+\setminus R^+_P}\alpha (\eta )$
.
$\ell (wt_{\lambda })+\ell (v)+\sum _{\alpha \in R^+\setminus R^+_P}\alpha (\eta )$
.
Proof. By Lemma 3.7, the virtual dimension of
![]() $\overline {\mathcal {M}}(wt_{\lambda },\eta )$
is equal to
$\overline {\mathcal {M}}(wt_{\lambda },\eta )$
is equal to
![]() $\ell (wt_{\lambda })+\dim G/P +\sum _{\alpha \in R^+\setminus R^+_P}\alpha (\eta )$
. It follows that the virtual dimension of
$\ell (wt_{\lambda })+\dim G/P +\sum _{\alpha \in R^+\setminus R^+_P}\alpha (\eta )$
. It follows that the virtual dimension of
![]() $\overline {\mathcal {M}}(wt_{\lambda },v,\eta )$
is equal to
$\overline {\mathcal {M}}(wt_{\lambda },v,\eta )$
is equal to
 $$ \begin{align*} & \left( \ell(wt_{\lambda})+\dim G/P +\sum_{\alpha\in R^+\setminus R^+_P}\alpha(\eta)\right) + \ell(v) - \dim G/P \\ =&~ \ell(wt_{\lambda})+\ell(v)+\sum_{\alpha\in R^+\setminus R^+_P}\alpha(\eta). \end{align*} $$
$$ \begin{align*} & \left( \ell(wt_{\lambda})+\dim G/P +\sum_{\alpha\in R^+\setminus R^+_P}\alpha(\eta)\right) + \ell(v) - \dim G/P \\ =&~ \ell(wt_{\lambda})+\ell(v)+\sum_{\alpha\in R^+\setminus R^+_P}\alpha(\eta). \end{align*} $$
Since
![]() $\overline {\mathcal {M}}(wt_{\lambda },v,\eta )$
is regular, its dimension is equal to its virtual dimension. The proof is complete.
$\overline {\mathcal {M}}(wt_{\lambda },v,\eta )$
is regular, its dimension is equal to its virtual dimension. The proof is complete.
4.3 Zero-dimensional components
Let
![]() $wt_{\lambda }\in W_{af}^-$
,
$wt_{\lambda }\in W_{af}^-$
,
![]() $v\in W^P$
and
$v\in W^P$
and
![]() $\eta \in Q^{\vee }/Q^{\vee }_P$
. Put
$\eta \in Q^{\vee }/Q^{\vee }_P$
. Put
![]() $\overline {\mathcal {M}}:=\overline {\mathcal {M}}(wt_{\lambda },v,\eta )$
, the stack defined at the end of Section 4.2.
$\overline {\mathcal {M}}:=\overline {\mathcal {M}}(wt_{\lambda },v,\eta )$
, the stack defined at the end of Section 4.2.
Proposition 4.8. The stack
![]() $\overline {\mathcal {M}}$
is nonempty and zero-dimensional if and only if
$\overline {\mathcal {M}}$
is nonempty and zero-dimensional if and only if
![]() $v\in wW_P$
,
$v\in wW_P$
,
![]() $\eta =\lambda +Q^{\vee }_P$
and the following set of conditions, which we denote by
$\eta =\lambda +Q^{\vee }_P$
and the following set of conditions, which we denote by
![]() $C(wt_{\lambda })$
, holds:
$C(wt_{\lambda })$
, holds:
 $$\begin{align*}\left\{ \begin{array}{rcl} \alpha\in (-w R_P^+)\cap R^+ &\Longrightarrow& \alpha(w(\lambda))=1\\ \alpha\in (-w R_P^+)\cap (-R^+) &\Longrightarrow& \alpha(w(\lambda))=0 \end{array} \right. .\end{align*}$$
$$\begin{align*}\left\{ \begin{array}{rcl} \alpha\in (-w R_P^+)\cap R^+ &\Longrightarrow& \alpha(w(\lambda))=1\\ \alpha\in (-w R_P^+)\cap (-R^+) &\Longrightarrow& \alpha(w(\lambda))=0 \end{array} \right. .\end{align*}$$
In this case,
![]() $\overline {\mathcal {M}}$
is a one-point stack with trivial stabilizer.
$\overline {\mathcal {M}}$
is a one-point stack with trivial stabilizer.
Proof. Suppose
![]() $\overline {\mathcal {M}}\ne \emptyset $
and
$\overline {\mathcal {M}}\ne \emptyset $
and
![]() $\dim \overline {\mathcal {M}}=0$
. Notice that the boundary of
$\dim \overline {\mathcal {M}}=0$
. Notice that the boundary of
![]() $\overline {\mathcal {M}}$
is stratified by the moduli spaces of stable maps satisfying the same conditions as those imposed on points of
$\overline {\mathcal {M}}$
is stratified by the moduli spaces of stable maps satisfying the same conditions as those imposed on points of
![]() $\overline {\mathcal {M}}$
, plus the condition that their domain curves are reducible and have fixed combinatorial types. Arguing as before, we conclude that these strata are smooth and of expected dimension. Since
$\overline {\mathcal {M}}$
, plus the condition that their domain curves are reducible and have fixed combinatorial types. Arguing as before, we conclude that these strata are smooth and of expected dimension. Since
![]() $\dim \overline {\mathcal {M}}=0$
, they are empty, and hence, every point of
$\dim \overline {\mathcal {M}}=0$
, they are empty, and hence, every point of
![]() $\overline {\mathcal {M}}$
is represented by a stable map u to
$\overline {\mathcal {M}}$
is represented by a stable map u to
![]() $\mathcal {E}_{f_{\mathcal {G}r,wt_{\lambda }}}(G/P)$
which factors through a section
$\mathcal {E}_{f_{\mathcal {G}r,wt_{\lambda }}}(G/P)$
which factors through a section
![]() $u'$
of
$u'$
of
![]() $\mathcal {E}_{f_{\mathcal {G}r,wt_{\lambda }}\circ \gamma }(G/P)$
for some
$\mathcal {E}_{f_{\mathcal {G}r,wt_{\lambda }}\circ \gamma }(G/P)$
for some
![]() $\gamma :\operatorname {\mathrm {Spec}}\mathbb {C}\rightarrow \Gamma _{wt_{\lambda }}$
. This section is necessarily T-invariant because
$\gamma :\operatorname {\mathrm {Spec}}\mathbb {C}\rightarrow \Gamma _{wt_{\lambda }}$
. This section is necessarily T-invariant because
![]() $\overline {\mathcal {M}}$
is zero-dimensional and has a T-action. It follows that
$\overline {\mathcal {M}}$
is zero-dimensional and has a T-action. It follows that
![]() $\gamma \in \Gamma _{wt_{\lambda }}^T$
, and hence,
$\gamma \in \Gamma _{wt_{\lambda }}^T$
, and hence,
![]() $f_{\mathcal {G}r,wt_{\lambda }}\circ \gamma =t^{\mu _{\gamma }}$
for some
$f_{\mathcal {G}r,wt_{\lambda }}\circ \gamma =t^{\mu _{\gamma }}$
for some
![]() $\mu _{\gamma }\in Q^{\vee }$
. Thus, we have
$\mu _{\gamma }\in Q^{\vee }$
. Thus, we have
![]() $u'=u_{\mu _{\gamma },v'}$
for some
$u'=u_{\mu _{\gamma },v'}$
for some
![]() $v'\in W^P$
, after identifying
$v'\in W^P$
, after identifying
![]() $\mathcal {E}_{f_{\mathcal {G}r,wt_{\lambda }}\circ \gamma }(G/P)$
with
$\mathcal {E}_{f_{\mathcal {G}r,wt_{\lambda }}\circ \gamma }(G/P)$
with
![]() $\mathcal {E}_{t^{\mu }}(G/P)$
.
$\mathcal {E}_{t^{\mu }}(G/P)$
.
Let us show
![]() $\mu _{\gamma }=w(\lambda )$
. Let
$\mu _{\gamma }=w(\lambda )$
. Let
![]() $w't_{\lambda '}\in W_{af}^-$
be the unique element such that
$w't_{\lambda '}\in W_{af}^-$
be the unique element such that
![]() $\mu _{\gamma }=w'(\lambda ')$
. Since
$\mu _{\gamma }=w'(\lambda ')$
. Since
![]() $t^{\mu _{\gamma }}\in \overline {\mathcal {B}\cdot t^{w(\lambda )}}$
, we have
$t^{\mu _{\gamma }}\in \overline {\mathcal {B}\cdot t^{w(\lambda )}}$
, we have
![]() $\ell (w't_{\lambda '})\leqslant \ell (wt_{\lambda })$
, and the equality holds if and only if
$\ell (w't_{\lambda '})\leqslant \ell (wt_{\lambda })$
, and the equality holds if and only if
![]() $wt_{\lambda }=w't_{\lambda '}$
. Observe that the section
$wt_{\lambda }=w't_{\lambda '}$
. Observe that the section
![]() $u_{\mu _{\gamma },v'}$
also represents a point of
$u_{\mu _{\gamma },v'}$
also represents a point of
![]() $\overline {\mathcal {M}}':=\overline {\mathcal {M}}(w't_{\lambda '},v,\eta )$
. It follows that
$\overline {\mathcal {M}}':=\overline {\mathcal {M}}(w't_{\lambda '},v,\eta )$
. It follows that
![]() $\overline {\mathcal {M}}'\ne \emptyset $
, and hence, by the regularity, we have
$\overline {\mathcal {M}}'\ne \emptyset $
, and hence, by the regularity, we have
![]() $\dim \overline {\mathcal {M}}'\geqslant 0$
. But by Lemma 4.7,
$\dim \overline {\mathcal {M}}'\geqslant 0$
. But by Lemma 4.7,
 $$\begin{align*}0=\dim\overline{\mathcal{M}}=\ell(wt_{\lambda})+\ell(v)+ \sum_{\alpha\in R^+\setminus R^+_P}\alpha(\eta) \geqslant \ell(w't_{\lambda'})+\ell(v)+ \sum_{\alpha\in R^+\setminus R^+_P}\alpha(\eta)=\dim\overline{\mathcal{M}}'\geqslant 0. \end{align*}$$
$$\begin{align*}0=\dim\overline{\mathcal{M}}=\ell(wt_{\lambda})+\ell(v)+ \sum_{\alpha\in R^+\setminus R^+_P}\alpha(\eta) \geqslant \ell(w't_{\lambda'})+\ell(v)+ \sum_{\alpha\in R^+\setminus R^+_P}\alpha(\eta)=\dim\overline{\mathcal{M}}'\geqslant 0. \end{align*}$$
It follows that
![]() $\ell (wt_{\lambda })=\ell (w't_{\lambda '})$
, and hence,
$\ell (wt_{\lambda })=\ell (w't_{\lambda '})$
, and hence,
![]() $wt_{\lambda }=w't_{\lambda '}$
as desired.
$wt_{\lambda }=w't_{\lambda '}$
as desired.
By a similar argument, we have
![]() $v'=v$
.
$v'=v$
.
To finish the proof, we need the following explicit formulae for the terms
![]() $\ell (wt_{\lambda })$
,
$\ell (wt_{\lambda })$
,
![]() $\ell (v)$
and
$\ell (v)$
and
![]() $\sum _{\alpha \in R^+\setminus R^+_P}\alpha (\eta )=\langle [\mathbb {P}^1], c_1(u_{w(\lambda ),v}^*\mathcal {T}^{vert})\rangle $
, where
$\sum _{\alpha \in R^+\setminus R^+_P}\alpha (\eta )=\langle [\mathbb {P}^1], c_1(u_{w(\lambda ),v}^*\mathcal {T}^{vert})\rangle $
, where
![]() $\mathcal {T}^{vert}$
is the vertical tangent bundle of the fiber bundle
$\mathcal {T}^{vert}$
is the vertical tangent bundle of the fiber bundle
![]() $\mathcal {E}_{t^{w(\lambda )}}(G/P)\rightarrow \mathbb {P}^1$
. To formulate them, pick a regular dominant element
$\mathcal {E}_{t^{w(\lambda )}}(G/P)\rightarrow \mathbb {P}^1$
. To formulate them, pick a regular dominant element
![]() $a\in \mathfrak {t}_{\mathbb {R}}:=Q^{\vee }\otimes _{\mathbb {Z}}\mathbb {R}$
which is sufficiently close to the origin and a dominant element
$a\in \mathfrak {t}_{\mathbb {R}}:=Q^{\vee }\otimes _{\mathbb {Z}}\mathbb {R}$
which is sufficiently close to the origin and a dominant element
![]() $b\in \mathfrak {t}_{\mathbb {R}}$
which determines the parabolic type of P (i.e.,
$b\in \mathfrak {t}_{\mathbb {R}}$
which determines the parabolic type of P (i.e.,
![]() $\alpha _i(b)=0$
if
$\alpha _i(b)=0$
if
![]() $\alpha _i\in R^+_P$
and
$\alpha _i\in R^+_P$
and
![]() $\alpha _i(b)>0$
otherwise). We have
$\alpha _i(b)>0$
otherwise). We have
 $$ \begin{align} \ell(wt_{\lambda}) &= \sum_{\alpha(w(\lambda)-a)>0}\lfloor \alpha(w(\lambda)-a)\rfloor\\ \ell(v) &= -\sum_{\alpha(v\cdot b)<0}\lfloor \alpha(-a)\rfloor \nonumber\\ \langle [\mathbb{P}^1],c_1(u_{w(\lambda),v}^*\mathcal{T}^{vert})\rangle &= -\sum_{\alpha(v\cdot b)<0} \alpha(w(\lambda)) \nonumber, \end{align} $$
$$ \begin{align} \ell(wt_{\lambda}) &= \sum_{\alpha(w(\lambda)-a)>0}\lfloor \alpha(w(\lambda)-a)\rfloor\\ \ell(v) &= -\sum_{\alpha(v\cdot b)<0}\lfloor \alpha(-a)\rfloor \nonumber\\ \langle [\mathbb{P}^1],c_1(u_{w(\lambda),v}^*\mathcal{T}^{vert})\rangle &= -\sum_{\alpha(v\cdot b)<0} \alpha(w(\lambda)) \nonumber, \end{align} $$
where the summations are taken over
![]() $\alpha \in R$
satisfying the stated conditions. The first formula will be proved below, the second is obvious, and the last follows from Lemma 4.1. Summing up these equations and using the assumption
$\alpha \in R$
satisfying the stated conditions. The first formula will be proved below, the second is obvious, and the last follows from Lemma 4.1. Summing up these equations and using the assumption
![]() $\dim \overline {\mathcal {M}}=0$
, we obtain
$\dim \overline {\mathcal {M}}=0$
, we obtain
The last equation can be written as
where
 $$\begin{align*}A(\alpha,v):= \left\{ \begin{array}{cc} -1& \alpha(v\cdot b)>0\\ 0& \alpha(v\cdot b)=0\\ 1& \alpha(v\cdot b)<0 \end{array} \right. \quad\text{ and }\quad B(\alpha,v) :=\left\{ \begin{array}{cc} 0& \alpha(v\cdot b)\leqslant 0\\ 1& \alpha(v\cdot b)>0 \end{array} \right. .\end{align*}$$
$$\begin{align*}A(\alpha,v):= \left\{ \begin{array}{cc} -1& \alpha(v\cdot b)>0\\ 0& \alpha(v\cdot b)=0\\ 1& \alpha(v\cdot b)<0 \end{array} \right. \quad\text{ and }\quad B(\alpha,v) :=\left\{ \begin{array}{cc} 0& \alpha(v\cdot b)\leqslant 0\\ 1& \alpha(v\cdot b)>0 \end{array} \right. .\end{align*}$$
Observe that each of the summands of the LHS of (4.12) is non-negative. It follows that they are all equal to 0. This holds precisely when the following conditions are satisfied:
 $$\begin{align*}\left\{ \begin{array}{rcl} \alpha\in v(R^+\setminus R_P^+) &\Longrightarrow& \alpha\in wR^+\\ \alpha\in vR_P \cap (-wR^+)\cap R^+ &\Longrightarrow& \alpha(w(\lambda))=1\\ \alpha\in vR_P \cap (-wR^+)\cap (-R^+) &\Longrightarrow& \alpha(w(\lambda))=0 \end{array} \right. .\end{align*}$$
$$\begin{align*}\left\{ \begin{array}{rcl} \alpha\in v(R^+\setminus R_P^+) &\Longrightarrow& \alpha\in wR^+\\ \alpha\in vR_P \cap (-wR^+)\cap R^+ &\Longrightarrow& \alpha(w(\lambda))=1\\ \alpha\in vR_P \cap (-wR^+)\cap (-R^+) &\Longrightarrow& \alpha(w(\lambda))=0 \end{array} \right. .\end{align*}$$
Here, we have used the assumption
![]() $wt_{\lambda }\in W_{af}^-$
, which implies
$wt_{\lambda }\in W_{af}^-$
, which implies
![]() $-w(\lambda )+a\in w\mathring {\Lambda }$
, where
$-w(\lambda )+a\in w\mathring {\Lambda }$
, where
![]() $\mathring {\Lambda }$
is the interior of the dominant chamber. Notice that the first condition is equivalent to
$\mathring {\Lambda }$
is the interior of the dominant chamber. Notice that the first condition is equivalent to
![]() $v\in wW_P$
, and the conjunction of the other two is equivalent, given the first condition, to
$v\in wW_P$
, and the conjunction of the other two is equivalent, given the first condition, to
![]() $C(wt_{\lambda })$
, since
$C(wt_{\lambda })$
, since
![]() $vR_P\cap (-wR^+)=-wR_P^+$
if
$vR_P\cap (-wR^+)=-wR_P^+$
if
![]() $v\in wW_P$
. By Lemma 4.2 and the fact that every element of
$v\in wW_P$
. By Lemma 4.2 and the fact that every element of
![]() $W_P$
descends to the identity in the quotient
$W_P$
descends to the identity in the quotient
![]() $Q^{\vee }/Q^{\vee }_P$
, we have
$Q^{\vee }/Q^{\vee }_P$
, we have
![]() $\eta =c([u_{w(\lambda ),v}])=v^{-1}w(\lambda )+Q^{\vee }_P=\lambda +Q^{\vee }_P$
. This proves one direction of Proposition 4.8. The other direction is clear from the above discussion.
$\eta =c([u_{w(\lambda ),v}])=v^{-1}w(\lambda )+Q^{\vee }_P=\lambda +Q^{\vee }_P$
. This proves one direction of Proposition 4.8. The other direction is clear from the above discussion.
The last assertion follows from the above discussion and the fact that
Proof of formula (4.11)
Denote by
![]() $\Delta _0$
the dominant alcove. Since
$\Delta _0$
the dominant alcove. Since
![]() $wt_{\lambda }$
is a minimal length coset representative, the line segment joining
$wt_{\lambda }$
is a minimal length coset representative, the line segment joining
![]() $w(\lambda )$
and a intersects the interior of
$w(\lambda )$
and a intersects the interior of
![]() $wt_{\lambda }(\Delta _0)$
. Therefore,
$wt_{\lambda }(\Delta _0)$
. Therefore,
![]() $\ell (wt_{\lambda })$
is equal to the number of affine walls intersecting the interior of this line segment which is easily seen to be the RHS of (4.11).
$\ell (wt_{\lambda })$
is equal to the number of affine walls intersecting the interior of this line segment which is easily seen to be the RHS of (4.11).
4.4 Final step
Following [Reference Lam and Shimozono16, Lemma 10.2], we define
![]() $(W^P)_{af}$
to be the set of
$(W^P)_{af}$
to be the set of
![]() $wt_{\lambda }\in W_{af}$
such that
$wt_{\lambda }\in W_{af}$
such that
 $$ \begin{align} \left\{ \begin{array}{rcl} \alpha\in R_P^+\cap (-w^{-1}R^+) &\Longrightarrow& \alpha(\lambda)=-1\\ \alpha\in R_P^+\cap w^{-1}R^+&\Longrightarrow& \alpha(\lambda)=0 \end{array} \right.. \end{align} $$
$$ \begin{align} \left\{ \begin{array}{rcl} \alpha\in R_P^+\cap (-w^{-1}R^+) &\Longrightarrow& \alpha(\lambda)=-1\\ \alpha\in R_P^+\cap w^{-1}R^+&\Longrightarrow& \alpha(\lambda)=0 \end{array} \right.. \end{align} $$
Theorem 4.9. The
![]() $H_T^{\bullet }(\operatorname {\mathrm {pt}})$
-algebra homomorphism
$H_T^{\bullet }(\operatorname {\mathrm {pt}})$
-algebra homomorphism
![]() $\Phi _{SS}$
defined in Definition 3.10 satisfies
$\Phi _{SS}$
defined in Definition 3.10 satisfies
 $$\begin{align*}\Phi_{SS}(\xi_{wt_{\lambda}})=\left\{ \begin{array}{cc} q^{\lambda+Q^{\vee}_P}\sigma_{\widetilde{w}}& wt_{\lambda}\in (W^P)_{af}\\ 0& \text{otherwise} \end{array} \right. \end{align*}$$
$$\begin{align*}\Phi_{SS}(\xi_{wt_{\lambda}})=\left\{ \begin{array}{cc} q^{\lambda+Q^{\vee}_P}\sigma_{\widetilde{w}}& wt_{\lambda}\in (W^P)_{af}\\ 0& \text{otherwise} \end{array} \right. \end{align*}$$
for any
![]() $wt_{\lambda }\in W_{af}^-$
, where
$wt_{\lambda }\in W_{af}^-$
, where
![]() $\widetilde {w}\in W^P$
is the minimal length representative of the coset
$\widetilde {w}\in W^P$
is the minimal length representative of the coset
![]() $wW_P$
.
$wW_P$
.
Proof. Write
![]() $\Phi _{SS}(\xi _{wt_{\lambda }})=\sum _{v\in W^P}\sum _{\eta \in Q^{\vee }/Q^{\vee }_P}q^{\eta } c_{\eta ,v} \sigma _v$
. Since
$\Phi _{SS}(\xi _{wt_{\lambda }})=\sum _{v\in W^P}\sum _{\eta \in Q^{\vee }/Q^{\vee }_P}q^{\eta } c_{\eta ,v} \sigma _v$
. Since
![]() $\overline {\mathcal {M}}(wt_{\lambda },v,\eta )$
is regular and
$\overline {\mathcal {M}}(wt_{\lambda },v,\eta )$
is regular and
![]() $f_{G/P,v}$
is the composition of a T-equivariant resolution
$f_{G/P,v}$
is the composition of a T-equivariant resolution
![]() $\Gamma _v\rightarrow \overline {B^+\cdot y_v}$
and the inclusion
$\Gamma _v\rightarrow \overline {B^+\cdot y_v}$
and the inclusion
![]() $\overline {B^+\cdot y_v}\hookrightarrow G/P$
, we have
$\overline {B^+\cdot y_v}\hookrightarrow G/P$
, we have
 $$\begin{align*}c_{\eta,v}=\int_{\overline{\mathcal{M}}(wt_{\lambda},v,\eta)} 1 \in H_T^{\bullet}(\operatorname{\mathrm{pt}}),\end{align*}$$
$$\begin{align*}c_{\eta,v}=\int_{\overline{\mathcal{M}}(wt_{\lambda},v,\eta)} 1 \in H_T^{\bullet}(\operatorname{\mathrm{pt}}),\end{align*}$$
which is zero unless
![]() $\overline {\mathcal {M}}(wt_{\lambda },v,\eta )$
is nonempty and zero-dimensional. By Proposition 4.8, the last condition is equivalent to
$\overline {\mathcal {M}}(wt_{\lambda },v,\eta )$
is nonempty and zero-dimensional. By Proposition 4.8, the last condition is equivalent to
![]() $v\in wW_P$
,
$v\in wW_P$
,
![]() $\eta =\lambda +Q^{\vee }_P$
and the condition
$\eta =\lambda +Q^{\vee }_P$
and the condition
![]() $C(wt_{\lambda })$
, and in this case,
$C(wt_{\lambda })$
, and in this case,
![]() $c_{\eta ,v}=1$
. It remains to show that
$c_{\eta ,v}=1$
. It remains to show that
![]() $C(wt_{\lambda })$
is equivalent to the condition
$C(wt_{\lambda })$
is equivalent to the condition
![]() $wt_{\lambda }\in (W^P)_{af}$
. This is proved by replacing
$wt_{\lambda }\in (W^P)_{af}$
. This is proved by replacing
![]() $\alpha $
in (4.13) with
$\alpha $
in (4.13) with
![]() $-w^{-1}\alpha $
.
$-w^{-1}\alpha $
.
Acknowledgements
The first version of this paper, which already contains all key ideas, was written when the author was a PhD student at the Chinese University of Hong Kong. He would like to thank the referees for useful comments which help to improve the exposition substantially.
Competing interest
The authors have no competing interests to declare.