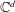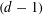Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Sheffer, Adam
Szabó, Endre
and
Zahl, Joshua
2018.
Point-Curve Incidences in the Complex Plane.
Combinatorica,
Vol. 38,
Issue. 2,
p.
487.