Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Louis, Anand
2015.
Hypergraph Markov Operators, Eigenvalues and Approximation Algorithms.
p.
713.
LENZ, JOHN
and
MUBAYI, DHRUV
2016.
Perfect Packings in Quasirandom Hypergraphs II.
Combinatorics, Probability and Computing,
Vol. 25,
Issue. 4,
p.
595.
Lenz, John
and
Mubayi, Dhruv
2016.
Perfect packings in quasirandom hypergraphs I.
Journal of Combinatorial Theory, Series B,
Vol. 119,
Issue. ,
p.
155.
Lenz, John
Mubayi, Dhruv
and
Mycroft, Richard
2016.
Hamilton cycles in quasirandom hypergraphs.
Random Structures & Algorithms,
Vol. 49,
Issue. 2,
p.
363.
Aigner-Horev, Elad
Conlon, David
Hàn, Hiệp
Person, Yury
and
Schacht, Mathias
2017.
Quasirandomness in hypergraphs.
Electronic Notes in Discrete Mathematics,
Vol. 61,
Issue. ,
p.
13.
Aigner-Horev, Elad
and
Hàn, Hiệp
2017.
Linear quasi-randomness of subsets of abelian groups and hypergraphs.
Electronic Notes in Discrete Mathematics,
Vol. 61,
Issue. ,
p.
21.
Towsner, Henry
2017.
σ‐algebras for quasirandom hypergraphs.
Random Structures & Algorithms,
Vol. 50,
Issue. 1,
p.
114.
REIHER, CHRISTIAN
and
SCHACHT, MATHIAS
2019.
FORCING QUASIRANDOMNESS WITH TRIANGLES.
Forum of Mathematics, Sigma,
Vol. 7,
Issue. ,
Aigner-Horev, Elad
and
Hàn, Hiệp
2020.
Linear quasi-randomness of subsets of abelian groups and hypergraphs.
European Journal of Combinatorics,
Vol. 88,
Issue. ,
p.
103116.
Mubayi, Dhruv
and
Suk, Andrew
2020.
Discrete Mathematics and Applications.
Vol. 165,
Issue. ,
p.
405.
Cooper, Joshua
2020.
Adjacency spectra of random and complete hypergraphs.
Linear Algebra and its Applications,
Vol. 596,
Issue. ,
p.
184.
Zhou, Zhixin
and
Zhu, Yizhe
2021.
Sparse random tensors: Concentration, regularization and applications.
Electronic Journal of Statistics,
Vol. 15,
Issue. 1,
Harris, Kameron Decker
and
Zhu, Yizhe
2021.
Deterministic Tensor Completion with Hypergraph Expanders.
SIAM Journal on Mathematics of Data Science,
Vol. 3,
Issue. 4,
p.
1117.
Aigner-Horev, Elad
and
Levy, Gil
2021.
Tight Hamilton cycles in cherry-quasirandom 3-uniform hypergraphs.
Combinatorics, Probability and Computing,
Vol. 30,
Issue. 3,
p.
412.
Mubayi, Dhruv
and
Razborov, Alexander
2021.
Polynomial to exponential transition in Ramsey theory.
Proceedings of the London Mathematical Society,
Vol. 122,
Issue. 1,
p.
69.
Ding, Laihao
Han, Jie
Sun, Shumin
Wang, Guanghui
and
Zhou, Wenling
2022.
F$F$‐factors in Quasi‐random Hypergraphs.
Journal of the London Mathematical Society,
Vol. 106,
Issue. 3,
p.
1810.
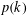 $p(k)$ denote the partition function of
$p(k)$ denote the partition function of 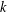 $k$. For each
$k$. For each 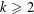 $k\geqslant 2$, we describe a list of
$k\geqslant 2$, we describe a list of  $p(k)-1$ quasirandom properties that a
$p(k)-1$ quasirandom properties that a  $k$-uniform hypergraph can have. Our work connects previous notions on linear hypergraph quasirandomness by Kohayakawa, Rödl, and Skokan, and by Conlon, Hàn, Person, and Schacht, and the spectral approach of Friedman and Wigderson. For each of the quasirandom properties that is described, we define the largest and the second largest eigenvalues. We show that a hypergraph satisfies these quasirandom properties if and only if it has a large spectral gap. This answers a question of Conlon, Hàn, Person, and Schacht. Our work can be viewed as a partial extension to hypergraphs of the seminal spectral results of Chung, Graham, and Wilson for graphs.
$k$-uniform hypergraph can have. Our work connects previous notions on linear hypergraph quasirandomness by Kohayakawa, Rödl, and Skokan, and by Conlon, Hàn, Person, and Schacht, and the spectral approach of Friedman and Wigderson. For each of the quasirandom properties that is described, we define the largest and the second largest eigenvalues. We show that a hypergraph satisfies these quasirandom properties if and only if it has a large spectral gap. This answers a question of Conlon, Hàn, Person, and Schacht. Our work can be viewed as a partial extension to hypergraphs of the seminal spectral results of Chung, Graham, and Wilson for graphs.