Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-28T08:31:20.741Z Has data issue: false hasContentIssue false
  • English
  • Français

THE DIMENSION OF A SUBCATEGORY OF MODULES

Part of: Theory of modules and ideals Abelian categories Representation theory of rings and algebras

Published online by Cambridge University Press:  09 October 2015

HAILONG DAO  and
RYO TAKAHASHI
HAILONG DAO
Affiliation:
Department of Mathematics, University of Kansas, Lawrence, KS 66045-7523, USA; [email protected]
RYO TAKAHASHI
Affiliation:
Graduate School of Mathematics, Nagoya University, Furocho, Chikusaku, Nagoya 464-8602, Japan Department of Mathematics, University of Nebraska, Lincoln, NE 68588-0130, USA; [email protected]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let 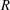$R$ be a commutative noetherian local ring. As an analog of the notion of the dimension of a triangulated category defined by Rouquier, the notion of the dimension of a subcategory of finitely generated 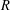$R$-modules is introduced in this paper. We found evidence that certain categories over nice singularities have small dimensions. When 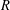$R$ is Cohen–Macaulay, under a mild assumption it is proved that finiteness of the dimension of the full subcategory consisting of maximal Cohen–Macaulay modules which are locally free on the punctured spectrum is equivalent to saying that 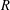$R$ is an isolated singularity. As an application, the celebrated theorem of Auslander, Huneke, Leuschke, and Wiegand is not only recovered but also improved. The dimensions of stable categories of maximal Cohen–Macaulay modules as triangulated categories are also investigated in the case where 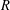$R$ is Gorenstein, and special cases of the recent results of Aihara and Takahashi, and Oppermann and Št́ovíček are recovered and improved. Our key technique involves a careful study of annihilators and supports of 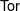$\mathsf{Tor}$, 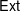$\mathsf{Ext}$, and $\underline{\mathsf{Hom}}$ between two subcategories.

MSC classification

Primary: 13C60: Module categories
Secondary: 13C14: Cohen-Macaulay modules 16G60: Representation type (finite, tame, wild, etc.) 18E30: Derived categories, triangulated categories
Type
Research Article
Information
Forum of Mathematics, Sigma , Volume 3 , 2015 , e19
Check for updates
Creative Commons
Creative Common License - CCCreative Common License - BY
This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
Copyright
© The Author(s) 2015

References

Aihara, T. and Takahashi, R., ‘Generators and dimensions of derived categories of modules’, Comm. Algebra 43(11) (2015), 5003–5029.Google Scholar
Auslander, M., ‘Isolated singularities and existence of almost split sequences’, inRepresentation Theory, II (Ottawa, Ont., 1984), Lecture Notes in Mathematics, 1178 (Springer, Berlin, 1986), 194–242.Google Scholar
Auslander, M. and Bridger, M., ‘Stable module theory’, inMemoirs of the American Mathematical Society 94 (American Mathematical Society, Providence, RI, 1969), 146.Google Scholar
Auslander, M. and Reiten, I., ‘Applications of contravariantly finite subcategories’, Adv. Math. 86(1) (1991), 111–152.Google Scholar
Avramov, L. L., ‘Infinite free resolutions’, inSix Lectures on Commutative Algebra (Bellaterra, 1996), Progress in Mathematics, 166 (Birkhäuser, Basel, 1998), 1–118.Google Scholar
Avramov, L. L., Buchweitz, R.-O., Iyengar, S. B. and Miller, C., ‘Homology of perfect complexes’, Adv. Math. 223(5) (2010), 1731–1781.Google Scholar
Benson, D. J., Carlson, J. F. and Rickard, J., ‘Thick subcategories of the stable module category’, Fund. Math. 153(1) (1997), 59–80.Google Scholar
Benson, D., Iyengar, S. B. and Krause, H., ‘Stratifying modular representations of finite groups’, Ann. of Math. (2) 174(3) (2011), 1643–1684.Google Scholar
Bondal, A. and van den Bergh, M., ‘Generators and representability of functors in commutative and noncommutative geometry’, Mosc. Math. J. 3(1) (2003), 1–36. 258.Google Scholar
Buchweitz, R.-O., Maximal Cohen–Macaulay modules and Tate-cohomology over Gorenstein rings, Unpublished Paper 1986, http://hdl.handle.net/1807/16682.Google Scholar
Buchweitz, R.-O., Greuel, G.-M. and Schreyer, F.-O., ‘Cohen–Macaulay modules on hypersurface singularities, II’, Invent. Math. 88(1) (1987), 165–182.Google Scholar
Dao, H. and Takahashi, R., ‘The radius of a subcategory of modules’, Algebra Number Theory 8(1) (2014), 141–172.Google Scholar
Dao, H. and Takahashi, R., ‘Classification of resolving subcategories and grade consistent functions’, Int. Math. Res. Not. IMRN (1) (2015), 119–149.Google Scholar
Dao, H. and Takahashi, R., ‘Upper bounds for dimensions of singularity categories’, C. R. Math. Acad. Sci. Paris 353(4) (2015), 297–301.Google Scholar
Dao, H. and Veliche, O., ‘Comparing complexities of pairs of modules’, J. Algebra 322(9) (2009), 3047–3062.Google Scholar
Devinatz, E. S., Hopkins, M. J. and Smith, J. H., ‘Nilpotence and stable homotopy theory, I’, Ann. of Math. (2) 128(2) (1988), 207–241.Google Scholar
Friedlander, E. M. and Pevtsova, J., ‘Π-supports for modules for finite group schemes’, Duke Math. J. 139(2) (2007), 317–368.CrossRefGoogle Scholar
Happel, D., Triangulated Categories in the Representation Theory of Finite-dimensional Algebras, London Mathematical Society Lecture Note Series, 119 (Cambridge University Press, Cambridge, 1988).Google Scholar
Hopkins, M. J., ‘Global methods in homotopy theory’, inHomotopy Theory (Durham, 1985), London Mathematical Society Lecture Note Series, 117 (Cambridge University Press, Cambridge, 1987), 73–96.Google Scholar
Hopkins, M. J. and Smith, J. H., ‘Nilpotence and stable homotopy theory, II’, Ann. of Math. (2) 148(1) (1998), 1–49.Google Scholar
Huneke, C. and Leuschke, G. J., ‘Two theorems about maximal Cohen–Macaulay modules’, Math. Ann. 324(2) (2002), 391–404.Google Scholar
Iyama, O. and Wemyss, M., ‘The classification of special Cohen–Macaulay modules’, Math. Z. 265(1) (2010), 41–83.Google Scholar
Leuschke, G. and Wiegand, R., ‘Ascent of finite Cohen–Macaulay type’, J. Algebra 2 (2000), 674–681.Google Scholar
Lipman, J., ‘Rational singularities with applications to algebraic surfaces and unique factorization’, Publ. Math. Inst. Hautes Études Sci. 36 (1969), 195–279.Google Scholar
Neeman, A., ‘The chromatic tower for D (R)’, Topology 31(3) (1992), 519–532: with an appendix by Marcel Bökstedt.CrossRefGoogle Scholar
Oppermann, S. and Št́ovíček, J., ‘Generating the bounded derived category and perfect ghosts’, Bull. Lond. Math. Soc. 44(2) (2012), 285–298.Google Scholar
Rouquier, R., ‘Dimensions of triangulated categories’, J. K-Theory 1 (2008), 193–256.Google Scholar
Takahashi, R., ‘Modules in resolving subcategories which are free on the punctured spectrum’, Pacific J. Math. 241(2) (2009), 347–367.Google Scholar
Takahashi, R., ‘Classifying thick subcategories of the stable category of Cohen–Macaulay modules’, Adv. Math. 225(4) (2010), 2076–2116.CrossRefGoogle Scholar
Takahashi, R., ‘Contravariantly finite resolving subcategories over commutative rings’, Amer. J. Math. 133(2) (2011), 417–436.Google Scholar
Takahashi, R., ‘Classifying resolving subcategories over a Cohen–Macaulay local ring’, Math. Z. 273(1–2) (2013), 569–587.Google Scholar
Thomason, R. W., ‘The classification of triangulated subcategories’, Compos. Math. 105(1) (1997), 1–27.Google Scholar
Verdier, J.-L., ‘Catégories dérivées, état 0’, inSGA 4[[()[]mml:mfrac[]()]][[()[]mml:mrow []()]]1[[()[]/mml:mrow[]()]] [[()[]mml:mrow []()]]2[[()[]/mml:mrow[]()]][[()[]/mml:mfrac[]()]], Lecture Notes in Mathematics, 569 (Springer, New York, 1977), 262–308.Google Scholar
Wang, H.-J., ‘On the Fitting ideals in free resolutions’, Michigan Math. J. 41(3) (1994), 587–608.Google Scholar
Wunram, J., ‘Reflexive modules on quotient surface singularities’, Math. Ann. 279(4) (1988), 583–598.Google Scholar
Yoshino, Y., ‘A functorial approach to modules of G-dimension zero’, Illinois J. Math. 49(2) (2005), 345–367.CrossRefGoogle Scholar