Hostname: page-component-586b7cd67f-dlnhk Total loading time: 0 Render date: 2024-11-23T05:37:05.780Z Has data issue: false hasContentIssue false
  • English
  • Français

COARSE AND FINE GEOMETRY OF THE THURSTON METRIC

Part of: Riemann surfaces Low-dimensional topology

Published online by Cambridge University Press:  26 May 2020

DAVID DUMAS Open the ORCID record for DAVID DUMAS [Opens in a new window] ,
ANNA LENZHEN ,
KASRA RAFI Open the ORCID record for KASRA RAFI [Opens in a new window]  and
JING TAO
DAVID DUMAS
Affiliation:
Department of Mathematics, Statistics, and Computer Science, University of Illinois at Chicago, Chicago, IL, USA; [email protected]
ANNA LENZHEN
Affiliation:
Department of Mathematics, University of Rennes, Rennes, France; [email protected]
KASRA RAFI
Affiliation:
Department of Mathematics, University of Toronto, Toronto, Canada; [email protected]
JING TAO
Affiliation:
Department of Mathematics, University of Oklahoma, Norman, OK, USA; [email protected]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We study the geometry of the Thurston metric on the Teichmüller space of hyperbolic structures on a surface 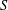$S$. Some of our results on the coarse geometry of this metric apply to arbitrary surfaces 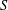$S$ of finite type; however, we focus particular attention on the case where the surface is a once-punctured torus. In that case, our results provide a detailed picture of the infinitesimal, local, and global behavior of the geodesics of the Thurston metric, as well as an analogue of Royden’s theorem.

MSC classification

Primary: 57M50: Geometric structures on low-dimensional manifolds
Secondary: 30F60: Teichmüller theory
Type
Differential Geometry and Geometric Analysis
Information
Forum of Mathematics, Sigma , Volume 8 , 2020 , e28
Creative Commons
Creative Common License - CCCreative Common License - BY
This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
Copyright
© The Author(s) 2020

References

Bonahon, F., ‘Bouts des variétés hyperboliques de dimension 3’, Ann. of Math. (2) 124(1) (1986), 71–158.CrossRefGoogle Scholar
Bonahon, F., ‘Shearing hyperbolic surfaces, bending pleated surfaces and Thurston’s symplectic form’, Ann. Fac. Sci. Toulouse Math. (6) 5(2) (1996), 233–297.CrossRefGoogle Scholar
Bonahon, F., ‘Variations of the boundary geometry of 3-dimensional hyperbolic convex cores’, J. Differential Geom. 50(1) (1998), 1–24.CrossRefGoogle Scholar
Bonahon, F. and Zhu, X., ‘The metric space of geodesic laminations on a surface. II. Small surfaces’, inProceedings of the Casson Fest, Geometry & Topology Monographs, 7 (Geometry & Topology Publications, Coventry, 2004), 509–547.Google Scholar
Choi, Y.-E. and Rafi, K., ‘Comparison between Teichmüller and Lipschitz metrics’, J. Lond. Math. Soc. (2) 76(3) (2007), 739–756.CrossRefGoogle Scholar
Choi, Y.-E., Rafi, K. and Series, C., ‘Lines of minima and Teichmüller geodesics’, Geom. Funct. Anal. 18(3) (2008), 698–754.CrossRefGoogle Scholar
Danciger, J., Guéritaud, F. and Kassel, F., ‘Geometry and topology of complete Lorentz spacetimes of constant curvature’, Ann. Sci. Éc. Norm. Supér. (4) 49(1) (2016), 1–56.CrossRefGoogle Scholar
Dumas, D., ‘CP1: complex projective structures toolkit’, 2013. https://github.com/daviddumas/cp1.Google Scholar
Farb, B. and Margalit, D., A Primer on Mapping Class Groups, Princeton Mathematical Series, 49 (Princeton University Press, Princeton, NJ, 2012).Google Scholar
Gardiner, F. P., ‘Infinitesimal bending and twisting in one-dimensional dynamics’, Trans. Amer. Math. Soc. 347(3) (1995), 915–937.CrossRefGoogle Scholar
Guéritaud, F., ‘Lengthening deformations of singular hyperbolic tori’, Ann. Fac. Sci. Toulouse 24(5) (2015), 1239–1260.CrossRefGoogle Scholar
Guéritaud, F., Personal communication, 2016.Google Scholar
Guéritaud, F. and Kassel, F., ‘Maximally stretched laminations on geometrically finite hyperbolic manifolds’, Geom. Topol. 21(2) (2017), 693–840.CrossRefGoogle Scholar
Ivanov, N. V., ‘Automorphism of complexes of curves and of Teichmüller spaces’, Int. Math. Res. Not. IMRN 14 (1997), 651–666.CrossRefGoogle Scholar
Kassel, F., ‘Quotients compacts d’espaces homogènes réels ou $p$-adiques’, PhD Thesis, Université Paris-Sud 11, 2009.Google Scholar
Keen, L., ‘On fundamental domains and the Teichmüller modular group’, inContributions to Analysis (A Collection of Papers Dedicated to Lipman Bers) (Academic Press, New York, 1974), 185–194.Google Scholar
Lenzhen, A., Rafi, K. and Tao, J., ‘Bounded combinatorics and the Lipschitz metric on Teichmüller space’, Geom. Dedicata 159 (2012), 353–371.CrossRefGoogle Scholar
Lenzhen, A., Rafi, K. and Tao, J., ‘The shadow of a Thurston geodesic to the curve graph’, J. Topol. 8(4) (2015), 1085–1118.CrossRefGoogle Scholar
Masur, H. A. and Minsky, Y. N., ‘Geometry of the complex of curves. II. Hierarchical structure’, Geom. Funct. Anal. 10(4) (2000), 902–974.CrossRefGoogle Scholar
Matveev, V. S. and Troyanov, M., ‘The Myers–Steenrod theorem for Finsler manifolds of low regularity’, Proc. Amer. Math. Soc. 145(6) (2017), 2699–2712.CrossRefGoogle Scholar
Minsky, Y. N., ‘Extremal length estimates and product regions in Teichmüller space’, Duke Math. J. 83(2) (1996), 249–286.CrossRefGoogle Scholar
Minsky, Y. N., ‘The classification of punctured-torus groups’, Ann. of Math. (2) 149(2) (1999), 559–626.CrossRefGoogle Scholar
Papadopoulos, A., ‘On Thurston’s boundary of Teichmüller space and the extension of earthquakes’, Topology Appl. 41(3) (1991), 147–177.CrossRefGoogle Scholar
Papadopoulos, A. and Théret, G., ‘On Teichmüller’s metric and Thurston’s asymmetric metric on Teichmüller space’, inHandbook of Teichmüller Theory, Vol. I, IRMA Lectures in Mathematics and Theoretical Physics, 11 (European Mathematical Society, Zürich, 2007), 111–204.CrossRefGoogle Scholar
Rafi, K., ‘A characterization of short curves of a Teichmüller geodesic’, Geom. Topol. 9 (2005), 179–202.CrossRefGoogle Scholar
Rafi, K., ‘Hyperbolicity in Teichmüller space’, Geom. Topol. 18(5) (2014), 3025–3053.CrossRefGoogle Scholar
Royden, H. L., ‘Automorphisms and isometries of Teichmüller space’, inAdvances in the Theory of Riemann Surfaces (Proc. Conf., Stony Brook, NY, 1969), Annals of Mathematical Studies, 66 (Princeton University Press, Princeton, NJ, 1971), 369–383.CrossRefGoogle Scholar
Théret, G., ‘On the negative convergence of Thurston’s stretch lines towards the boundary of Teichmüller space’, Ann. Acad. Sci. Fenn. Math. 32(2) (2007), 381–408.Google Scholar
Thurston, W. P., ‘Geometry and topology of 3-manifolds’. Princeton University Lecture Notes, 1986. Available at www.msri.org/publications/books/gt3m.Google Scholar
Thurston, W. P., ‘Hyperbolic structures on 3-manifolds, II: surface groups and 3-manifolds which fiber over the circle’, Preprint, 1986, arXiv:math/9801045.Google Scholar
Thurston, W. P., ‘Minimal stretch maps between hyperbolic surfaces’, Preprint, 1986, arXiv:math.GT/9801039.Google Scholar
Walsh, C., ‘The horoboundary and isometry group of Thurston’s Lipschitz metric’, inHandbook of Teichmüller Theory, Vol. IV, IRMA Lectures in Mathematics and Theoretical Physics, 19 (European Mathematical Society, Zürich, 2014), 327–353.Google Scholar