Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-26T09:14:46.007Z Has data issue: false hasContentIssue false
  • English
  • Français

Bounds for sets with no polynomial progressions

Part of: Sequences and sets

Published online by Cambridge University Press:  05 January 2021

Sarah Peluse
Sarah Peluse*
Affiliation:
School of Mathematics, Institute for Advanced Study, 1 Einstein Drive, Princeton, NJ08540, USA; E-mail: [email protected]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

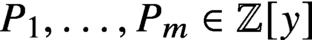Let $P_1,\dots ,P_m\in \mathbb{Z} [y]$ be polynomials with distinct degrees, each having zero constant term. We show that any subset A of $\{1,\dots ,N\}$ with no nontrivial progressions of the form $x,x+P_1(y),\dots ,x+P_m(y)$ has size $|A|\ll N/(\log \log {N})^{c_{P_1,\dots ,P_m}}$. Along the way, we prove a general result controlling weighted counts of polynomial progressions by Gowers norms.

MSC classification

Primary: 11B30: Arithmetic combinatorics; higher degree uniformity
Type
Number Theory
Information
Forum of Mathematics, Pi , Volume 8 , 2020 , e16
Check for updates
Creative Commons
Creative Common License - CCCreative Common License - BY
This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
Copyright
© The Author(s), 2020. Published by Cambridge University Press

References

Balog, A., Pelikán, J., Pintz, J. and Szemerédi, E., ‘Difference sets without $\kappa$th powers’, Acta Math. Hungar. 65(2) (1994), 165–187.10.1007/BF01874311CrossRefGoogle Scholar
Bergelson, V. and Leibman, A., ‘Polynomial extensions of van der Waerden’s and Szemerédi’s theorems’, J. Amer. Math. Soc. 9(3) (1996), 725–753.10.1090/S0894-0347-96-00194-4CrossRefGoogle Scholar
Bloom, T. F., ‘A quantitative improvement for Roth’s theorem on arithmetic progressions’, J. Lond. Math. Soc. (2) 93(3) (2016), 643–663.10.1112/jlms/jdw010CrossRefGoogle Scholar
Bourgain, J. and Chang, M.-C., ‘Nonlinear Roth type theorems in finite fields’, Israel J. Math., 221 (2017), 853–867.10.1007/s11856-017-1577-9CrossRefGoogle Scholar
Dong, D., Li, X. and Sawin, W., ‘Improved estimates for polynomial Roth type theorems in finite fields’, Preprint, 2017, arXiv:1709.00080.Google Scholar
Gowers, W. T., ‘A new proof of Szemerédi’s theorem for arithmetic progressions of length four’, Geom. Funct. Anal. 8(3) (1998), 529–551.10.1007/s000390050065CrossRefGoogle Scholar
Gowers, W. T., ‘A new proof of Szemerédi’s theorem’, Geom. Funct. Anal. 11(3) (2001), 465–588.10.1007/s00039-001-0332-9CrossRefGoogle Scholar
Gowers, W. T., ‘Arithmetic progressions in sparse sets’, in Current Developments in Mathematics, 2000, pp. 149–196 (International Press, Somerville, MA, 2001).Google Scholar
Green, B. and Tao, T., ‘Linear equations in primes’, Ann. of Math. (2), 171(3) (2010), 1753–1850.10.4007/annals.2010.171.1753CrossRefGoogle Scholar
Green, B. and Tao, T., ‘New bounds for Szemerédi’s theorem, III: a polylogarithmic bound for ${r}_4(N)$’, Mathematika 63(3) (2017), 944–1040.10.1112/S0025579317000316CrossRefGoogle Scholar
Lucier, J., ‘Intersective sets given by a polynomial’, Acta Arith. 123(1) (2006), 57–95.10.4064/aa123-1-4CrossRefGoogle Scholar
Montgomery, H. L., Ten Lectures on the Interface between Analytic Number Theory and Harmonic Analysis, Vol. 84 of CBMS Regional Conference Series in Mathematics (American Mathematical Society, Providence, RI, 1994).Google Scholar
Peluse, S., ‘Three-term polynomial progressions in subsets of finite fields’, Israel J. Math. 228(1) (2018), 379–405.10.1007/s11856-018-1768-zCrossRefGoogle Scholar
Peluse, S., ‘On the polynomial Szemerédi theorem in finite fields’, Duke Math. J. 168(5) (2019), 749–774.10.1215/00127094-2018-0051CrossRefGoogle Scholar
Peluse, S. and Prendiville, S., ‘Quantitative bounds in the non-linear Roth theorem’, Preprint, 2019, arXiv:1903.02592.Google Scholar
Prendiville, S., ‘Quantitative bounds in the polynomial Szemerédi theorem: the homogeneous case’, Discrete Anal. 5 (2017), 34 pages.Google Scholar
Rice, A., ‘A maximal extension of the best-known bounds for the Furstenberg-Sárközy theorem’, Acta Arith. 187(1) (2019), 1–41.10.4064/aa170828-26-8CrossRefGoogle Scholar
Sárközy, A., ‘On difference sets of sequences of integers. I’, Acta Math. Acad. Sci. Hungar. 31(1–2) (1978), 125–149.10.1007/BF01896079CrossRefGoogle Scholar
Sárközy, A., ‘On difference sets of sequences of integers. III’, Acta Math. Acad. Sci. Hungar. 31 (1978), 355–386.10.1007/BF01901984CrossRefGoogle Scholar
Slijepčević, S., ‘A polynomial Sárközy-Furstenberg theorem with upper bounds’, Acta Math. Hungar. 98(1–2) (2003), 111–128.10.1023/A:1022813623110CrossRefGoogle Scholar
Szemerédi, E., ‘On sets of integers containing no $k$elements in arithmetic progression’, Acta Arith. 27 (1975), 199–245. Collection of articles in memory of Juriĭ Vladimirovič Linnik.10.4064/aa-27-1-199-245CrossRefGoogle Scholar
Tao, T., Higher Order Fourier Analysis, Vol. 142 of Graduate Studies in Mathematics (American Mathematical Society, Providence, RI, 2012).Google Scholar
Tao, T. and Ziegler, T., ‘The primes contain arbitrarily long polynomial progressions’, Acta Math. 201(2) (2008), 213–305.10.1007/s11511-008-0032-5CrossRefGoogle Scholar
Tao, T. and Ziegler, T., ‘Concatenation theorems for anti-Gowers-uniform functions and Host-Kra characteristic factors’, Discrete Anal. 60 (2016), 61 pages.Google Scholar
Tao, T. and Ziegler, T., ‘Polynomial patterns in the primes’, Forum Math. Pi 6 (2018), e1, 60 pages.10.1017/fmp.2017.3CrossRefGoogle Scholar