3. Results and Discussion

Different modes of droplet manipulation are possible with our microfluidic device. The standard mode is the reciprocating motion of droplets between the side channels, that can be used to introduce reagents into a droplet from one side channel and subtract reaction products from the opposing channel (Reference Shojaeian and HardtShojaeian & Hardt, 2020). By switching off the electric field, the droplet can be fixed at a position between the side channels, while the exchange with the reservoirs is suspended. Most notably, the partial coalescence mode that was previously observed for much larger droplets (Reference Hamlin, Creasey and RistenpartHamlin et al., 2012) also occurs for our micron-scale droplets. More specifically, for a salt concentration inside the droplet below a threshold value (in our case approx. 0.17 mM), a droplet may bounce back without volume loss for large enough values of the electric field strength, or it may experience a volume loss (partial coalescence) for smaller electric fields. This is demonstrated in Figure 2a. In this panel, and also in the other panels of this figure, the region of interest is rotated by 90° relative to Figure 1. Figure 2a shows snapshots from subsequent cycles of a droplet reciprocating between the two side channels, where the direction of motion is indicated by arrows. Since the electric field lines mainly extend between the two menisci, the field strength at the right meniscus is higher than that at the left meniscus. As a result, the conditions at the right meniscus are such that a droplet bounces off from that meniscus, while it undergoes partial coalescence at the left meniscus. This process is visible in the supplementary movies.

Figure 2. (a) Droplet reciprocating between the two menisci, undergoing partial coalescence at the left meniscus. The salt concentration is 0.17 mM and a voltage of 200 V was applied between the menisci. (b) Snapshots of consecutive cycles, showing the same droplet before getting in contact with the left meniscus. (c) A droplet with a diameter of approximately 800 nm that has been formed by partial coalescence and travels from left to right. The numbers indicate the time in milliseconds, and the red circle encloses the droplet. The parameters in panels b and c are the same as in panel a.

Partial coalescence gives the opportunity to reduce the diameter of droplets to the submicron scale. Figure 2b shows subsequent cycles from a reciprocating droplet motion shortly before undergoing partial coalescence with the left meniscus. The diameters of the droplets shown in the successive frames are 8.3, 6.9, 5.1, 3.4 and 1.6 μm. Our experiments do not give any indication that the cascade of droplet volume reduction comes to an end. However, due to the resolution limit of the optics, from a certain point on the droplets can no longer be imaged faithfully, but rather appear as a tiny grey patch. This is exemplified in Figure 2c. The figure shows a droplet experiencing a dramatic volume loss after partial coalescence with the left meniscus. The first frame shows the droplet before partial coalescence with the left meniscus. The daughter droplet, marked by the red circle, travels from left to right and has a diameter of approximately 800 nm. To estimate the size of such small droplets, a Gaussian function was fitted to the grey-scale values of the pixels around the position of a droplet. The full width at half-maximum was taken as an estimate for the droplet diameter. The smallest droplets that could be identified based on this method have a diameter of approximately 400 nm. However, the submicron diameters reported here should be taken with a grain of salt, since the Abbé resolution limit of the employed imaging system is approximately 600 nm. This means that the apparent droplet diameters are expected to be larger than the true diameters, since diffraction tends to broaden the grey-scale distributions. We speculate that the cascade of partial coalescence events continues for even smaller droplets, but these could not be detected using the available imaging system.

One key question is what determines the size of the daughter droplet for a given size of the parent droplet. When fixing the size of the parent droplet and the voltage between the side channels, there is still some fluctuation in the size of the daughter droplet. There are a few reports on the factors influencing the partial electrocoalescence of charged droplets with an oil–water interface, albeit for much larger droplets than considered in this work. (Reference Hamlin, Creasey and RistenpartHamlin et al. 2012) performed experiments from which they deduced that

(3.1)\begin{equation}\frac{a}{{{a_0}}} = K{\left( {\frac{{qE}}{{\gamma {a_0}}}} \right)^\alpha },\end{equation}

where q is the charge of the daughter droplet, E is the electric field strength, γ is the interfacial tension, a is the radius of the daughter droplet and a ₀ that of the parent droplet. For the scaling exponent they obtained $\alpha = 1/2$ and for the pre-factor $K = 0.85$. This is contrasted by the experiments reported by (Reference Anand, Juvekar and ThaokarAnand et al. 2020), who determined a scaling exponent $\alpha = 3/2$.

To study which factors influence the size of the daughter droplet, we have performed a number of experiments with varying parent droplet size, salt concentration and applied voltage. In previous studies only the charge of the daughter droplet was considered, but it is not a priori clear that the charge of the parent droplet should not play an equally important role in the partial coalescence process. Therefore, we have determined the droplet charge before and after partial coalescence. Details are given in the supplementary material. Figure 3 shows the effects of the electric field and salt concentration on the daughter droplet size for droplets with the initial sizes of 7 and 11 μm. The ratio of daughter and parent droplet sizes is plotted as a function of the square root of the electric Bond number ${(qE/\gamma {a_0})^{1/2}}$. This parameter is largely analogous to the parameter used in (Reference Hamlin, Creasey and RistenpartHamlin et al. 2012), with the difference that, in our case, q is the arithmetic average of the daughter and parent droplet charges; E denotes the electric field, which, however, only enters the equations in form of the product qE. This product was determined based on the procedure described in the supplementary material. Figure 3 indicates a similarity relationship, i.e. to a good approximation the experimental data depend linearly on the square root of the electric Bond number. In total, the scaling relationship is given by

(3.2)\begin{equation}\frac{a}{{{a_0}}} = 0.978{\left( {\frac{{qE}}{{\gamma {a_0}}}} \right)^{1/2}}\theta \left[ {{{\left( {\frac{{qE}}{{\gamma {a_0}}}} \right)}^{1/2}} - 0.515} \right],\end{equation}

where θ is the Heaviside function and the numerical factors were determined based on a least-squares fit. A major difference to the results obtained for much larger droplets by Hamlin et al. lies in the fact that only above a critical value of the scaling parameter can partial coalescence occur. Translated to the charge of the droplet, this means that a minimum charge is required for partial coalescence, below that only full coalescence will occur. Equation (2) also allows us to check the results obtained for the size of droplets that are so small that they only appear as faint spots (cf. Figure 2c). From the velocity of these spots the droplet charge can be determined. The prediction of the scaling relationship is in good agreement with the Gaussian fits to the grey-scale distribution.

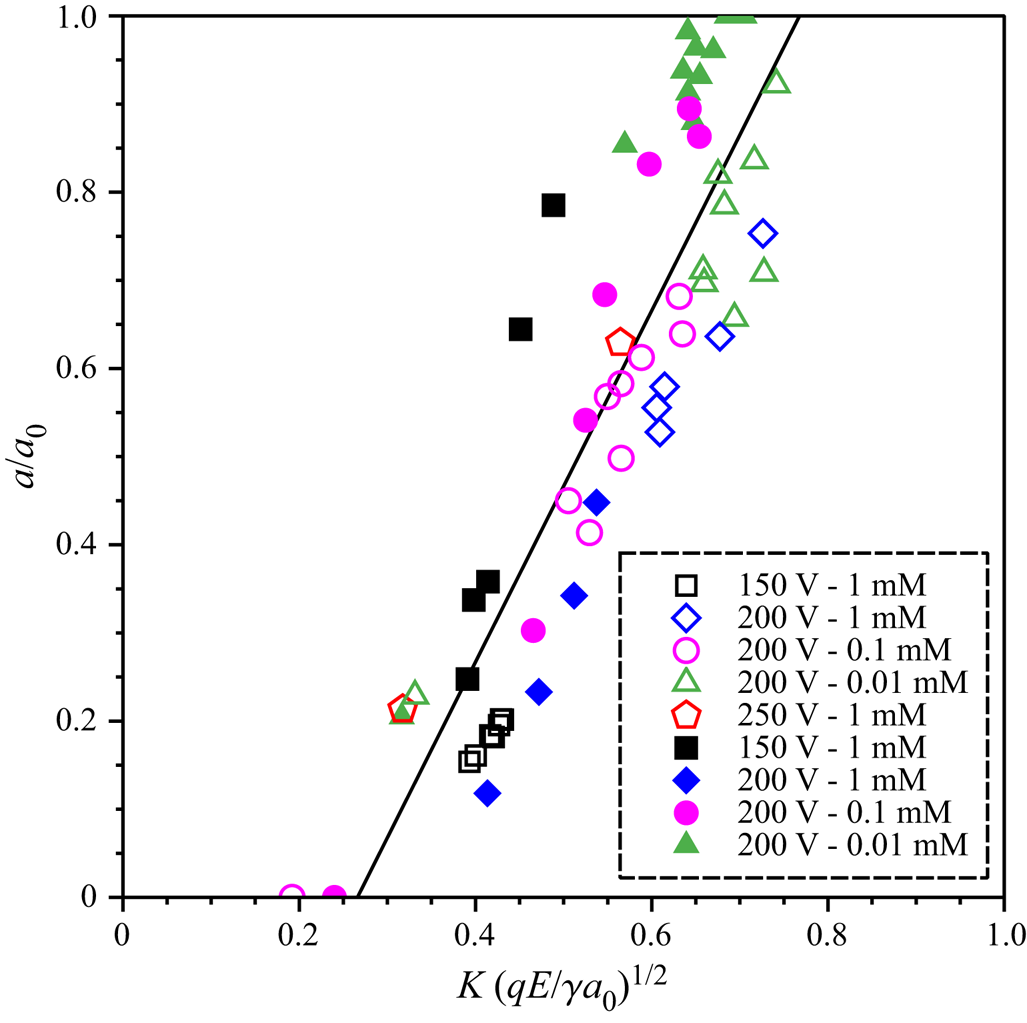
Figure 3. Dimensionless radius of the daughter droplet, plotted as a function of the square root of the electric Bond number. Experiments with different parent droplet diameters, salt concentrations and applied voltages were performed. The results for a parent droplet diameter of 7 $\mu$m are represented as open symbols, those for 11 $\mu$m as full symbols. The droplet charge could not be controlled in the experiments but was determined by measuring the droplet velocity.

Via equation (2), the charge of a droplet is a key factor determining its volume loss upon partial coalescence. To study how the droplet charge evolves when a droplet reciprocates between the two menisci, we observed droplets over a large number of cycles. Figure 4 shows results for two different droplets obtained for a salt concentration of 0.17 mM and a voltage of 250 V. Panel a shows the droplet diameter and panel b the ratio $qE/a$, both as a function of cycle number. The quantities q and a are the droplet charge and radius measured right after partial coalescence with the meniscus at the larger side channel, respectively; $qE/a$ defines the scale of the electrostatic stress on the liquid–liquid interface, in the sense that dividing this ratio by a yields a characteristic value of the stress. It can be seen that the droplets perform a large number of cycles upon which their diameter reduces gradually. After a critical diameter is reached, a rapid volume loss is observed. This transition corresponds to a transition in $qE/a$, which remains approximately constant before the critical diameter is reached and suddenly decreases above the critical diameter. Since the electric field was constant in these experiments, this means that, up to the critical diameter, $q/a$ remains approximately constant. Note that the values of $qE/a$ are of the same order of magnitude as the interfacial tension between the two liquids (~35 mN m⁻¹), which is an indication that the partial coalescence could be governed by a balance between interfacial tension and electrostatic forces. When the voltage is increased to 300 V, qualitatively the same behaviour is observed as in Figure 4a, with a slightly reduced critical diameter (see supplementary information). If the voltage is too low (for the experiments shown in Figure 4a, ~150 V), a critical diameter below which the droplet size rapidly decreases is not observed. The critical diameter is independent of the initial droplet size at the start of the reciprocating motion, where we consider initial diameters larger than the critical diameter. The gradual decrease in droplet diameter visible in Figure 4a can be utilized to consecutively withdraw minute samples from small droplets.

Figure 4. (a) Evolution of the diameter of two different droplets over a large number of cycles. The horizontal line indicates the approximate value of the critical diameter and serves as a guide to the eye. (b) Corresponding evolution of the parameter qE/a. The salt concentration was 0.17 mM and the voltage 250 V.

The discussion of the preceding paragraphs, especially the dependence on the electric Bond number $qE/\gamma {a_0}$, gives some vague hints to the physics underlying the partial coalescence of these micron-sized drops. While we emphasize that the purpose of this manuscript is not to discuss the flow physics in any detail, we wish to briefly comment on the underlying mechanisms. The physics of partial coalescence of millimetre-sized droplets has been studied in some detail (see, for example, Reference Hamlin, Creasey and RistenpartHamlin et al., 2012; Reference Li, Wang, Vivacqua, Ghadiri, Wang, Zhang and WangLi et al., 2020, Reference Li, Dou, Yu, Huang, Zhang, Xu and Wang2021). Compared with that, the droplets considered here have a diameter that is approximately three orders of magnitude smaller, implying that the corresponding dimensionless groups differ vastly as well. This excludes transferring the insights from larger scales to the smaller scales considered here.

We are only aware of one study that discusses the partial coalescence of micron-sized droplets under an electric field (Reference Pillai, Berry, Harvie and DavidsonPillai et al., 2017). In this numerical study, equal properties of the droplet liquid and the oil phase were assumed, a marked difference to our studies where the oil had a viscosity more than three orders of magnitude higher than the droplet. Still, there are some similarities in the results. Roughly, in (Reference Pillai, Berry, Harvie and DavidsonPillai et al. 2017), the partial coalescence mechanism is described as a competition between electrostatic and capillary forces. When a droplet approaches the meniscus, it is already polarized by the electric field, where the part of the droplet that faces the meniscus (the “south pole”) carries charges of a sign opposite to that of the charges at the meniscus. After the droplet gets in touch with the meniscus, charge exchange happens, but most of the charges at the ‘north pole’ of the droplet remain there, inducing a force of the order of $qE$ that pulls the droplet away from the meniscus. This force is counteracted by the capillary force $\gamma {a_0}$ that pushes the droplet towards the meniscus. It is that balance that explains the emergence of the electric Bond number $qE/\gamma {a_0}$. (Reference Pillai, Berry, Harvie and DavidsonPillai et al. 2017) and (Reference Hamlin, Creasey and RistenpartHamlin et al. 2012) agree as far as the relevance of this parameter is concerned, but they report different scaling exponents. Our scaling exponent agrees with that of (Reference Hamlin, Creasey and RistenpartHamlin et al. 2012), but an important difference to both papers is that in our case q is the average between daughter and parent droplet charge, whereas both works referred to define q as the daughter droplet charge. Our choice of the electric Bond number could hint to the fact that during the partial coalescence process charge is continuously transferred to the droplet, which makes a description only in terms of the daughter droplet charge questionable. Apart from that, more work is definitely needed to understand the physics of partial coalescence on such small scales. For example, what comes as a surprise considering the high oil viscosity is the fact that the viscosity does not enter Equation (2), at first sight suggesting that viscous forces do not play a role. Of course, the viscosity may be hidden in the numerical parameters appearing in this equation. Unfortunately, our attempts to study the influence of viscosity were unsuccessful, leaving this issue to be settled by future studies. The same type of silicone oil is also available with a viscosity of 100 cSt. It turned out that, with the lower viscosity silicone oil, upon application of an electric field, the menisci became unstable and the water phase protruded into the main channel. We speculate that surface-active contaminants are responsible for this failure mode.