Article contents
A note on analytic integrability of planar vector fields
Published online by Cambridge University Press: 23 April 2012
Abstract
We give a new characterisation of integrability of a planar vector field at the origin. This allows us to prove that the analytic systems
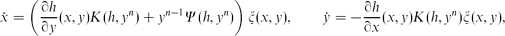 where h, K, Ψ and ξ are analytic functions defined in the neighbourhood of O with K(O) ≠ 0 or Ψ(O) ≠ 0 and n ≥ 1, have a local analytic first integral at the origin. We show new families of analytically integrable systems that are held in the above class. In particular, this class includes all the nilpotent and generalised nilpotent integrable centres that we know.
where h, K, Ψ and ξ are analytic functions defined in the neighbourhood of O with K(O) ≠ 0 or Ψ(O) ≠ 0 and n ≥ 1, have a local analytic first integral at the origin. We show new families of analytically integrable systems that are held in the above class. In particular, this class includes all the nilpotent and generalised nilpotent integrable centres that we know.
Keywords
- Type
- Papers
- Information
- Copyright
- Copyright © Cambridge University Press 2012
References
- 7
- Cited by


