Article contents
Asymptotics of the principal eigenvalue of the Laplacian in 2D periodic domains with small traps
Published online by Cambridge University Press: 04 June 2021
Abstract
We derive and numerically implement various asymptotic approximations for the lowest or principal eigenvalue of the Laplacian with a periodic arrangement of localised traps of small 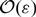 \[\mathcal{O}(\varepsilon )\] spatial extent that are centred at the lattice points of an arbitrary Bravais lattice in
\[\mathcal{O}(\varepsilon )\] spatial extent that are centred at the lattice points of an arbitrary Bravais lattice in  \[{\mathbb{R}^2}\]. The expansion of this principal eigenvalue proceeds in powers of
\[{\mathbb{R}^2}\]. The expansion of this principal eigenvalue proceeds in powers of 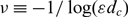 \[\nu \equiv - 1/\log (\varepsilon {d_c})\], where dc is the logarithmic capacitance of the trap set. An explicit three-term approximation for this principal eigenvalue is derived using strong localised perturbation theory, with the coefficients in this series evaluated numerically by using an explicit formula for the source-neutral periodic Green’s function and its regular part. Moreover, a transcendental equation for an improved approximation to the principal eigenvalue, which effectively sums all the logarithmic terms in powers of v, is derived in terms of the regular part of the periodic Helmholtz Green’s function. By using an Ewald summation technique to first obtain a rapidly converging infinite series representation for this regular part, a simple Newton iteration scheme on the transcendental equation is implemented to numerically evaluate the improved ‘log-summed’ approximation to the principal eigenvalue. From a numerical computation of the PDE eigenvalue problem defined on the fundamental Wigner–Seitz (WS) cell for the lattice, it is shown that the three-term asymptotic approximation for the principal eigenvalue agrees well with the numerical result only for a rather small trap radius. In contrast, the log-summed asymptotic result provides a very close approximation to the principal eigenvalue even when the trap radius is only moderately small. For a circular trap, the first few transcendental correction terms that further improves the log-summed approximation for the principal eigenvalue are derived. Finally, it is shown numerically that, amongst all Bravais lattices with a fixed area of the primitive cell, the principal eigenvalue is maximised for a regular hexagonal arrangement of traps.
\[\nu \equiv - 1/\log (\varepsilon {d_c})\], where dc is the logarithmic capacitance of the trap set. An explicit three-term approximation for this principal eigenvalue is derived using strong localised perturbation theory, with the coefficients in this series evaluated numerically by using an explicit formula for the source-neutral periodic Green’s function and its regular part. Moreover, a transcendental equation for an improved approximation to the principal eigenvalue, which effectively sums all the logarithmic terms in powers of v, is derived in terms of the regular part of the periodic Helmholtz Green’s function. By using an Ewald summation technique to first obtain a rapidly converging infinite series representation for this regular part, a simple Newton iteration scheme on the transcendental equation is implemented to numerically evaluate the improved ‘log-summed’ approximation to the principal eigenvalue. From a numerical computation of the PDE eigenvalue problem defined on the fundamental Wigner–Seitz (WS) cell for the lattice, it is shown that the three-term asymptotic approximation for the principal eigenvalue agrees well with the numerical result only for a rather small trap radius. In contrast, the log-summed asymptotic result provides a very close approximation to the principal eigenvalue even when the trap radius is only moderately small. For a circular trap, the first few transcendental correction terms that further improves the log-summed approximation for the principal eigenvalue are derived. Finally, it is shown numerically that, amongst all Bravais lattices with a fixed area of the primitive cell, the principal eigenvalue is maximised for a regular hexagonal arrangement of traps.
MSC classification
- Type
- Papers
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
- 5
- Cited by



