Hostname: page-component-f554764f5-rj9fg Total loading time: 0 Render date: 2025-04-14T13:23:22.770Z Has data issue: false hasContentIssue false
  • English
  • Français

Convergent finite element discretizations of thenonstationary incompressible magnetohydrodynamics system

Published online by Cambridge University Press:  12 August 2008

Andreas Prohl
Andreas Prohl*
Affiliation:
Mathematisches Institut, Universität Tübingen, Auf der Morgenstelle 10, 72076 Tübingen, Germany. [email protected]
Get access

Abstract

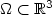The incompressible MHD equations couple Navier-Stokes equations with Maxwell's equationsto describe the flow of a viscous, incompressible, and electrically conducting fluid ina Lipschitz domain $\Omega \subset \mathbb{R}^3$ .We verify convergence of iterates of different coupling anddecoupling fully discrete schemes towards weak solutions forvanishing discretization parameters. Optimal first order of convergence is shown in the presence of strong solutions for a splitting scheme which decouplesthe computation of velocity field, pressure, and magnetic fields atevery iteration step.

Keywords

Magneto-hydrodynamicsdiscretizationFEMfixed-point schemesplitting-method.
Type
Research Article
Information
ESAIM: Mathematical Modelling and Numerical Analysis , Volume 42 , Issue 6 , November 2008 , pp. 1065 - 1087
Copyright
© EDP Sciences, SMAI, 2008

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Amrouche, C., Bernardi, C., Dauge, M. and Girault, V., Vector potentials in three-dimensional nonsmooth domains. Math. Meth. Appl. Sci. 21 (1998) 823–864.
Armero, F. and Simo, J.C., Long-time dissipativity of time-stepping algorithms for an abstract evolution equation with applications to the incompressible MHD and Navier-Stokes equations. Comp. Meth. Appl. Mech. Engrg. 131 (1996) 41–90.
L. Banas and A. Prohl, Convergent finite element discretization of the multi-fluid nonstationary incompressible magnetohydrodynamics equations. (In preparation).
Boffi, D., Fernandes, P., Gastaldi, L. and Perugia, I., Computational models of electromagnetic resonators: analysis of edge element approximation. SIAM J. Numer. Anal. 36 (1999) 1264–1290. CrossRef
S.C. Brenner and L.R. Scott, The mathematical theory of finite element methods. Springer (1994).
Cattabriga, L., Su un problema al contorno relativo al sistemo di equazioni di Stokes. Rend. Sem Mat. Univ. Padova 31 (1961) 308–340.
Chen, Z., Du, Q. and Zou, J., Finite element methods with matching and nonmatching meshes for Maxwell equations with discontinuous coefficients. SIAM J. Numer. Anal. 37 (2000) 1542–1570. CrossRef
Chorin, A.J., Numercial solution of the Navier-Stokes equations. Math. Comp. 22 (1968) 745–762. CrossRef
Costabel, M. and Dauge, M., Weighted regularization of Maxwell equations in polyhedral domains. Numer. Math. 93 (2002) 239–277. CrossRef
Georgescu, V., Some boundary value problems for differential forms on compact Riemannian manifolds. Ann. Math. Pura Appl. 122 (1979) 159–198. CrossRef
Gerbeau, J.-F., A stabilized finite element method for the incompressible magnetohydrodynamic equations. Numer. Math. 87 (2000) 83–111. CrossRef
J.-F. Gerbeau, C. Le Bris and T. Lelievre, Mathematical methods for the magnetohydrodynamics of liquid crystals. Oxford Science Publication (2006).
Girault, V., Nochetto, R.H. and Scott, R., Maximum-norm stability of the finite element Stokes projection. J. Math. Pures Appl. 84 (2005) 279–330.
V. Girault and P.-A. Raviart, Finite element methods for Navier-Stokes equations. Springer (1986).
Gunzburger, M.D., Meir, A.J. and Peterson, J.S., On the existence and uniqueness and finite element approximation of solutions of the equations of stationary incompressible magnetohydrodynamics. Math. Comp. 56 (1991) 523–563. CrossRef
Hasler, U., Schneebeli, A. and Schötzau, D., Mixed finite element approximation of incompressible MHD problems based on weighted regularization. Appl. Numer. Math. 51 (2004) 19–45.
Heywood, J.G. and Rannacher, R., Finite element solution of the nonstationary Navier-Stokes problem, I. Regularity of solutions and second-order error estimates for spatial discretization. SIAM J. Numer. Anal. 19 (1982) 275–311. CrossRef
Hiptmair, R., Finite elements in computational electromagnetism. Acta Numer. 11 (2002) 237–339. CrossRef
Hughes, T.J.R., Franca, L.P. and Balestra, M., A new finite element formulation for computational fluid mechanics: V. Circumventing the Babuska-Brezzi condition: A stable Petrov-Galerkin formulation of the Stokes problem accommodating equal order interpolation. Comp. Meth. Appl. Mech. Eng. 59 (1986) 85–99.
Kikuchi, F., On a discrete compactness property for the Nédélec finite elements. J. Fac. Sci. Univ. Tokyo Sec. IA 36 (1989) 479–490.
P. Monk, Finite element methods for Maxwell's equations. Oxford University Press, New York (2003).
A. Prohl, Projection and quasi-compressibility methods for solving the incompressible Navier-Stokes equations. Teubner-Verlag, Stuttgart (1997).
Prohl, A., On the pollution effect of quasi-compressibility methods in magneto-hydrodynamics and reactive flows. Math. Meth. Appl. Sci. 22 (1999) 1555–1584.
A. Prohl, On pressure approximation via projection methods for nonstationary incompressible Navier-Stokes equations. SIAM J. Numer. Anal. (to appear).
Schötzau, D., Mixed finite element methods for stationary incompressible magneto-hydrodynamics. Numer. Math. 96 (2004) 771–800. CrossRef
Sermange, M. and Temam, R., Some mathematical questions related to the MHD equations. Comm. Pure Appl. Math. 36 (1983) 635–664. CrossRef
Temam, R., Sur l'approximation de la solutoin des equations de Navier-Stokes par la méthode de pas fractionnaires II. Arch. Rat. Mech. Anal. 33 (1969) 377–385.
Zhao, J., Analysis of finite element approximation for time-dependent Maxwell problems. Math. Comp. 73 (2003) 1089–1105. CrossRef