Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Schneider, Reinhold
and
Weber, Toralf
2006.
Wavelets for density matrix computation in electronic structure calculation.
Applied Numerical Mathematics,
Vol. 56,
Issue. 10-11,
p.
1383.
Flad, Heinz-Jürgen
Hackbusch, Wolfgang
Luo, Hongjun
and
Kolb, Dietmar
2006.
Analysis, Modeling and Simulation of Multiscale Problems.
p.
299.
Flad, Heinz-Jürgen
Hackbusch, Wolfgang
and
Schneider, Reinhold
2007.
BestN-term approximation in electronic structure calculations. II. Jastrow factors.
ESAIM: Mathematical Modelling and Numerical Analysis,
Vol. 41,
Issue. 2,
p.
261.
Chinnamsetty, Sambasiva Rao
Espig, Mike
Khoromskij, Boris N.
Hackbusch, Wolfgang
and
Flad, Heinz-Jürgen
2007.
Tensor product approximation with optimal rank in quantum chemistry.
The Journal of Chemical Physics,
Vol. 127,
Issue. 8,
Yserentant, Harry
2007.
The hyperbolic cross space approximation of electronic wavefunctions.
Numerische Mathematik,
Vol. 105,
Issue. 4,
p.
659.
Khoromskij, Boris N.
2008.
On tensor approximation of Green iterations for Kohn-Sham equations.
Computing and Visualization in Science,
Vol. 11,
Issue. 4-6,
p.
259.
Flad, Heinz‐Jürgen
Schneider, Reinhold
and
Schulze, Bert‐Wolfgang
2008.
Asymptotic regularity of solutions to Hartree–Fock equations with Coulomb potential.
Mathematical Methods in the Applied Sciences,
Vol. 31,
Issue. 18,
p.
2172.
Fournais, Søren
Hoffmann-Ostenhof, Maria
Hoffmann-Ostenhof, Thomas
and
Sørensen, Thomas Østergaard
2009.
Analytic Structure of Many-Body Coulombic Wave Functions.
Communications in Mathematical Physics,
Vol. 289,
Issue. 1,
p.
291.
Fournais, Søren
Hoffmann-Ostenhof, Maria
Hoffmann-Ostenhof, Thomas
and
Sørensen, Thomas Østergaard
2009.
Analytic structure of solutions to multiconfiguration equations.
Journal of Physics A: Mathematical and Theoretical,
Vol. 42,
Issue. 31,
p.
315208.
Schneider, Reinhold
2009.
Analysis of the projected coupled cluster method in electronic structure calculation.
Numerische Mathematik,
Vol. 113,
Issue. 3,
p.
433.
Khoromskij, B. N.
and
Khoromskaia, V.
2009.
Multigrid Accelerated Tensor Approximation of Function Related Multidimensional Arrays.
SIAM Journal on Scientific Computing,
Vol. 31,
Issue. 4,
p.
3002.
Chinnamsetty, Sambasiva Rao
Espig, Mike
Flad, Heinz-Jürgen
and
Hackbusch, Wolfgang
2010.
Canonical Tensor Products as a Generalization of Gaussian-type Orbitals.
Zeitschrift für Physikalische Chemie,
Vol. 224,
Issue. 3-4,
p.
681.
Flad, Heinz-Jürgen
Rohwedder, Thorsten
and
Schneider, Reinhold
2010.
Adaptive Methods in Quantum Chemistry.
Zeitschrift für Physikalische Chemie,
Vol. 224,
Issue. 3-4,
p.
651.
Yserentant, Harry
2011.
The mixed regularity of electronic wave functions multiplied by explicit correlation factors.
ESAIM: Mathematical Modelling and Numerical Analysis,
Vol. 45,
Issue. 5,
p.
803.
Flad, Heinz-Jürgen
Harutyunyan, Gohar
Schneider, Reinhold
and
Schulze, Bert-Wolfgang
2011.
Explicit Green operators for quantum mechanical Hamiltonians. I. The hydrogen atom.
Manuscripta Mathematica,
Vol. 135,
Issue. 3-4,
p.
497.
Kreusler, Hans-Christian
and
Yserentant, Harry
2012.
The mixed regularity of electronic wave functions in fractional order and weighted Sobolev spaces.
Numerische Mathematik,
Vol. 121,
Issue. 4,
p.
781.
Flad, Heinz-Jürgen
and
Schneider, Reinhold
2012.
s∗-compressibility of the discrete Hartree-Fock equation.
ESAIM: Mathematical Modelling and Numerical Analysis,
Vol. 46,
Issue. 5,
p.
1055.
Hackbusch, Wolfgang
2014.
Numerical tensor calculus.
Acta Numerica,
Vol. 23,
Issue. ,
p.
651.
Fournais, Søren
and
Sørensen, Thomas Østergaard
2021.
Estimates on derivatives of Coulombic wave functions and their electron densities.
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 2021,
Issue. 775,
p.
1.
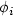 and reduced density matrices ρemerging from Hartree-Fock and density functional theory. The approximation spaces $A^\alpha_q(H^1)$
and reduced density matrices ρemerging from Hartree-Fock and density functional theory. The approximation spaces $A^\alpha_q(H^1)$ 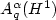 for anisotropicwavelet tensor product bases have been recently characterized by Nitsche in terms of tensor product Besov spaces. We have used the norm equivalence of these spaces to weighted $\ell_q$
for anisotropicwavelet tensor product bases have been recently characterized by Nitsche in terms of tensor product Besov spaces. We have used the norm equivalence of these spaces to weighted $\ell_q$ 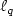 spaces of wavelet coefficients toproof that both $\phi_i$
spaces of wavelet coefficients toproof that both $\phi_i$ 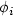 and ρ are in $A^\alpha_q(H^1)$
and ρ are in $A^\alpha_q(H^1)$ 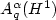 for all $\alpha > 0$
for all $\alpha > 0$ 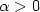 with $\alpha = \frac{1}{q} - \frac{1}{2}$
with $\alpha = \frac{1}{q} - \frac{1}{2}$ 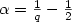 . Our proof is based on the assumption that the $\phi_i$
. Our proof is based on the assumption that the $\phi_i$ 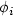 possess an asymptotic smoothness property at the electron-nuclear cusps.
possess an asymptotic smoothness property at the electron-nuclear cusps.