Published online by Cambridge University Press: 15 September 2002
We consider the problem of localizing an
inaccessible piece I of the boundary of a conducting medium Ω, and
a cavity D contained in Ω, from boundary measurements on the
accessible part A of ∂Ω. Assuming that g(t,σ) is
the given thermal flux for (t,σ) ∈ (0,T) x A, and
that the corresponding output datum is the temperature u(T0,σ)
measured at a given time T0 for σ ∈ Aout ⊂ A, we
prove that I and D are uniquely localized from knowledge of all possible
pairs of input-output data $(g,u(T_0)_{\mid A_{{\rm out}}})$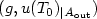 . The same
result holds when a mean value of the temperature is measured over a small
interval of time.
. The same
result holds when a mean value of the temperature is measured over a small
interval of time.