Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Souganidis, Panagiotis E.
and
Perthame, Benoît
2009.
Asymmetric potentials and motor effect: a homogenization approach.
Annales de l'Institut Henri Poincaré C, Analyse non linéaire,
Vol. 26,
Issue. 6,
p.
2055.
Mason, Paolo
and
Sigalotti, Mario
2010.
Generic Controllability Properties for the Bilinear Schrödinger Equation.
Communications in Partial Differential Equations,
Vol. 35,
Issue. 4,
p.
685.
Allaire, Grégoire
Mikelić, Andro
and
Piatnitski, Andrey
2010.
Homogenization Approach to the Dispersion Theory for Reactive Transport through Porous Media.
SIAM Journal on Mathematical Analysis,
Vol. 42,
Issue. 1,
p.
125.
Henning, Patrick
and
Ohlberger, Mario
2010.
The heterogeneous multiscale finite element method for advection-diffusion problems with rapidly oscillating coefficients and large expected drift.
Networks & Heterogeneous Media,
Vol. 5,
Issue. 4,
p.
711.
Freidlin, M.
and
Hu, W.
2011.
Smoluchowski–Kramers approximation in the case of variable friction.
Journal of Mathematical Sciences,
Vol. 179,
Issue. 1,
p.
184.
Perthame, Benoît
and
Souganidis, Panagiotis E.
2011.
A homogenization approach to flashing ratchets.
Nonlinear Differential Equations and Applications NoDEA,
Vol. 18,
Issue. 1,
p.
45.
Allaire, Grégoire
Capdeboscq, Yves
and
Puel, Marjolaine
2012.
Homogenization of a one-dimensional spectral problem for a singularly perturbed elliptic operator with Neumann boundary conditions.
Discrete & Continuous Dynamical Systems - B,
Vol. 17,
Issue. 1,
p.
1.
Armstrong, Scott N.
and
Souganidis, Panagiotis E.
2013.
Concentration phenomena for neutronic multigroup diffusion in random environments.
Annales de l'Institut Henri Poincaré C, Analyse non linéaire,
Vol. 30,
Issue. 3,
p.
419.
Ryzhik, Lenya
Iyer, Gautam
Komorowski, Tomasz
and
Novikov, Alexei
2014.
From homogenization to averaging in cellular flows.
Annales de l'Institut Henri Poincaré C, Analyse non linéaire,
Vol. 31,
Issue. 5,
p.
957.
Henning, Patrick
and
Ohlberger, Mario
2016.
A-posteriori error estimate for a heterogeneous multiscale approximation of advection-diffusion problems with large expected drift.
Discrete and Continuous Dynamical Systems - Series S,
Vol. 9,
Issue. 5,
p.
1393.
Allaire, Grégoire
and
Hutridurga, Harsha
2016.
Upscaling nonlinear adsorption in periodic porous media – homogenization approach.
Applicable Analysis,
Vol. 95,
Issue. 10,
p.
2126.
Zhikov, Vasilii Vasil'evich
and
Pastukhova, Svetlana Evgenievna
2016.
Об операторных оценках в теории усреднения.
Успехи математических наук,
Vol. 71,
Issue. 3(429),
p.
27.
Abdulle, A.
and
Huber, M. E.
2017.
Numerical homogenization method for parabolic advection–diffusion multiscale problems with large compressible flows.
Numerische Mathematik,
Vol. 136,
Issue. 3,
p.
603.
Piatnitski, A.
and
Zhizhina, E.
2019.
Homogenization of biased convolution type operators.
Asymptotic Analysis,
Vol. 115,
Issue. 3-4,
p.
241.
Raveendran, Vishnu
Cirillo, Emilio
and
Muntean, Adrian
2022.
Upscaling of a reaction-diffusion-convection problem with exploding non-linear drift.
Quarterly of Applied Mathematics,
Vol. 80,
Issue. 4,
p.
641.
Jing, Wenjia
and
Zhang, Yiping
2023.
On the Periodic Homogenization of Elliptic Equations in Nondivergence Form with Large Drifts.
Multiscale Modeling & Simulation,
Vol. 21,
Issue. 4,
p.
1486.
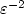 and the drift or first-order term is scaled as $\varepsilon^{-1}$
and the drift or first-order term is scaled as $\varepsilon^{-1}$  . Under a structuralhypothesis on the first cell eigenvalue, which is assumed to admit a unique minimum in the domain withnon-degenerate quadratic behavior, we prove an exponential localization at this minimum point. The homogenizedproblem features a diffusion equation with quadratic potential in the whole space.
. Under a structuralhypothesis on the first cell eigenvalue, which is assumed to admit a unique minimum in the domain withnon-degenerate quadratic behavior, we prove an exponential localization at this minimum point. The homogenizedproblem features a diffusion equation with quadratic potential in the whole space.