Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-22T23:24:06.056Z Has data issue: false hasContentIssue false
  • English
  • Français

A differential inclusion: the case of an isotropic set

Published online by Cambridge University Press:  15 December 2004

Gisella Croce
Gisella Croce*
Affiliation:
Département de Mathématiques, EPFL, 1015 Lausanne, Switzerland; [email protected]
Get access

Abstract

In this article we are interested in the following problem: tofind a map $u: \Omega \to \mathbb{R}^2$ that satisfies $$\left\{\begin{array}{ll}D u \in E\,\, &\mbox{{\it a.e.} in } \Omega\\ u(x)=\varphi(x) &x \in \partial \Omega\end{array}\right.$$ 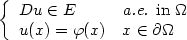where Ω is an open set of $\mathbb{R}^2$ 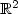and E is acompact isotropic set of $\mathbb{R}^{2\times 2}$ 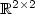. We will show anexistence theorem under suitable hypotheses on φ.

Keywords

Rank one convex hullpolyconvex hulldifferentialinclusionisotropic set.
Type
Research Article
Information
ESAIM: Control, Optimisation and Calculus of Variations , Volume 11 , Issue 1 , January 2005 , pp. 122 - 138
Copyright
© EDP Sciences, SMAI, 2005

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Cardaliaguet, P. and Tahraoui, R., Equivalence between rank-one convexity and polyconvexity for isotropic sets of $\Bbb R\sp {2\times 2}$ . I. Nonlinear Anal. 50 (2002) 1179–1199. CrossRef
G. Croce, Ph.D. Thesis (2004).
B. Dacorogna and P. Marcellini, Implicit partial differential equations. Progr. Nonlinear Diff. Equ. Appl. 37 (1999).
B. Dacorogna and G. Pisante, A general existence theorem for differential inclusions in the vector valued case. Submitted.
M. Gromov, Partial differential relations. Ergeb. Math. Grenzgeb. 9 (1986).
R.A. Horn and C.R. Johnson, Topics in matrix analysis. Cambridge University Press, Cambridge (1991).
Kolář, J., Non-compact lamination convex hulls. Ann. Inst. H. Poincaré Anal. Non Linéaire 20 (2003) 391–403. CrossRef
Müller, S. and Šverák, V., Convex integration for Lipschitz mappings and counterexamples to regularity. Ann. Math. 157 (2003) 715–742. CrossRef
R.T. Rockafellar, Convex analysis. Princeton Landmarks in Mathematics. Princeton University Press, Princeton, NJ (1997). Reprint of the 1970 original, Princeton Paperbacks.