Γ-convergence techniques and relaxation results of constrained energy functionals are used to identify the limiting energy as the thickness ε approaches zero of a ferromagnetic thin structure $\Omega_\varepsilon=\omega\times(-\varepsilon,\varepsilon)$, $\omega\subset\mathbb R^2$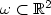, whose energy is given by $$ {\cal E}_{\varepsilon}({\overline{m}})=\frac{1}{\varepsilon} \int_{\Omega_{\varepsilon}}\left(W({\overline{m}},\nabla{\overline{m}}) +{\frac{1}{2}}\nabla {\overline{u}}\cdot {\overline{m}}\right)\,{\rm d}x $$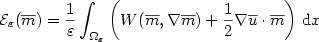 subject to $$ \hbox{div}(-\nabla {\overline{u}}+{\overline{m}}\chi_{\Omega_\varepsilon})=0 \quad\hbox{ on }\mathbb R^3, $$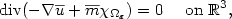 and to the constraint $$ |\overline{m}|=1 \hbox{ on }\Omega_\varepsilon, $$ 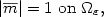where W is any continuous function satisfying p-growth assumptions with p> 1. Partial results are also obtained in the case p=1, under an additional assumption on W.