Let 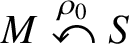$M\stackrel {\rho _0}{\curvearrowleft }S$ be a 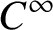$C^\infty $ locally free action of a connected simply connected solvable Lie group S on a closed manifold M. Roughly speaking, 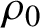$\rho _0$ is parameter rigid if any 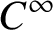$C^\infty $ locally free action of S on M having the same orbits as $\rho _0$ is $C^\infty $ conjugate to 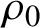$\rho _0$ . In this paper we prove two types of result on parameter rigidity.

First let G be a connected semisimple Lie group with finite center of real rank at least 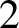$2$ without compact factors nor simple factors locally isomorphic to $\mathop {\mathrm {SO}}\nolimits _0(n,1)(n\,{\geq}\, 2)$ or $\mathop {\mathrm {SU}}\nolimits (n,1)(n\geq 2)$ , and let $\Gamma $ be an irreducible cocompact lattice in G. Let 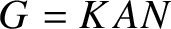$G=KAN$ be an Iwasawa decomposition. We prove that the action $\Gamma \backslash G\curvearrowleft AN$ by right multiplication is parameter rigid. One of the three main ingredients of the proof is the rigidity theorems of Pansu, and Kleiner and Leeb on the quasi-isometries of Riemannian symmetric spaces of non-compact type.

Secondly we show that if $M\stackrel {\rho _0}{\curvearrowleft }S$ is parameter rigid, then the zeroth and first cohomology of the orbit foliation of $\rho _0$ with certain coefficients must vanish. This is a partial converse to the results in the author’s [Vanishing of cohomology and parameter rigidity of actions of solvable Lie groups. Geom. Topol. 21(1) (2017), 157–191], where we saw sufficient conditions for parameter rigidity in terms of vanishing of the first cohomology with various coefficients.