Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-23T18:50:17.519Z Has data issue: false hasContentIssue false
  • English
  • Français

Uniformly positive entropy of induced transformations

Part of: Classical measure theory Basic constructions Topological dynamics Probability theory on algebraic and topological structures

Published online by Cambridge University Press:  28 December 2020

NILSON C. BERNARDES JR ,
UDAYAN B. DARJI Open the ORCID record for UDAYAN B. DARJI [Opens in a new window]  and
RÔMULO M. VERMERSCH
NILSON C. BERNARDES JR
Affiliation:
Departamento de Matemática Aplicada, Instituto de Matemática, Universidade Federal do Rio de Janeiro, Caixa Postal 68530, Rio de Janeiro, RJ21945-970, Brazil (e-mail:[email protected])
UDAYAN B. DARJI*
Affiliation:
Department of Mathematics, University of Louisville, Louisville, KY40208-2772, USA
RÔMULO M. VERMERSCH
Affiliation:
Departamento de Matemática, Centro de Ciências Físicas e Matemáticas, Universidade Federal de Santa Catarina, Florianópolis, SC88040-900, Brazil (e-mail:[email protected])
*
e-mail:[email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Let $(X,T)$ be a topological dynamical system consisting of a compact metric space X and a continuous surjective map $T : X \to X$ . By using local entropy theory, we prove that 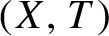$(X,T)$ has uniformly positive entropy if and only if so does the induced system $({\mathcal {M}}(X),\widetilde {T})$ on the space of Borel probability measures endowed with the weak* topology. This result can be seen as a version for the notion of uniformly positive entropy of the corresponding result for topological entropy due to Glasner and Weiss.

Keywords

continuous surjective mapsdynamicsprobability measuresProhorov metricweak* topology

MSC classification

Primary: 37B40: Topological entropy
Secondary: 28A33: Spaces of measures, convergence of measures 60B10: Convergence of probability measures 54B20: Hyperspaces
Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 42 , Issue 1 , January 2022 , pp. 9 - 18
Check for updates
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Adler, R. L., Konheim, A. G. and McAndrew, M. H.. Topological entropy. Trans. Amer. Math. Soc. 114 (1965), 309–319.10.1090/S0002-9947-1965-0175106-9CrossRefGoogle Scholar
Akin, E., Auslander, J. and Nagar, A.. Dynamics of induced systems. Ergod. Th. & Dynam. Sys. 37(7) (2017), 2034–2059.10.1017/etds.2016.7CrossRefGoogle Scholar
Bauer, W. and Sigmund, K.. Topological dynamics of transformations induced on the space of probability measures. Monatsh. Math. 79 (1975), 81–92.10.1007/BF01585664CrossRefGoogle Scholar
Bernardes, N. C. Jr and Darji, U. B.. Graph theoretic structure of maps of the Cantor space. Adv. Math. 231(3–4) (2012), 1655–1680.10.1016/j.aim.2012.05.024CrossRefGoogle Scholar
Bernardes, N. C. Jr, Peris, A. and Rodenas, F.. Set-valued chaos in linear dynamics. Integral Equations Operator Theory 88(4) (2017), 451–463.10.1007/s00020-017-2394-6CrossRefGoogle Scholar
Bernardes, N. C. Jr and Vermersch, R. M.. Hyperspace dynamics of generic maps of the Cantor space. Canad. J. Math. 67(2) (2015), 330–349.10.4153/CJM-2014-005-5CrossRefGoogle Scholar
Bernardes, N. C. Jr and Vermersch, R. M.. On the dynamics of induced maps on the space of probability measures. Trans. Amer. Math. Soc. 368(11) (2016), 7703–7725.10.1090/tran/6615CrossRefGoogle Scholar
Billingsley, P.. Convergence of Probability Measures, 2nd edn. John Wiley & Sons, New York, 1999.10.1002/9780470316962CrossRefGoogle Scholar
Blanchard, F.. Fully positive topological entropy and topological mixing. Symbolic Dynamics and Its Applications (New Haven, CT, 1991) (Contemporary Mathematics, 135). American Mathematical Society, Providence, RI, 1992, pp. 95–105.Google Scholar
Blanchard, F.. A disjointness theorem involving topological entropy. Bull. Soc. Math. France 121(4) (1993), 465–478.10.24033/bsmf.2216CrossRefGoogle Scholar
Darji, U. B. and Kato, H.. Chaos and indecomposability. Adv. Math. 304 (2017), 793–808.10.1016/j.aim.2016.09.012CrossRefGoogle Scholar
Dudley, R. M.. Real Analysis and Probability (Cambridge Studies in Advanced Mathematics, 74). Cambridge University Press, Cambridge, 2002.10.1017/CBO9780511755347CrossRefGoogle Scholar
Fernández, L. and Good, C.. Shadowing for induced maps of hyperspaces. Fund. Math. 235(3) (2016), 277–286.10.4064/fm136-2-2016CrossRefGoogle Scholar
Glasner, E.. A simple characterization of the set of $\mu$ -entropy pairs and applications. Israel J. Math. 102(1) (1997), 13–27.10.1007/BF02773793CrossRefGoogle Scholar
Glasner, E. and Weiss, B.. Dynamics and entropy of the space of measures. C. R. Math. Acad. Sci. Paris Sér. I 317(3) (1993), 239–243.Google Scholar
Glasner, E. and Weiss, B.. Strictly ergodic, uniform positive entropy models. Bull. Soc. Math. France 122(3) (1994), 399–412.10.24033/bsmf.2239CrossRefGoogle Scholar
Glasner, E. and Weiss, B.. Quasi-factors of zero entropy systems. J. Amer. Math. Soc. 8(3) (1995), 665–686.Google Scholar
Glasner, E. and Ye, X.. Local entropy theory. Ergod. Th. & Dynam. Sys. 29(2) (2009), 321–356.10.1017/S0143385708080309CrossRefGoogle Scholar
Guirao, J. L. G., Kwietniak, D., Lampart, M., Oprocha, P. and Peris, A.. Chaos on hyperspaces. Nonlinear Anal. 71(1–2) (2009), 1–8.10.1016/j.na.2008.10.055CrossRefGoogle Scholar
Huang, W. and Ye, X.. A local variational relation and applications. Israel J. Math. 151 (2006), 237–279.10.1007/BF02777364CrossRefGoogle Scholar
Illanes, A. and Nadler, S. B. Jr. Hyperspaces: Fundamentals and Recent Advances. Marcel Dekker, New York, 1999.Google Scholar
Kechris, A. S.. Classical Descriptive Set Theory. Springer, New York, 1995.10.1007/978-1-4612-4190-4CrossRefGoogle Scholar
Kerr, D. and Li, H.. Dynamical entropy in Banach spaces. Invent. Math. 162(3) (2005), 649–686.10.1007/s00222-005-0457-9CrossRefGoogle Scholar
Kerr, D. and Li, H.. Independence in topological and ${C}^{\ast }$ -dynamics. Math. Ann. 338(4) (2007), 869–926.10.1007/s00208-007-0097-zCrossRefGoogle Scholar
Kwietniak, D. and Oprocha, P.. Topological entropy and chaos for maps induced on hyperspaces. Chaos Solitons Fractals 33(1) (2007), 76–86.10.1016/j.chaos.2005.12.033CrossRefGoogle Scholar
Li, J., Oprocha, P. and Wu, X.. Furstenberg families, sensitivity and the space of probability measures. Nonlinearity 30(3) (2017), 987–1005.10.1088/1361-6544/aa5495CrossRefGoogle Scholar
Li, J., Yan, K. and Ye, X.. Recurrence properties and disjointness on the induced spaces. Discrete Contin. Dyn. Syst. 35(3) (2015), 1059–1073.10.3934/dcds.2015.35.1059CrossRefGoogle Scholar
Qiao, Y. and Zhou, X.. Zero sequence entropy and entropy dimension. Discrete Contin. Dyn. Syst. 37(1) (2017), 435–448.10.3934/dcds.2017018CrossRefGoogle Scholar
Sigmund, K.. Affine transformations on the space of probability measures. Astérisque 51 (1978), 415–427.Google Scholar