Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-19T04:36:05.657Z Has data issue: false hasContentIssue false
  • English
  • Français

Topology and convexity in the space of actions modulo weak equivalence

Published online by Cambridge University Press:  03 April 2017

PETER BURTON
PETER BURTON*
Affiliation:
Department of Mathematics, California Institute of Technology, Pasadena, CA 91125, USA email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We analyze the structure of the quotient $\text{A}_{{\sim}}(\unicode[STIX]{x1D6E4},X,\unicode[STIX]{x1D707})$ of the space of measure-preserving actions of a countable discrete group by the relation of weak equivalence. This space carries a natural operation of convex combination. We introduce a variant of an abstract construction of Fritz which encapsulates the convex combination operation on 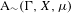$\text{A}_{{\sim}}(\unicode[STIX]{x1D6E4},X,\unicode[STIX]{x1D707})$. This formalism allows us to define the geometric notion of an extreme point. We also discuss a topology on $\text{A}_{{\sim}}(\unicode[STIX]{x1D6E4},X,\unicode[STIX]{x1D707})$ due to Abért and Elek in which it is Polish and compact, and show that this topology is equivalent to others defined in the literature. We show that the convex structure of 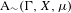$\text{A}_{{\sim}}(\unicode[STIX]{x1D6E4},X,\unicode[STIX]{x1D707})$ is compatible with the topology, and as a consequence deduce that 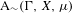$\text{A}_{{\sim}}(\unicode[STIX]{x1D6E4},X,\unicode[STIX]{x1D707})$ is path connected. Using ideas of Tucker-Drob, we are able to give a complete description of the topological and convex structure of 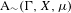$\text{A}_{{\sim}}(\unicode[STIX]{x1D6E4},X,\unicode[STIX]{x1D707})$ for amenable $\unicode[STIX]{x1D6E4}$ by identifying it with the simplex of invariant random subgroups. In particular, we conclude that 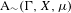$\text{A}_{{\sim}}(\unicode[STIX]{x1D6E4},X,\unicode[STIX]{x1D707})$ can be represented as a compact convex subset of a Banach space if and only if $\unicode[STIX]{x1D6E4}$ is amenable. In the case of general $\unicode[STIX]{x1D6E4}$ we prove a Krein–Milman-type theorem asserting that finite convex combinations of the extreme points of 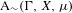$\text{A}_{{\sim}}(\unicode[STIX]{x1D6E4},X,\unicode[STIX]{x1D707})$ are dense in this space. We also consider the space 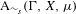$\text{A}_{{\sim}_{s}}(\unicode[STIX]{x1D6E4},X,\unicode[STIX]{x1D707})$ of stable weak equivalence classes and show that it can always be represented as a compact convex subset of a Banach space. In the case of a free group 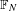$\mathbb{F}_{N}$, we show that if one restricts to the compact convex set 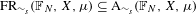$\text{FR}_{{\sim}_{s}}(\mathbb{F}_{N},X,\unicode[STIX]{x1D707})\subseteq \text{A}_{{\sim}_{s}}(\mathbb{F}_{N},X,\unicode[STIX]{x1D707})$ consisting of the stable weak equivalence classes of free actions, then the extreme points are dense in 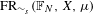$\text{FR}_{{\sim}_{s}}(\mathbb{F}_{N},X,\unicode[STIX]{x1D707})$.

Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 38 , Issue 7 , October 2018 , pp. 2508 - 2536
Copyright
© Cambridge University Press, 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abért, M. and Elek, G.. The space of actions, partition metric and combinatorial rigidity. Preprint, 2011, arXiv:1108.2147.Google Scholar
Abért, M., Glasner, Y. and Virág, B.. Kesten’s theorem for invariant random subgroups. Duke Math. J. 163(3) (2014), 465–488.Google Scholar
Abért, M. and Weiss, B.. Bernoulli actions are weakly contained in any free action. Ergod. Th. & Dynam. Sys. 33(2) (2013), 323–333.Google Scholar
Bowen, L.. Invariant random subgroups of the free group. Groups Geom. Dyn. 9(3) (2015), 891–916.Google Scholar
Bowen, L., Grigorchuk, R. and Kravchenko, R.. Invariant random subgroups of lamplighter groups. Israel J. Math. 207(2) (2015), 763–782.Google Scholar
Capraro, V. and Fritz, T.. On the axiomatization of convex subsets of Banach spaces. Proc. Amer. Math. Soc. 141(6) (2013), 2127–2135.Google Scholar
Conley, C. T., Kechris, A. S. and Tucker-Drob, R. D.. Ultraproducts of measure preserving actions and graph combinatorics. Ergod. Th. & Dynam. Sys. 33 (2013), 334–374.Google Scholar
Eisenmann, A. and Glasner, Y.. Generic IRS in free groups, after Bowen. Proc. Amer. Math. Soc. 144(10) (2016), 4231–4246.Google Scholar
Fritz, T.. Convex spaces I: definition and examples. Preprint, 2009, http://arxiv.org/pdf/0903.5522.pdf.Google Scholar
Kechris, A. S.. Classical Descriptive Set Theory (Graduate Texts in Mathematics, 156) . Springer, New York, 1995.Google Scholar
Kechris, A. S.. Global Aspects of Ergodic Group Actions (Mathematical Surveys and Monographs, 160) . American Mathematical Society, Providence, RI, 2010.Google Scholar
Kechris, A. S. and Tsankov, T.. Amenable actions and almost invariant sets. Proc. Amer. Math. Soc. 136 (2008), 687–697.Google Scholar
Tucker-Drob, R. D.. Weak equivalence and non-classifiability of measure preserving actions. Ergod. Th. & Dynam. Sys. 35(1) (2015), 293–336.Google Scholar