Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-26T01:11:00.556Z Has data issue: false hasContentIssue false
  • English
  • Français

Templates for geodesic flows

Published online by Cambridge University Press:  28 November 2012

TALI PINSKY
TALI PINSKY*
Affiliation:
The Technion, Israeli Institute of Technology, Mathematics Department, Haifa 32000, Israel (email: [email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

We construct templates for geodesic flows on an infinite family of Hecke triangle groups. Our results generalize those of E. Ghys [Knots and dynamics. Proc. Int. Congress of Mathematicians. Vol. 1. International Congress of Mathematicians, Zürich, 2007], who constructed a template for the modular flow in the complement of the trefoil knot in 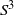$S^3$. A significant difficulty that arises in any attempt to go beyond the modular flow is the fact that for other Hecke triangles the geodesic flow cannot be viewed as a flow in 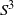$S^3$, and one is led to consider embeddings into lens spaces. Our final result is an explicit description of a single ‘Hecke template’ which contains all other templates we construct, allowing a topological study of the periodic orbits of different Hecke triangle groups all at once.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 34 , Issue 1 , February 2014 , pp. 211 - 235
Copyright
Copyright © 2012 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1]Berry, M. V.. Quantum theory near the classical limit. Highlights Phys. (SERC) (1990), 50–51.Google Scholar
[2]Birman, J.. Book review: Braid groups, ordering braids. Bull. Amer. Math. Soc. 48(1) (2011), 137–146.CrossRefGoogle Scholar
[3]Birman, J. and Williams, R. F.. Knotted periodic orbits in dynamical systems–I: Lorenz’s equations. Topology 22(1) (1983), 47–82.CrossRefGoogle Scholar
[4]Birman, J. and Williams, R. F.. Knotted periodic orbits in dynamical systems-II: Knot holders for fibered knots. Cont. Math. 20 (1983), l–60.Google Scholar
[5]Cvitanovic, P.. Dynamical averaging in terms of periodic orbits. Physica D 83 (1995), 109–123.Google Scholar
[6]Dehornoy, P.. Les noeuds de Lorenz. L’enseignement Math. (2) 57 (2011), 211–280.CrossRefGoogle Scholar
[7]Dehornoy, P.. Geodesic flow, left-handedness, and templates. Preprint, 2011, arXiv:1112.6296.Google Scholar
[8]Ghrist, R. W., Holmes, P. J. and Sullivan, M. C.. Knots and Links in Three-Dimensional Flows. Princeton University Press, Princeton, NJ, 1997.Google Scholar
[9]Ghrist, R. W.. Branched two-manifolds supporting all links. Topology 36 (1997), 423–448.Google Scholar
[10]Ghys, E.. Knots and dynamics. Proc. Int. Congress of Mathematicians. Vol. 1. International Congress of Mathematicians, Zürich, 2007.Google Scholar
[11]Mackenzie, D.. A new twist in knot theory. What’s Happening Math. Sci. 7 (2009), 2–17.Google Scholar
[12]Montesinos, J. M.. Classical Tessellations and Three-Manifolds. Springer, 1987.Google Scholar
[13]Pinsky, T.. Primeness of Hecke knots, in preparation.Google Scholar
[14]Rolfsen, D.. Knots and Links. American Mathematical Society, Providence, RI, 2003.Google Scholar
[15]Seifert, H.. Topology of 3-dimensional fibered spaces. Acta. Math. 60 (1933), 147–288.CrossRefGoogle Scholar
[16]Steenrod, N.. The Topology of Fiber Bundles. Princeton University Press, Princeton, NJ, 1951.Google Scholar
[17]Sullivan, M.. Prime decomposition of knots in Lorenz-like templates. J. Knot Theory Ramifications 2(4) (1993), 453–462.Google Scholar
[18]Sullivan, M.. Factoring families of positive knots on Lorenz-like templates. J. Knot Theory Ramifications 17(10) (2008).Google Scholar
[19]Tsanov, V. V.. Triangle groups, automorphic forms, and torus knots. Preprint, arXiv:1011.0461v2.Google Scholar
[20]Watkins, M.. A short survey of lens spaces. Undergraduate dissertation, University of Kent at Canterbury, 1990.Google Scholar