Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-24T14:25:24.709Z Has data issue: false hasContentIssue false
  • English
  • Français

A survey on spectral multiplicities of ergodic actions

Published online by Cambridge University Press:  09 December 2011

ALEXANDRE I. DANILENKO
ALEXANDRE I. DANILENKO*
Affiliation:
Institute for Low Temperature Physics & Engineering of National Academy of Sciences of Ukraine, 47 Lenin Ave., Kharkov, 61164, Ukraine (email: [email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

Given a transformation T of a standard measure space (X,μ), let ℳ(T) denote the set of spectral multiplicities of the Koopman operator UT defined in by UTf:=f∘T. In this survey paper we discuss which subsets of 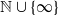are realizable as ℳ(T) for various T: ergodic, weakly mixing, mixing, Gaussian, Poisson, ergodic infinite measure-preserving, etc. The corresponding constructions are considered in detail. Generalizations to actions of Abelian locally compact second countable groups are also discussed.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 33 , Issue 1 , February 2013 , pp. 81 - 117
Copyright
Copyright © Cambridge University Press 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[AbLe]El Abdalaoui, E. H. and Lemańczyk, M.. Approximately transitive dynamical systems and simple spectrum. Archiv. Math. 97 (2011), 187–197.CrossRefGoogle Scholar
[Ad]Adams, T. M.. Smorodinsky’s conjecture on rank one systems. Proc. Amer. Math. Soc. 126 (1998), 739–744.CrossRefGoogle Scholar
[Ag1]Ageev, O. N.. Dynamical systems with even-multiplicity Lebesgue component in the spectrum. Sb. Math. 64 (1989), 305–317.CrossRefGoogle Scholar
[Ag2]Ageev, O. N.. On ergodic transformations with homogeneous spectrum. J. Dyn. Control Syst. 5 (1999), 149–152.CrossRefGoogle Scholar
[Ag3]Ageev, O. N.. The spectral multiplicity function and geometric representations of interval exchange transformations. Sb. Math. 190 (1999), 1–28.CrossRefGoogle Scholar
[Ag4]Ageev, O. N.. On the spectrum of Cartesian powers of classical automorphisms. Math. Notes 68 (2000), 547–551.CrossRefGoogle Scholar
[Ag5]Ageev, O. N.. On the multiplicity function of generic group extensions with continuous spectrum. Ergod. Th. & Dynam. Sys. 21 (2001), 321–338.CrossRefGoogle Scholar
[Ag6]Ageev, O. N.. The homogeneous spectrum problem in ergodic theory. Invent. Math. 160 (2005), 417–446.CrossRefGoogle Scholar
[Ag7]Ageev, O. N.. Mixing with staircase multiplicity fuction. Ergod. Th. & Dynam. Sys. 28 (2008), 1687–1700.CrossRefGoogle Scholar
[Ba]Baxter, J. R.. A class of ergodic transformations having simple spectrum. Proc. Amer. Math. Soc. 27 (1971), 275–279.CrossRefGoogle Scholar
[BlLe]Blanchard, F. and Lemańczyk, M.. Measure-preserving diffeomorphisms with an arbitrary spectral multiplicity. Topol. Methods Nonlinear Anal. 1 (1993), 275–294.CrossRefGoogle Scholar
[Ch]Chacon, R. V.. Approximation and spectral multiplicity. Contributions to Ergodic Theory and Probability (Proc. First Midwestern Conf. Ergodic Theory, Athens, OH, 1970) (Lecture Notes in Mathematics, 160). 1970, pp. 18–27.CrossRefGoogle Scholar
[CoWo]Connes, A. and Woods, E. J.. Approximately transitive flows and ITPFI factors. Ergod. Th. & Dynam. Sys. 5 (1985), 203–236.CrossRefGoogle Scholar
[Co-Sin]Cornfeld, I., Fomin, S. and Sinai, Ya. G.. Ergodic Theory. Springer, New York, 1982.CrossRefGoogle Scholar
[CrSi]Creutz, D. and Silva, C.. Mixing on rank-one transformations. Studia Math. 199 (2010), 43–72.CrossRefGoogle Scholar
[Da1]Danilenko, A. I.. Explicit solution of Rokhlin’s problem on homogeneous spectrum and applications. Ergod. Th. & Dynam. Sys. 26 (2006), 1467–1490.CrossRefGoogle Scholar
[Da2]Danilenko, A. I.. (C,F)-actions in ergodic theory geometry and dynamics of groups and spaces. Progr. Math. 265 (2008), 325–351.CrossRefGoogle Scholar
[Da3]Danilenko, A. I.. On new spectral multiplicities for ergodic maps. Studia Math. 197 (2010), 57–68.CrossRefGoogle Scholar
[Da4]Danilenko, A. I.. New spectral multiplicities for mixing transformations. Ergod. Th. & Dynam. Sys. to appear. doi:10.1017/SO143385710000672.CrossRefGoogle Scholar
[DaLe]Danilenko, A. I. and Lemańczyk, M.. Spectral multiplicities for ergodic flows. Discrete Contin. Dyn. Syst. to appear.Google Scholar
[DaRy1]Danilenko, A. I. and Ryzhikov, V. V.. Spectral multiplicities for infinite measure preserving transformations. Funct. Anal. Appl. 44 (2010), 161–170.CrossRefGoogle Scholar
[DaRy2]Danilenko, A. I. and Ryzhikov, V. V.. Mixing constructions with infinite invariant measure and spectral multiplicities. Ergod. Th. & Dynam. Sys. 31 (2011), 853–873.CrossRefGoogle Scholar
[DaSi]Danilenko, A. I. and Silva, C. E.. Ergodic Theory: Nonsingular Transformations (Encyclopedia of Complexity and Systems Science). Springer, 2009, pp. 3055–3083.Google Scholar
[DaSo]Danilenko, A. I. and Solomko, A. V.. Ergodic Abelian Actions with Homogeneous Spectrum (Contemporary Mathematics, 532). American Mathematical Society, Providence, RI, 2010, pp. 137–148.Google Scholar
[dJ1]del Junco, A.. Transformations with discrete spectrum are stacking transformations. Canad. J. Math. 28 (1976), 836–839.CrossRefGoogle Scholar
[dJ2]del Junco, A.. A transformation with simple spectrum which is not rank one. Canad. J. Math. 29 (1977), 655–663.CrossRefGoogle Scholar
[dJRu]del Junco, A. and Rudolph, D.. A rank one, rigid, simple, prime map. Ergod. Th. & Dynam. Sys. 7 (1987), 229–247.CrossRefGoogle Scholar
[dR]de la Rue, T.. Rang des systèmes dynamiques gaussiens. Israel J. Math. 104 (1998), 261–283.CrossRefGoogle Scholar
[FiKw]Fillipowicz, I. and Kwiatkowski, J.. Rank, covering number and a simple spectrum. J. Anal. Math. 66 (1995), 185–215.CrossRefGoogle Scholar
[FoWi]Forman, M. and Weiss, B.. An anti-classification theorem for ergodic measure preserving transformations. J. Eur. Math. Soc. (JEMS) 6 (2004), 277–292.CrossRefGoogle Scholar
[Fr]Fra̧czek, K.. Cyclic space isomorphism of unitary operators. Studia Math. 124 (1997), 259–267.CrossRefGoogle Scholar
[Fu]Furstenberg, H.. Recurrence in Ergodic Theory and Combinatorial Number Theory. Princeton University Press, Princeton, NJ, 1981.CrossRefGoogle Scholar
[Gi]Girsanov, I. V.. Spectra of dynamical systems generated by stationary Gaussian processes. Dokl. Akad. Nauk SSSR (N.S.) 119 (1958), 851–853 (in Russian).Google Scholar
[Gl-We]Glasner, E., Thouvenot, J.-P. and Weiss, B.. Every countable group has the weak Rohlin property. Bull. Lond. Math. Soc. 38 (2006), 932–936.CrossRefGoogle Scholar
[GolSi]Golodets, V. Ya. and Sinelshchikov, S. D.. Classification and structure of cocycles of amenable ergodic equivalence relations. J. Funct. Anal. 121 (1994), 455–485.CrossRefGoogle Scholar
[Go]Goodson, G. R.. A survey of recent results in the spectral theory of ergodic dynamical systems. J. Dyn. Control Syst. 5 (1999), 173–226.CrossRefGoogle Scholar
[Go-Li]Goodson, G. R., Kwiatkowski, J., Lemańczyk, M. and Liardet, P.. On the multiplicity function of ergodic group extensions of rotations. Studia Math. 102 (1992), 157–174.CrossRefGoogle Scholar
[Kal]Kalikov, S. A.. Twofold mixing implies threefold mixing for rank one transformations. Ergod. Th. & Dynam. Sys. 4 (1984), 237–259.CrossRefGoogle Scholar
[Ka]Katok, A. B.. Combinatorial Constructions in Ergodic Theory and Dynamics (University Lecture Series, 30). American Mathematical Society, Providence, RI, 2003.CrossRefGoogle Scholar
[KaLe]Katok, A. and Lemańczyk, M.. Some new cases of realization of spectral multiplicity function for ergodic transformations. Fund. Math. 206 (2009), 185–215.CrossRefGoogle Scholar
[KaSt]Katok, A. B. and Stepin, A. M.. Approximations in ergodic theory. Russian Math. Surveys 22 (1967), 77–102.CrossRefGoogle Scholar
[KaTh]Katok, A. B. and Thouvenot, J.-P.. Spectral Properties and Combinatorial Constructions in Ergodic Theory (Handbook of dynamical systems, 1B). Elsevier, Amsterdam, 2006, pp. 649–743.Google Scholar
[Ki]Kirillov, A. A.. Elements of the Theory of Representations. Springer, Berlin, 1976.CrossRefGoogle Scholar
[KwLa]Kwiatkowski, J. and Lacroix, Y.. Multiplicity, rank pairs. J. Anal. Math. 71 (1997), 205–235.CrossRefGoogle Scholar
[KwiLe]Kwiatkowski (Jr), J. and Lemańczyk, M.. On the multiplicity function of ergodic group extensions. II Studia Math. 116 (1995), 207–215.CrossRefGoogle Scholar
[Le1]Lemańczyk, M.. Toeplitz -extensions. Ann. Inst. Henri Poincare Probab. Stat. 24 (1988), 1–43.Google Scholar
[Le2]Lemańczyk, M.. Introduction to ergodic theory from the point of view of the spectral theory. Lecture Notes of the Tenth KAIST Mathematics Workshop. Korea Advanced Institute of Science and Tenology Mathematics Research, Taejon, 1996.Google Scholar
[Le3]Lemańczyk, M.. Spectral theory of dynamical systems. Encyclopedia of Complexity and Systems Science. Springer, 2009.Google Scholar
[Le4]Lemańczyk, M.. Teoria spektralna dla ergodyków (in Polish). Preprint, available at http://www-users.mat.umk.pl/∼mlem/didactics.php.Google Scholar
[LePa]Lemańczyk, M. and Parreau, F.. Special flows over irrational rotations with the simple convolution property. Available at http://www-users.mat.umk.pl/∼mlem/files/SC-16042011.pdf.Google Scholar
[Le-Th]Lemańczyk, M., Parreau, F. and Thouvenot, J.-P.. Gaussian automorphisms whose ergodic self-joinings are Gaussian. Fund. Math. 164 (2000), 253–293.CrossRefGoogle Scholar
[Ma]Mackey, G.. Induced representations of locally compact groups. I. Ann. of Math. (2) 55 (1952), 101–139.CrossRefGoogle Scholar
[MatNa]Mathew, J. and Nadkarni, M. G.. A measure-preserving transformation whose spectrum has a Lebesgue component of multiplicity two. Bull. Lond. Math. Soc. 16 (1984), 402–406.CrossRefGoogle Scholar
[Na]Nadkarni, M. G.. Spectral Theory of Dynamical Systems (Birkhäuser Advanced Texts: Basler Lehrbücher). Birkhäuser, Basel, 1998.Google Scholar
[Nai]Naimark, M.. Normed Rings. Noordhoff, Groningen, 1959.Google Scholar
[Ne]Neretin, Yu.. Categories of Symmetries and Infinite Dimensional Groups. Oxford University Press, Oxford, 1986.Google Scholar
[New]Newton, D.. On Gaussian processes with simple spectrum. Z. Wahr. Verw. Geb. 5 (1966), 207–209.CrossRefGoogle Scholar
[Or]Ornstein, D. S.. On the root problem in ergodic theory. Proc. Sixth Berkley Symp. Math. Stat. Prob. (University of California, Berkeley, CA, 1970/1971). Volume II: Probability Theory. University of California Press, Berkeley, CA, 1972, pp. 347–356.Google Scholar
[Os]Oseledec, V. I.. On the spectrum of ergodic automorphisms. Soviet Math. Dokl. 168 (1966), 776–779.Google Scholar
[Pr]Prikhodko, A.. On flat trigonometric sums and ergodic flow with simple Lebesgue spectrum. Preprint, arXiv:1002.2808.Google Scholar
[PrRy]Prikhodko, A. and Ryzhikov, V. V.. Disjointness of the convolutions for Chacon’s transformations. Colloq. Math. 84/85 (2000), 67–74.CrossRefGoogle Scholar
[Ro1]Robinson, E. A.. Ergodic measure-preserving transformations with arbitrary finite spectral multiplicities. Invent. Math. 72 (1983), 299–314.CrossRefGoogle Scholar
[Ro2]Robinson, E. A.. Mixing and spectral multiplicities. Ergod. Th. & Dynam. Sys. 5 (1985), 617–624.CrossRefGoogle Scholar
[Ro3]Robinson, E. A.. Transformations with highly nonhomogeneous spectrum of finite multiplicity. Israel J. Math. 56 (1986), 75–88.CrossRefGoogle Scholar
[Ro4]Robinson, E. A.. Nonabelian extensions have nonsimple spectrum. Compos. Math. 65 (1988), 155–170.Google Scholar
[Roy]Roy, E.. Poisson suspensions and infinite ergodic theory. Ergod. Th. & Dynam. Sys. 29 (2009), 667–683.CrossRefGoogle Scholar
[Ry1]Ryzhikov, V. V.. Mixing, rank and minimal self-joinings of actions with invariant measure. Sb. Math. 183 (1992), 133–160.Google Scholar
[Ry2]Ryzhikov, V. V.. Transformations having homogeneous spectra. J. Dyn. Control Syst. 5 (1999), 145–148.CrossRefGoogle Scholar
[Ry3]Ryzhikov, V. V.. Homogeneous spectrum, disjointness of convolutions, and mixing properties of dynamical systems. Selected Russian Mathematics 1 (1999), 13–24.Google Scholar
[Ry4]Ryzhikov, V. V.. Weak limits of powers, the simple spectrum of symmetric products and mixing constructions of rank 1. Sb. Math. 198 (2007), 733–754.CrossRefGoogle Scholar
[Ry5]Ryzhikov, V. V.. Spectral multiplicities and asymptotic operator properties of actions with invariant measure. Sb. Math. 200 (2009), 1833–1845.CrossRefGoogle Scholar
[Ry6]Ryzhikov, V. V.. Spectral multiplicities for powers of weakly mixing transformations. Preprint, arXiv:1102.3068.Google Scholar
[Ry7]Ryzhikov, V. V.. Simple spectrum for tensor products of mixing map powers. Preprint, arXiv:1107.4745.Google Scholar
[Ry8]Ryzhikov, V. V.. On mixing of staircase transformations. Preprint, arXiv:1108.3522.Google Scholar
[Ru]Rudolph, D.. k-fold mixing lifts to weakly mixing isometric extensions. Ergod. Th. & Dynam. Sys. 5 (1985), 445–447.CrossRefGoogle Scholar
[Sc]Schmidt, K.. Cocycles of Ergodic Transformation Groups (Lecture Notes in Mathematics, 1). Macmillan Publishers India, Delhi, 1977.Google Scholar
[So]Solomko, A. V.. New spectral multiplicities for ergodic actions. Preprint arXiv:1109.4367.Google Scholar
[St]Stepin, A. M.. Spectral properties of typical dynamical systems. Mathematics of the USSR-Izvestiya 29 (1987), 159–192.CrossRefGoogle Scholar
[Ti1]Tikhonov, S. V.. A complete metric on the set of mixing transformations. Sb. Math. 198 (2007), 575–596.CrossRefGoogle Scholar
[Ti2]Tikhonov, S. V.. Mixing transformations with homogeneous spectrum Sb. Math. to appear.Google Scholar
[Zi]Zimmer, R.. Induced and amenable ergodic actions of Lie groups. Ann. Sci. Éc Norm. Supér. (4) 11 (1978), 407–428.CrossRefGoogle Scholar