Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-26T03:09:09.791Z Has data issue: false hasContentIssue false
  • English
  • Français

Sarnak’s conjecture for sequences of almost quadratic word growth

Part of: Multiplicative number theory Probabilistic theory: distribution modulo $1$; metric theory of algorithms Ergodic theory

Published online by Cambridge University Press:  04 December 2020

REDMOND MCNAMARA
REDMOND MCNAMARA*
Affiliation:
UCLALos Angeles, CA, USA (e-mail: [email protected])
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We prove the logarithmic Sarnak conjecture for sequences of subquadratic word growth. In particular, we show that the Liouville function has at least quadratically many sign patterns. We deduce the main theorem from a variant which bounds the correlations between multiplicative functions and sequences with subquadratically many words which occur with positive logarithmic density. This allows us to actually prove that our multiplicative functions do not locally correlate with sequences of subquadratic word growth. We also prove a conditional result which shows that if the (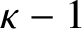 $\kappa -1$ )-Fourier uniformity conjecture holds then the Liouville function does not correlate with sequences with 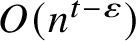$O(n^{t-\varepsilon })$ many words of length n where $t = \kappa (\kappa +1)/2$ . We prove a variant of the 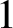$1$ -Fourier uniformity conjecture where the frequencies are restricted to any set of box dimension less than $1$ .

Keywords

arithmetic and algebraic dynamicsnumber theoryclassical ergodic theory

MSC classification

Primary: 37A45: Relations with number theory and harmonic analysis 11N37: Asymptotic results on arithmetic functions
Secondary: 11K65: Arithmetic functions
Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 41 , Issue 10 , October 2021 , pp. 3060 - 3115
Check for updates
Creative Commons
Creative Common License - CCCreative Common License - BYCreative Common License - NCCreative Common License - SA
This is an Open Access article, distributed under the terms of the Creative Commons Attribution-NonCommercial-ShareAlike licence (http://creativecommons.org/licenses/by-nc-sa/4.0/), which permits non-commercial re-use, distribution, and reproduction in any medium, provided the same Creative Commons licence is included and the original work is properly cited. The written permission of Cambridge University Press must be obtained for commercial re-use.
Copyright
© The Author(s), 2020. Published by Cambridge University Press

References

REFERENCES

Bourgain, J., Demeter, C. and Guth, L.. Proof of the main conjecture in Vinogradov’s mean value theorem for degrees higher than three. Ann. of Math. (2) 184(2) (2016), 633–682.10.4007/annals.2016.184.2.7CrossRefGoogle Scholar
Bourgain, J.. Möbius-Walsh correlation bounds and an estimate of Mauduit and Rivat. J. Anal. Math. 119 (2013), 147–163.10.1007/s11854-013-0005-2CrossRefGoogle Scholar
Bourgain, J.. On the correlation of the Moebius function with rank-one systems. J. Anal. Math. 120 (2013), 105–130.CrossRefGoogle Scholar
Bourgain, J., Sarnak, P. and Ziegler, T.. Disjointness of Moebius from horocycle flows. From Fourier Analysis and Number Theory to Radon Transforms and Geometry. Springer, New York, NY, 2013, pp. 67–83.10.1007/978-1-4614-4075-8_5CrossRefGoogle Scholar
Downarowicz, T. and Kasjan, S.. Odometers and Toeplitz Systems revisited in the context of Sarnak’s conjecture. Studia Math. 229(1) (2015), 45–72.Google Scholar
El Abdalaoui, E. H., Przymus, J. K., Lemańczyk, M. and de la Rue, T.. The Chowla and the Sarnak conjectures from ergodic theory point of view. Discrete Contin. Dyn. Syst. 37(6) (2017), 2899–2944.10.3934/dcds.2017125CrossRefGoogle Scholar
El Abdalaoui, E. H., Kasjan, S. and Lemańczyk, M.. 0-1 sequences of the Thue-Morse type and Sarnak’s conjecture. Proc. Amer. Math. Soc. 144(1) (2016), 161–176.CrossRefGoogle Scholar
Frantzikinakis, N. and Host, B.. Furstenberg systems of bounded multiplicative functions and applications. Int. Math. Res. Not. doi:https://doi.org/10.1093/imrn/rnz037. Published online 21 February 2019.CrossRefGoogle Scholar
Frantzikinakis, N. and Host, B.. The logarithmic Sarnak conjecture for ergodic weights. Ann. of Math. (2) 187(3) (2018), 869–931.CrossRefGoogle Scholar
Fan, A.-H. and Jiang, Y.. Oscillating sequences, MMA and MMLS flows and Sarnak’s conjecture. Ergod. Th. & Dynam. Sys. 38(5) (2018), 1709–1744.CrossRefGoogle Scholar
Furstenberg, H., Katznelson, Y. and Ornstein, D.. The ergodic theoretical proof of Szemerédi’s theorem. Bull. Amer. Math. Soc. (N.S.) 7(3) (1982), 527–552.CrossRefGoogle Scholar
Frantzikinakis, N.. Ergodicity of the Liouville system implies the Chowla conjecture. Discrete Anal. 41(19) (2017).Google Scholar
Gomilko, A., Lemańczyk, M. and de La Rue, T.. Möbius orthogonality in density for zero entropy dynamical systems. Preprint, 2019, arXiv:https://arxiv.org/abs/1905.06563.Google Scholar
Green, B. and Tao, T.. Linear equations in primes. Ann. of Math (2) 171(3) (2010), 1753–1850.CrossRefGoogle Scholar
Green, B. and Tao, T.. The Möbius function is strongly orthogonal to nilsequences. Ann. of Math. (2) 175(2) (2012), 541–566.CrossRefGoogle Scholar
Green, B. and Tao, T.. The quantitative behaviour of polynomial orbits on nilmanifolds. Ann. of Math. 175 (2012), 465–540.CrossRefGoogle Scholar
Green, B., Tao, T. and Ziegler, T.. An inverse theorem for the Gowers ${U}^{s+1}\left[N\right]$ -norm. Ann. of Math. (2) 176(2) (2012), 1231–1372.CrossRefGoogle Scholar
Hildebrand, A.. On consecutive values of the Liouville function. Enseign. Math. (2) 32(3–4) (1986), 219–226.Google Scholar
Host, B. and Kra, B.. Nonconventional ergodic averages and nilmanifolds. Ann. of Math. (2) 161(1) (2005), 397–488.CrossRefGoogle Scholar
Host, B. and Kra, B.. Nilpotent Structures in Ergodic Theory (Mathematical Surveys and Monographs, 236), American Mathematical Society, Providence, RI, 2018.Google Scholar
Huang, W., Lian, Z., Shao, S. and Ye, X.. Sequences from zero entropy noncommutative toral automorphisms and Sarnak conjecture. J. Differential Equations 263(1) (2017), 779–810.CrossRefGoogle Scholar
Liu, J. and Sarnak, P.. The Möbius function and distal flows. Duke Math. J. 164(7) (2015), 1353–1399.10.1215/00127094-2916213CrossRefGoogle Scholar
Martin, B., Mauduit, C. and Rivat, J.. Théoréme des nombres premiers pour les fonctions digitales. Acta Arith. 165(1) (2014), 11–45.10.4064/aa165-1-2CrossRefGoogle Scholar
Moreira, J.. Tao’s proof of (logarithmically averaged) Chowla’s conjecture for two point correlations (2018), available at https://joelmoreira.wordpress.com/2018/11/04/taos-proof-of-logarithmically-averaged-chowlas-conjecture-for-two-point-correlations/.Google Scholar
Matomäki, K. and Radziwiłł, M.. Multiplicative functions in short intervals. Ann. of Math. (2) 183(3) (2016), 1015–1056.10.4007/annals.2016.183.3.6CrossRefGoogle Scholar
Matomäki, K., Radziwiłł, M. and Tao, T.. An averaged form of Chowla’s conjecture. Algebra Number Theory 9(9) (2015), 2167–2196.CrossRefGoogle Scholar
Matomäki, K., Radziwiłł, M. and Tao, T.. Sign patterns of the Liouville and Möbius functions. Forum Math. Sigma 4 (2016), e14, 44.CrossRefGoogle Scholar
Matomäki, K., Radziwiłł, M. and Tao, T.. Fourier uniformity of bounded multiplicative functions in short intervals on average. Invent. Math. 220 (2020), 59.CrossRefGoogle Scholar
Müllner, C.. Automatic sequences fulfill the Sarnak conjecture. Duke Math. J. 166(17) (2017), 3219–3290.10.1215/00127094-2017-0024CrossRefGoogle Scholar
Peckner, R.. Möbius disjointness for homogeneous dynamics. Duke Math. J. 167(14) (2018), 2745–2792.CrossRefGoogle Scholar
Sarnak, P.. Mobius randomness and dynamics. Not. S. Afr. Math. Soc. 43(2) (2012), 89–97.Google Scholar
Sawin, W.. Dynamical models for Liouville and obstructions to further progress on sign patters. J. Number Theory 213 (2020), 1–15.CrossRefGoogle Scholar
Tao, T.. The logarithmically averaged Chowla and Elliott conjectures for two-point correlations. Forum Math. Pi 4 (2016), e8, 36.10.1017/fmp.2016.6CrossRefGoogle Scholar
Tao, T.. Equivalence of the logarithmically averaged Chowla and Sarnak conjectures. Number Theory—Diophantine Problems, Uniform Distribution and Applications. Springer, Cham, 2017, pp. 391–421.CrossRefGoogle Scholar
Tao, T.. Furstenberg limits of the Liouville function (2017), available at https://terrytao.wordpress.com/2017/03/05/furstenberg-limits-of-the-liouville-function/.Google Scholar
Tao, T. and Teräväinen, J.. Odd order cases of the logarithmically averaged Chowla conjecture. J. Théor. Nombres Bordeaux 30 (2018), 997–1015, available at https://jtnb.centre-mersenne.org/article/JTNB_2018__30_3_997_0.pdf.CrossRefGoogle Scholar
Tao, T. and Teräväinen, J.. The structure of logarithmically averaged correlations of multiplicative functions, with applications to the Chowla and Elliott conjectures. Duke Math. J. 168 (2019), 1977–2027.CrossRefGoogle Scholar
Veech, W. A.. Möbius orthogonality for generalized Morse-Kakutani flows. Amer. J. Math. 139(5) (2017), 1157–1203.CrossRefGoogle Scholar
Wang, Z.. Möbius disjointness for analytic skew products. Invent. Math. 209(1) (2017), 175–196.CrossRefGoogle Scholar