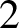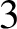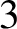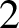Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Geiges, Hansjörg
2022.
What does a vector field know about volume?.
Journal of Fixed Point Theory and Applications,
Vol. 24,
Issue. 2,
Cristofaro-Gardiner, Daniel
Hryniewicz, Umberto
Hutchings, Michael
and
Liu, Hui
2023.
Contact three-manifolds with exactly two simple Reeb orbits.
Geometry & Topology,
Vol. 27,
Issue. 9,
p.
3801.