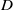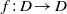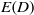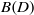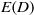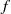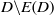Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Sun, Taixiang
Chen, Zhanhe
Liu, Xinhe
and
Xi, Hongjian
2014.
Equicontinuity of dendrite maps.
Chaos, Solitons & Fractals,
Vol. 69,
Issue. ,
p.
10.
Abdelrazak, Jmel
2015.
Pointwise periodic homeomorphisms on dendrites.
Dynamical Systems,
Vol. 30,
Issue. 1,
p.
34.
Hattab, Hawete
and
Salhi, Ezzeddine
2016.
Homeomorphisms of Locally Finite Graphs.
Qualitative Theory of Dynamical Systems,
Vol. 15,
Issue. 2,
p.
481.
Marzougui, Habib
and
Naghmouchi, Issam
2016.
Minimal sets for group actions on dendrites.
Proceedings of the American Mathematical Society,
Vol. 144,
Issue. 10,
p.
4413.
Abouda, Haithem
and
Naghmouchi, Issam
2016.
Monotone maps on dendrites and their induced maps.
Topology and its Applications,
Vol. 204,
Issue. ,
p.
121.
Sun, Tai Xiang
Su, Guang Wang
Xi, Hong Jian
and
Kong, Xin
2017.
Equicontinuity of maps on a dendrite with finite branch points.
Acta Mathematica Sinica, English Series,
Vol. 33,
Issue. 8,
p.
1125.
Haj Salem, Aymen
and
Hattab, Hawete
2017.
Dendrite Flows.
Qualitative Theory of Dynamical Systems,
Vol. 16,
Issue. 3,
p.
623.
BYSZEWSKI, JAKUB
FALNIOWSKI, FRYDERYK
and
KWIETNIAK, DOMINIK
2017.
Transitive dendrite map with zero entropy.
Ergodic Theory and Dynamical Systems,
Vol. 37,
Issue. 7,
p.
2077.
Sun, Taixiang
Tang, Yalin
Su, Guangwang
Xi, Hongjian
and
Qin, Bin
2018.
Special $$\alpha $$ α -Limit Points and $$\gamma $$ γ -Limit Points of a Dendrite Map.
Qualitative Theory of Dynamical Systems,
Vol. 17,
Issue. 1,
p.
245.
Sun, Taixiang
Su, Guangwang
and
Qin, Bin
2019.
The depths of the centres and the attracting centres of a class of dendrite maps.
Journal of Mathematical Analysis and Applications,
Vol. 479,
Issue. 1,
p.
1158.
Su, Guangwang
Sun, Taixiang
Han, Caihong
and
Qin, Bin
2020.
Characteristic of pointwise-recurrent maps on a dendrite.
Journal of Dynamical Systems and Geometric Theories,
Vol. 18,
Issue. 1,
p.
1.
Askri, Ghassen
and
Naghmouchi, Issam
2020.
Pointwise Recurrence on Local Dendrites.
Qualitative Theory of Dynamical Systems,
Vol. 19,
Issue. 1,
Hattab, Hawete
and
Ghareeb, A.
2022.
Recurrence and Chaos of Local Dendrite Maps.
Journal of Mathematics,
Vol. 2022,
Issue. 1,
Su, Guang Wang
Han, Cai Hong
Sun, Tai Xiang
and
Li, Lue
2022.
The Depths and the Attracting Centres for Continuous Maps on a Dendrite Whose Rank is Finite.
Acta Mathematica Sinica, English Series,
Vol. 38,
Issue. 9,
p.
1643.
FORYŚ-KRAWIEC, MAGDALENA
HANTÁKOVÁ, JANA
KUPKA, JIŘÍ
OPROCHA, PIOTR
and
ROTH, SAMUEL
2023.
Dendrites and measures with discrete spectrum.
Ergodic Theory and Dynamical Systems,
Vol. 43,
Issue. 2,
p.
545.