Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-26T01:07:49.225Z Has data issue: false hasContentIssue false
  • English
  • Français

On interpreting Patterson–Sullivan measures of geometrically finite groups as Hausdorff and packing measures

Published online by Cambridge University Press:  21 July 2015

DAVID SIMMONS
DAVID SIMMONS*
Affiliation:
Ohio State University, Department of Mathematics, 231 W. 18th Avenue, Columbus, OH 43210-1174, USA email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We provide a new proof of a theorem whose proof was sketched by Sullivan [Disjoint spheres, approximation by imaginary quadratic numbers, and the logarithm law for geodesics. Acta Math.149(3–4) (1982), 215–237], namely that if the Poincaré exponent of a geometrically finite Kleinian group 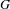$G$ is strictly between its minimal and maximal cusp ranks, then the Patterson–Sullivan measure of $G$ is not proportional to the Hausdorff or packing measure of any gauge function. This disproves a conjecture of Stratmann [Multiple fractal aspects of conformal measures; a survey. Workshop on Fractals and Dynamics (Mathematica Gottingensis, 5). Eds. M. Denker, S.-M. Heinemann and B. Stratmann. Springer, Berlin, 1997, pp. 65–71; Fractal geometry on hyperbolic manifolds. Non-Euclidean Geometries (Mathematical Applications (N.Y.), 581). Springer, New York, 2006, pp. 227–247].

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 36 , Issue 8 , December 2016 , pp. 2675 - 2686
Copyright
© Cambridge University Press, 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ala-Mattila, V.. Geometric characterizations for Patterson–Sullivan measures of geometrically finite Kleinian groups. Ann. Acad. Sci. Fenn. Math. Diss. 157 (2011).Google Scholar
Bishop, C. J. and Jones, P. W.. The law of the iterated logarithm for Kleinian groups. Lipa’s Legacy (New York, 1995) (Contemporary Mathematics, 211) . American Mathematical Society, Providence, RI, 1997, pp. 17–50.Google Scholar
Bishop, C. J. and Peres, Y.. Fractal sets in probability and analysis, Preprint, http://www.math.washington.edu/∼solomyak/TEACH/582/12/BishopPeres.pdf.Google Scholar
Bowditch, B. H.. Geometrical finiteness for hyperbolic groups. J. Funct. Anal. 113(2) (1993), 245–317.Google Scholar
Hersonsky, S. D. and Paulin, F.. Counting orbit points in coverings of negatively curved manifolds and Hausdorff dimension of cusp excursions. Ergod. Th. & Dynam. Sys. 24(3) (2004), 803–824.Google Scholar
Mauldin, R. D., Szarek, T. and Urbański, M.. Graph directed Markov systems on Hilbert spaces. Math. Proc. Cambridge Philos. Soc. 147 (2009), 455–488.Google Scholar
Rogers, C. A. and Taylor, S. J.. The analysis of additive set functions in Euclidean space. Acta Math. 101 (1959), 273–302.Google Scholar
Schapira, B.. Lemme de l’ombre et non divergence des horosphères d’une variété géométriquement finie [The shadow lemma and non-divergence of the horospheres of a geometrically finite manifold]. Ann. Inst. Fourier (Grenoble) 54 (2004), 939–987 (in French).Google Scholar
Stratmann, B. O.. Multiple fractal aspects of conformal measures; a survey. Workshop on Fractals and Dynamics (Mathematica Gottingensis, 5) . Eds. Denker, M., Heinemann, S.-M. and Stratmann, B.. Springer, Berlin, 1997, pp. 65–71.Google Scholar
Stratmann, B. O.. Fractal geometry on hyperbolic manifolds. Non-Euclidean Geometries (Mathematical Applications (N.Y.), 581) . Springer, New York, 2006, pp. 227–247.Google Scholar
Stratmann, B. O. and Urbański, M.. The box-counting dimension for geometrically finite Kleinian groups. Fund. Math. 149(1) (1996), 83–93.Google Scholar
Stratmann, B. O. and Velani, S. L.. The Patterson measure for geometrically finite groups with parabolic elements, new and old. Proc. Lond. Math. Soc. (3) 71(1) (1995), 197–220.Google Scholar
Sullivan, D. P.. Discrete conformal groups and measurable dynamics. Bull. Amer. Math. Soc. (N.S.) 6(1) (1982), 57–73.Google Scholar
Sullivan, D. P.. Disjoint spheres, approximation by imaginary quadratic numbers, and the logarithm law for geodesics. Acta Math. 149(3–4) (1982), 215–237.Google Scholar
Sullivan, D. P.. Entropy, Hausdorff measures old and new, and limit sets of geometrically finite Kleinian groups. Acta Math. 153(3–4) (1984), 259–277.Google Scholar
Taylor, S. J. and Tricot, C.. Packing measure, and its evaluation for a Brownian path. Trans. Amer. Math. Soc. 288(2) (1985), 679–699.Google Scholar