Published online by Cambridge University Press: 19 September 2008
Let  be an irreducible 0–1 matrix such that the non-zero entries in each row are consecutive. Let
be an irreducible 0–1 matrix such that the non-zero entries in each row are consecutive. Let  be the class of piecewise linear Markov transformations τ on [0, 1] into [0, 1] induced by
be the class of piecewise linear Markov transformations τ on [0, 1] into [0, 1] induced by  for which the absolutely continuous invariant measure has maximal entropy. The main result presents necessary and sufficient slope conditions on τ which guarantee that τ ∈
for which the absolutely continuous invariant measure has maximal entropy. The main result presents necessary and sufficient slope conditions on τ which guarantee that τ ∈ 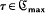 .
.