Hostname: page-component-586b7cd67f-2plfb Total loading time: 0 Render date: 2024-12-01T02:50:46.095Z Has data issue: false hasContentIssue false
  • English
  • Français

Isospectral sets for boundary value problems on the unit interval

Published online by Cambridge University Press:  10 December 2009

Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

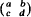We analyse isospectral sets of potentials associated to a given ‘generalized periodic’ boundary condition in SL(2, R) for the Sturm-Liouville equation on the unit interval. This is done by first studying the larger manifold M of all pairs of boundary conditions and potentials with a given spectrum and characterizing the critical points of the map from M to the trace a + d Isospectral sets appear as slices of M whose geometry is determined by the critical point structure of the trace function. This paper completes the classification of isospectral sets for all real self-adjoint boundary conditions.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 8 , Volume 8: Charles Conley Memorial Issue , December 1988 , pp. 301 - 358
Copyright
Copyright © Cambridge University Press 1988

Footnotes

†

With an appendix by Johan Tysk, Department of Mathematics, University of California, Los Angeles, CA 90024, USA.

References

REFERENCES

Dahlberg, B. E. J. & Trubowitz, E.. The inverse Sturm-Liouville problem III. Commun. Pure Appl. Math. 37 (1984), 255–268.Google Scholar
Garnett, J. & Trubowitz, E.. Gaps and bands of one-dimensional periodic Schrödinger operators. Commun. Math. Helv. 59 (1984), 258–312.Google Scholar
Isaacson, E., McKean, H. & Trubowitz, E.. The inverse Sturm-Liouville problem II. Commun. Pure Appl. Math. 37 (1984), 1–12.Google Scholar
Isaacson, E. & Trubowitz, E.. The inverse Sturm-Liouville problem I. Commun. Pure Appl. Math. 36 (1983), 767–783.Google Scholar
Trubowitz, E.. The inverse problem for periodic potentials. Commun. Pure Appl. Math. 30 (1977), 321–337.Google Scholar
Pöschel, J. & Trubowitz, E.. Inverse Spectral Theory. Academic Press (1987).Google Scholar