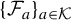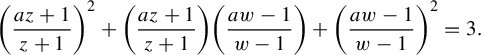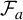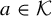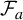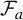1 Introduction
In 1965, Brolin [Reference Brolin3] studied asymptotic properties of polynomials
$P(z)\in \mathbb {C}[z]$
of degree bigger than or equal to
$2$
. He proved the existence of a probability measure for which the preimages
$P^{-n}(z_0)$
at time n of any point
$z_0\in \mathbb {C}$
(with at most one exception) asymptotically equidistribute, as n tends to infinity. In 1983, Freire, Lopes, and Mañé [Reference Freire, Lopes and Mañé20] and Ljubich [Reference Ljubich24] independently proved the generalization to rational maps of degree at least
$2$
on the Riemann sphere. These results have been generalized to different settings. For instance, see [Reference Dinh, Sibony, Gentili, Guenot and Patrizio18, §1.4] and the references therein for higher dimensions, and [Reference Favre and Rivera-Letelier19, Reference Gignac22] for the non-Archimedian setting.
The equidistribution properties of holomorphic correspondences have also attracted considerable interest. Roughly speaking, a holomorphic correspondence on a complex manifold X is a multivalued map induced by a formal sum
$\Gamma =\sum n_i\Gamma (i)$
of complex varieties
$\Gamma (i)\subset X\times X$
of the same dimension. The multivalued map sends z to w if
$(z,w)$
belongs to some
$\Gamma (i)$
(see §2.1). Let
$d(F)$
denote the number of pre-images of a generic point under F. We call this number the topological degree of F, just as in the case of rational maps. We study the existence of a Borel probability measure
$\mu $
on X that has the property that for all but at most finitely many
$z_0\in X$
,
$$ \begin{align*} \frac{1}{d(F)^n}(F^n)_*\delta_{z_0}\to\mu, \end{align*} $$
as
$n\to \infty $
. Here,
$(F^n)_*$
denotes the push-forward operator associated to
$F^n$
. In [Reference Dinh13], Dinh studied the case of polynomial correspondences whose Lojasiewicz exponent is strictly bigger than
$1$
, in which case we always have that
$d(F^{-1})<d(F)$
. The case where
$d(F)=d(F^{-1})$
is open but some subcases are known. For instance, Clozel, Oh, and Ullmo [Reference Clozel, Oh and Ullmo9] proved equidistribution for irreducible modular correspondences, Clozel and Otal [Reference Clozel and Otal10] proved it for exterior modular correspondences, and Clozel and Ullmo [Reference Clozel and Ullmo11] for those that are self-adjoint. On the other hand, Dinh, Kaufmann, and Wu proved in [Reference Dinh, Kaufmann and Wu15] that if such F is not weakly modular, then the statement holds for both F and
$F^{-1}$
. Modular correspondences are weakly modular, but the reverse containment does not hold. On a different classification, Bharali and Sridharan [Reference Bharali and Sridharan1] proved equidistribution for correspondences with
$d(F)\geq d(F^{-1})$
having a repeller in the sense of [Reference McGehee26]. We will study a
$1$
-parameter family in the gap between weak-modularity and modularity, and for which the result in [Reference Bharali and Sridharan1] does not apply.
Our object of study is the family of correspondences
$\lbrace \mathcal {F}_a\rbrace _{a\in \mathcal {K}}$
on the Riemann sphere
$\widehat {\mathbb {C}}$
, where
$\mathcal {F}_a$
is given in affine coordinates by
$$ \begin{align} \bigg(\frac{az+1}{z+1}\bigg)^2+\bigg(\frac{az+1}{z+1}\bigg)\bigg(\frac{aw-1}{w-1}\bigg)+\bigg(\frac{aw-1}{w-1}\bigg)^2=3, \end{align} $$
and
$\mathcal {K}$
is the Klein combination locus defined in §2.2. This family was studied by Bullett et al in [Reference Bullett and Lomonaco4–Reference Bullett and Penrose6, Reference Bullett and Harvey8]. In [Reference Bullett and Lomonaco4], Bullett and Lomonaco proved that there is a two sided restriction
$f_a$
of
$\mathcal {F}_a$
that is hybrid equivalent to a quadratic rational map P that has a fixed point with multiplier
$1$
. We refer the reader to [Reference Lomonaco25] for conjugacy of parabolic-like mappings. Moreover, for the parameters for which the Julia set of P is connected, we have that
$\mathcal {F}_a$
is a mating between the rational map P and the modular group
$\operatorname {PSL}_2(\mathbb {Z})$
. This generalizes a previous result by Bullett and Penrose [Reference Bullett and Penrose6]. The correspondence
$\mathcal {F}_a$
has two homeomorphic copies of the filled Julia set
$K$
of
$P_A$
, denoted
$\Lambda _{a,-}$
and
$\Lambda _{a,+}$
, and they satisfy that
$\mathcal {F}_a^{-1}(\Lambda _{a,-})=\Lambda _{a,-}$
and
$\mathcal {F}_a(\Lambda _{a,+})=\Lambda _{a,+}$
. These are called the backward and forward limit set, respectively (see §3.2).
The following theorem states that this family does not fit the conditions for any of the equidistribution results listed above (see §1.1).
Theorem 1.1. For every
$a\in \mathcal {K}$
, we have that:
-
(1)
$\mathcal {F}_a$
is a weakly modular correspondence that is not modular; and -
(2)
$\partial \Lambda _{a,-}$
is not a repeller for
$\mathcal {F}_a$
.
Furthermore, we prove that
$\mathcal {F}_a$
satisfies a property that is stronger than weak-modularity (see Remark 3.4).
The purpose of this paper is to show that equidistribution holds for the family
$\lbrace \mathcal {F}_a\rbrace _{a\in \mathcal {K}}$
. Put
We prove the following equidistribution theorem.
Theorem 1.2. Let
$a\in \mathcal {K}$
. There exist two Borel probability measures
$\mu _+$
and
$\mu _-$
on
$\widehat {\mathbb {C}}$
, with
$\operatorname {supp}(\mu _+)=\partial \Lambda _{a,+}$
and
$\operatorname {supp}(\mu _-)=\partial \Lambda _{a,-}$
, such that for every
$z_0\in \widehat {\mathbb {C}}\setminus \mathcal {E}_a$
,
$$ \begin{align*}\frac{1}{2^n}(\mathcal{F}_a^n)_*\delta_{z_0}\to\mu_+\quad\mbox{and}\quad\frac{1}{2^n}(\mathcal{F}_a^{-n})_*\delta_{z_0}\to\mu_-,\end{align*} $$
weakly, as
$n\to \infty $
.
In later work [Reference Matus de la Parra12], we prove that the measures
$\mu _+$
and
$\mu _-$
maximize entropy: the metric entropy in [Reference Vivas and Sirvent31] yields equality for the half-variational principle with the topological entropy in [Reference Dinh and Sibony17].
Let F be a holomorphic correspondence on X with graph
$\Gamma $
. Denote by
$\Gamma ^{(n)}$
the graph of
$F^n$
and by
the diagonal in
$X\times X$
. Then the set of periodic points of F of period n is defined as the set
and for
$z\in \operatorname {Per}_n(F)$
, we define the multiplicity of z as a periodic point of F of order n to be the number
, defined in §2.1.
Another source of motivation is whether or not periodic points equidistribute. In [Reference Ljubich24], Ljubich showed that this is the case for rational maps of degree bigger than or equal to
$2$
, where periodic points are counted either with or without multiplicity. The equidistribution of periodic points is also studied in [Reference Briend and Duval2, Reference Dinh, Nguyen and Truong16, Reference Favre and Rivera-Letelier19] in the case of maps, and in [Reference Dinh13, Reference Dinh14] in the case of correspondences. We prove this holds for the family
$\lbrace \mathcal {F}_a\rbrace _{a\in \mathcal {K}}$
as well.
Theorem 1.3. For
$a\in \mathcal {K}$
,
are both weakly convergent to
$\tfrac 12(\mu _-+\mu _+)$
, as
$n\to \infty $
.
In §5, we define the set
$\hat {P}^{\Gamma }_n$
of superstable parameters of order n. Combining the main results of [Reference Bullett and Lomonaco5, Reference Petersen and Roesch29], we obtain a homeomorphism
$\Psi :\mathcal {M}\to \mathcal {M}_{\Gamma }$
between the Mandelbrot set
$\mathcal {M}$
and the connectedness locus
$\mathcal {M}_{\Gamma }$
of the family
$\lbrace \mathcal {F}_a\rbrace _{a\in \mathcal {K}}$
. These results, together with the equidistribution result in [Reference Levin23], yield the following theorem.
Theorem 1.4. In
$\mathcal {M}_{\Gamma }$
, superstable parameters equidistribute with respect to
$\Psi ^*m_{\operatorname {BIF}}$
, that is,
$$ \begin{align*}\lim\limits_{n\to\infty}\frac{1}{2^{n-1}}\sum\limits_{a\in\hat{P}^{\Gamma}_n}\delta_a=\Psi^*m_{\operatorname{BIF}}.\end{align*} $$
1.1 Notes and references
There is a bigger family studied by Bullett and Harvey in [Reference Bullett and Harvey8], given by replacing the right-hand side of equation (1) by
$3k$
, where
$k\in \mathbb {C}$
. For these correspondences, it is also possible to define limit sets
$\Lambda _{a,k,-}$
and
$\Lambda _{a,k,+}$
analogous to those in the case where
$k=1$
. In [Reference Bharali and Sridharan1], Bharali and Sridharan show how their equidistribution result applies to these correspondences in the case where
$\Lambda _{a,k,-}$
is a repeller. Parameters for which this is the case exist from the results in [Reference Bullett and Harvey8]. However, we prove in Theorem 1.1 part
$(2)$
that this is never the case when
$k=1$
and
$a\in \mathcal {K}$
.
1.2 Organization
The structure of this paper is as follows. In §2.1, we give an introduction to holomorphic correspondences and their action on Borel measures. In §2.2, we introduce the correspondences
$\mathcal {F}_a$
given by equation (1). We define critical values and find those of
$\mathcal {F}_a$
in §2.3, and define Klein combination pair and the Klein combination locus
$\mathcal {K}$
in §2.4. In §3.1, we define modular and weakly modular correspondences, and prove part
$(1)$
of Theorem 1.1. To prove that
$\mathcal {F}_a$
is weakly modular, we use the decomposition
$\mathcal {F}_a=\operatorname {J}_a\circ \operatorname {Cov^Q_0}$
, given in [Reference Bullett and Lomonaco4], into a certain involution
$\operatorname {J}_a$
composed with the deleted covering correspondence
$\operatorname {Cov^Q_0}$
described in §2.2, and construct the measures in the definition of weakly modular using the symmetry of the graph of
$\operatorname {Cov^Q_0}$
. The fact that
$\mathcal {F}_a$
is not modular follows from the fact that Borel measures assigning positive measure to non-empty open sets are not invariant by
$\mathcal {F}_a$
. In §3.2, we define the limit sets
$\Lambda _{a,-}$
and
$\Lambda _{a,+}$
, and prove part
$(2)$
of Theorem 1.1 by showing that the parabolic fixed point in
$\partial \Lambda _{a,-}$
violates the definition of a repeller. In §4, we describe the exceptional set of the two-sided restriction
$f_a$
and the set
$\operatorname {Per}_n(\mathcal {F}_a)$
of periodic points of period n. Finally, in §5, we use the description of
$f_a$
given in [Reference Bullett and Lomonaco4] together with the results in [Reference Freire, Lopes and Mañé20, Reference Ljubich24] to prove Theorems 1.2 and 1.3 about asymptotic equidistribution of images, preimages, and periodic points. We finish with the proof of Theorem 1.4 about equidistribution of special points in the modular Mandelbrot set
$\mathcal {M}_{\Gamma }$
.
2 Preliminaries
2.1 Holomorphic correspondences
Let X be a compact Riemann surface and let
$\pi _j:X\times X\to X$
be the canonical projection to the jth coordinate,
$j=1,2$
. We say that a formal sum
$\Gamma =\sum _i n_i\Gamma (i)$
is a holomorphic 1-chain on
$X\times X$
if its support
is a subvariety of
$X\times X$
of pure dimension
$1$
whose irreducible components are exactly the
$\Gamma (i)$
, and the
$n_i$
are non-negative integers. We say that the
$\Gamma (i)$
are the irreducible components of
$\Gamma $
.
Let
$\Gamma =\sum _in_i\Gamma (i)$
be a holomorphic 1-chain satisfying that for
$j=1,2$
and every i such that
$n_i>0$
, the restriction
of the canonical projection
$X\times X\to X$
to the irreducible component
$\Gamma (i)$
is surjective. The chain
$\Gamma $
induces a multivalued map F from X to itself by
The multivalued map F is called a holomorphic correspondence and it is said to be irreducible if
$\sum _i n_i=1$
. We say that
is the graph of the holomorphic correspondence F. Let
$\iota :X\times X\to X\times X$
be the involution
$(z,w)\mapsto (w,z)$
. We can define the adjoint correspondence
$F^{-1}$
of F by the relation
, which is a holomorphic correspondence, whose graph is the holomorphic 1-chain
$\Gamma _F^{-1}=\sum _in_i\iota (\Gamma (i))$
.
In [Reference Stoll30], Stoll introduced a notion of multiplicity that will be useful for this paper. Let M be a quasi-projective variety and N a smooth quasi-projective variety. If
$g:M\to N$
is regular, and
$a\in M$
, then we say that a neighborhood U of a is distinguished with respect to g and a if
$\overline {U}$
is compact and
$g^{-1}(g(a))\cap \overline {U}=\lbrace a\rbrace $
. Such neighborhoods exist if and only if
$\dim _a g^{-1}(g(a))=0$
and, in this case, they form a base of neighborhoods. If U is distinguished with respect to g and a, then put
. It can be shown that
does not depend on the distinguished neighborhood U, and the maps
$n_b$
defined on N by
$a\mapsto \sum \nolimits _{b\in g^{-1}(a)}\hat {\nu }_g(b)$
are constant in each component.
Suppose that
$g:M\to N$
is a finite and surjective regular map, with M and N as above. Stoll proved in [Reference Stoll30] that
$\hat {\nu }_g(a)$
generalizes the notion of multiplicity of g at a and whenever
$\varphi $
is a continuous function with compact support in M, the map
$$ \begin{align*}a\mapsto\sum\limits_{b\in g^{-1}(a)}\hat{\nu}_g(b)\varphi(b)\end{align*} $$
is continuous.
To study dynamics, we proceed to define the composition of two holomorphic correspondences F and G with associated holomorphic 1-chains
$\Gamma _F=\sum _i n_i\Gamma _F(i)$
and
$\Gamma _G=\sum _j m_j\Gamma _G(j)$
, respectively. For each i and j, let
$A_{i,j}$
be the image of the projection
$p_{i,j}:(\Gamma _G(j)\times \Gamma _F(i))\cap \lbrace x_2=x_3\rbrace \hookrightarrow X\times X$
that forgets the second and third coordinates, i.e.,
Let
$\lbrace \Gamma (i,j,k)\rbrace _{k=1}^{N(i,j)}$
be the irreducible components of
$A_{i,j}$
. Observe that since
$\Gamma _G(j)$
and
$\Gamma _F(i)$
are both quasi-projective, and so is
$\lbrace x_2=x_4\rbrace \subset X^4$
, then
$p_{i,j}$
is a regular map from the quasi-projective variety
$(\Gamma _G(j)\times \Gamma _F(i))\cap \lbrace x_2=x_3\rbrace $
to the smooth quasi-projective variety
$X\times X$
. Then we have that the map
defined on
$\Gamma (i,j,k)$
is constant. Therefore,
denotes the number of
$x\in X$
such that
$((z,x),(x,w))\in \Gamma _G(j)\times \Gamma _F(i)$
, for a generic point
$(z,w)\in \Gamma (i,j,k)$
. Define the composition
$F\circ G$
as the holomorphic correspondence determined by the holomorphic 1-chain
Note that the
$\operatorname {supp}\Gamma _{F\circ G}=\bigcup _{i,j}A_{i,j}$
.
Set
. We have that
$d(F\circ G)=d(F)d(G)$
. Thus, in particular, for every integer
$n\geq 1$
, we have that
$d(F^n)=(d(F))^n$
. We call
$d(F)$
the topological degree of F, and it corresponds to the number of preimages of a generic point under F.
If F is an irreducible holomorphic correspondence over X with graph
$\Gamma $
and
$\varphi :X\to \mathbb {C}$
is a continuous function, then
is continuous as well, see [Reference Clozel and Ullmo11, Lemma 1.1]. Now let F be a holomorphic correspondence that is not necessarily irreducible, with graph
$\Gamma _F=\sum _i n_i\Gamma (i)$
. We denote by
$F_i$
the holomorphic correspondence induced by
$\Gamma (i)$
and we put
and
. Then for every continuous function
$\varphi :X\to \mathbb {C}$
, the map
$$ \begin{align*} z\mapsto\sum\limits_{w\in F(z)}\nu_{F}(z,w)\varphi(w)&=\sum\limits_{w\in f(z)}\bigg(\sum\limits_i n_i\nu_{F_i}(z,w)\bigg)\varphi(w)\\ &=\sum\limits_i n_i {F_i}_*\varphi(w) \end{align*} $$
is also continuous.
The holomorphic correspondence F induces an action
$F_*$
on finite Borel measures
$\mu $
by duality, namely
, called the push-forward operator and the resultant measure
$F_*\mu $
is the push-forward measure of
$\mu $
under F. We define as well the action
, called the pull-back operator and the resultant measure
$F^*\mu $
is called the pull-back measure of
$\mu $
under F. This action on measures agrees with the action on points
where
$\delta _z$
is the Dirac delta at z.
To see this, note that for every continuous function
$\varphi :X\to \mathbb {C}$
,
$$ \begin{align*} \bigg\langle\sum\limits_{w\in F(z)}\nu_{F}(z,w)\delta_w,\varphi\bigg\rangle&=\sum\limits_{w\in F(z)}\nu_{F}(z,w)\langle\delta_w,\varphi\rangle\\ &=\sum\limits_{w\in F(z)}\nu_{F}(z,w)\varphi(w)\\ &=\int\bigg(\sum\limits_{w\in F(\zeta)}\nu_{F}(\zeta,w)\varphi(w)\bigg)d\delta_z(\zeta)\\ &=\langle\delta_z,F_*\varphi\rangle\\ &=\langle F^*\delta_z,\varphi\rangle. \end{align*} $$
2.2 The family
$\lbrace \mathcal {F}_a\rbrace _a$
Let
$Q(z)\in \mathbb {C}[z]$
be a nonlinear polynomial. The deleted covering relation of Q on
$\mathbb {C}\times \mathbb {C}$
is defined by
$w\in \operatorname {Cov^Q_0}(z)$
if and only if
Note that the denominator ‘deletes’ the obvious association of z with itself in the equation
$Q(z)=Q(w)$
.
In this section, we will identify
$\widehat {\mathbb {C}}$
with the complex projective line when it is convenient to work with homogeneous coordinates
$(z:w)$
.
Proposition 2.1. Put
. The closure of the relation in equation (2) is an irreducible quasiprojective complex variety
$\Gamma _0$
of
$\widehat {\mathbb {C}}\times \widehat {\mathbb {C}}$
of dimension
$1$
. Moreover, the projections
and
are both surjective and of degree
$2$
.
Proof. Note that
$P_Q(z,w)=z^2+zw+w^2-3$
and consider
$P_Q(z,w)$
as a single variable polynomial in
$(\mathbb {C}[z])[w]$
. Then its discriminant
$-3z^2+12$
is not a square in
$\mathbb {C}[z]$
. Therefore,
$P_Q(z,w)$
is an irreducible polynomial, and hence
is an irreducible subvariety of
$\mathbb {C}\times \mathbb {C}$
.
Now we want to describe the closure
$\overline {\mathcal {Z}}$
of
$\mathcal {Z}$
in
$\widehat {\mathbb {C}}\times \widehat {\mathbb {C}}$
. Observe that if we fix
$z\in \mathbb {C}$
, then
$\lim \nolimits _{w\to \infty }P_Q(z,w)=\infty $
, and if we fix
$w\in \mathbb {C}$
, then
$\lim \nolimits _{z\to \infty }P_Q(z,w)=\infty $
. Therefore, there are no points of the form
$(z,\infty )$
or
$(\infty , w)$
in
$\overline {\mathcal {Z}}$
. Given
$R>0$
, let
$z\in \mathbb {C}$
be such that
$|z|=R$
. Observe that
$P_Q(z,\cdot )\in \mathbb {C}[w]$
is non-constant and therefore has at least one root in
$\mathbb {C}$
. Let
$w\in \mathbb {C}$
be a root. Then
$(z,w)\in \mathcal {Z}$
and we have that
$|w^3-3w|=|Q(w)|=|Q(z)|=|z^3-3z|\geq R^3-3R$
. By taking
$R\to \infty $
, we get that
$(z,w)\to (\infty ,\infty )$
. Therefore,
$\overline {\mathcal {Z}}=\mathcal {Z}\cup \lbrace (\infty ,\infty )\rbrace $
. In particular,
$\overline {\mathcal {Z}}$
extends the relation given by equation (2) from
$\mathbb {C}\times \mathbb {C}$
to
$\widehat {\mathbb {C}}\times \widehat {\mathbb {C}}$
.
Take the homogenization
of
$P_Q(z,w)$
, so
$P_Q(z,w)=T(z,1,w,1)$
, and note that
$T(\unicode{x3bb} _1z,\unicode{x3bb} _1x,\unicode{x3bb} _2w,\unicode{x3bb} _2y)=\unicode{x3bb} _1^2\unicode{x3bb} _2^2T(z,x,w,y)$
. Thus, for the closed subvariety
of
$\widehat {\mathbb {C}}\times \widehat {\mathbb {C}}$
, we have that
$\Gamma _0=\overline {\mathcal {Z}}$
. To prove that
$\Gamma _0$
is irreducible, note that each of its irreducible components intersecting
$\mathbb {C}\times \mathbb {C}$
must be a closed subset of
$\Gamma _0$
containing
$\Gamma _0\cap (\mathbb {C}\times \mathbb {C})=\mathcal {Z}$
, and therefore it is
$\Gamma _0$
itself. Thus,
$\Gamma _0$
has only one irreducible component and hence it is irreducible.
We proceed to show
$\Gamma _0$
has dimension 1. Observe that the polynomial
$T(z,x,w,y)$
is irreducible in
$\mathbb {C}[z,x,w,y]$
, as whenever
$S(z,x,w,y)|T(z,x,w,y)$
in
$\mathbb {C}[z,x,w,y]$
, then
$S(z,1,w,1)|P_Q(z,w)$
in
$\mathbb {C}[z,w]$
. Therefore, the zero set
$Z(T)\subset \mathbb {C}^2\times \mathbb {C}^2$
of T is an irreducible hypersurface of
$\mathbb {C}^2\times \mathbb {C}^2$
, and hence it has codimension 1. Now let
$p:\mathbb {C}^2\setminus \lbrace (0,0)\rbrace \to \widehat {\mathbb {C}}$
be the projection sending
$(z,x)\mapsto (z:x)$
. Note that in the chart
$$ \begin{align*}U_1=\lbrace (z,w)\in\mathbb{C}^2\setminus\lbrace (0,0)\rbrace|w\neq 0\rbrace,\end{align*} $$
the map p is simply
$(z,w)\mapsto (z/w:1)$
, and in the chart
$$ \begin{align*}U_2=\lbrace (z,w)\in\mathbb{C}^2\setminus\lbrace (0,0)\rbrace|z\neq 0\rbrace,\end{align*} $$
it becomes
$(z,w)\mapsto (1:w/z)$
. Let
$\hat {p}:(\mathbb {C}^2\setminus \lbrace (0,0)\rbrace )\times (\mathbb {C}^2\setminus \lbrace (0,0)\rbrace )\to \widehat {\mathbb {C}}\times \widehat {\mathbb {C}}$
be the map defined by
. Then
is a regular map between irreducible varieties, and
has constant fiber dimension equal to 2, as T is homogeneous in
$(z,x)$
and in
$(w,y)$
. Therefore,
Finally, observe that the polynomial equation (2) has at least one and at most two solutions for every
$z\in \mathbb {C}$
, and by symmetry, the same holds for
$w\in \mathbb {C}$
. Note as well that
$\infty $
is in correspondence with and only with itself. Thus, the projections
and
are both surjective. Moreover,
$P_Q(1,1)=P_Q(1,-2)=0$
, and hence
$P_Q(1,w)$
has exactly two solutions. Therefore,
.
Remark 2.2. Proposition 2.1 holds for a large class of polynomials
$Q(z)$
. Observe that no polynomial can have
$Q(z)=Q(\infty )$
for a finite number z. Therefore, following the proof of Proposition 2.1, we conclude that to get an irreducible holomorphic correspondence, it suffices to prove that
$P_Q(z,w)$
is irreducible over
$\mathbb {C}$
. This holds under fairly general conditions. For instance, this is the case when Q is indecomposable and not linearly related to either
$z^n$
or a Chebyshev polynomial (see [Reference Fried21]).
On the other hand, note that whenever
$Q=R\circ S$
with R and S of degree greater than
$1$
, then
$P_S(z,w)$
divides
$P_Q(z,w)$
, and therefore
$P_Q(z,w)$
is reducible.
Proposition 2.1 says that
$\Gamma _0$
is the graph of an irreducible holomorphic correspondence, where
$\Gamma _0$
is a quasi-projective variety and
is a finite and surjective morphism over
$\mathbb {C}$
, and hence we can use our definition of pull-back and push-forward operators induced by the correspondence on finite measures. We call this correspondence the deleted covering correspondence of Q, denoted by
$\operatorname {Cov^Q_0}$
as well. That is,
$\operatorname {Cov^Q_0}$
is the holomorphic correspondence on
$\widehat {\mathbb {C}}\times \widehat {\mathbb {C}}$
such that
$\Gamma _{\operatorname {Cov^Q_0}}=\Gamma _0$
. From now on, we always consider
$Q(z)=z^3-3z$
.
Now take
$a\in \mathbb {C}\setminus \lbrace 1\rbrace $
and let
$\operatorname {J}_a:\widehat {\mathbb {C}}\to \widehat {\mathbb {C}}$
be the involution
The composition of
$\operatorname {Cov^Q_0}$
with the involution
$\operatorname {J}_a$
is again an irreducible holomorphic correspondence
on
$\widehat {\mathbb {C}}\times \widehat {\mathbb {C}}$
with graph
$\Gamma _a$
for which we can use the pull-back and push-forward operators above as well. Note that
$d(\mathcal {F}_a)=d(\mathcal {F}_a^{-1})=2$
and
$\mathcal {F}_a^{-1}=\operatorname {Cov^Q_0}\circ \operatorname {J}_a=\operatorname {J}_a\circ \mathcal {F}_a\circ \operatorname {J}_a$
, since
$\operatorname {Cov^Q_0}^{-1}=\operatorname {Cov^Q_0}$
and
$\operatorname {J}_a^{-1}=\operatorname {J}_a$
.
Set
. Then
$\phi _a^{-1}\circ \mathcal {F}_a\circ \phi _a$
is a holomorphic correspondence on
$\widehat {\mathbb {C}}\times \widehat {\mathbb {C}}$
that, restricted to
$(\widehat {\mathbb {C}}\setminus \lbrace -1\rbrace )\times (\widehat {\mathbb {C}}\setminus \lbrace 1\rbrace )$
, induces the relation given by equation (1). Thus,
$(z,w)\in (\widehat {\mathbb {C}}\setminus \lbrace -1\rbrace )\times (\widehat {\mathbb {C}}\setminus \lbrace 1\rbrace )$
satisfies equation (1) if and only if
$w\in \mathcal {F}_a(z)$
[Reference Bullett and Lomonaco4, Lemma 3.1].
Observe that
$\mathcal {F}_a^{-1}(1)=\operatorname {Cov^Q_0}(\operatorname {J}_a(1))=\operatorname {Cov^Q_0}(1)=\lbrace 1,-2\rbrace $
, independent of
$a\in \mathbb {C}\setminus \lbrace 1\rbrace $
. In particular,
$1\in \operatorname {Per}_1(\mathcal {F}_a)$
and we say that
$1$
is a fixed point of
$\mathcal {F}_a$
.
2.3 Critical values
In this section, we will discuss what parts of the graph of
$\mathcal {F}_a$
are locally the graph of a holomorphic function, by defining and finding all critical values and ramification points of
$\Gamma _a$
. This will be used in §4 to find the exceptional set.
Definition 2.3. Let
$\Gamma $
be the graph of an irreducible holomorphic correspondence on
$\widehat {\mathbb {C}}$
, and put
for
$j=1,2$
and
We extend the definition to holomorphic 1-chains
$\Gamma =\sum _i n_i\Gamma (i)$
by
We call
$A_2(\Gamma )$
the set of ramification points of the holomorphic correspondence associated to
$\Gamma $
, and
$B_2(\Gamma )$
the set of its critical values.
Note that
$A_1(\Gamma )=\iota (A_2(\Gamma ^{-1}))$
and
$A_2(\Gamma )=\iota (A_1(\Gamma ^{-1}))$
, where
$\iota :\widehat {\mathbb {C}}\times \widehat {\mathbb {C}}\to \widehat {\mathbb {C}}\times \widehat {\mathbb {C}}$
is the involution
$\iota (z,w)=(w,z)$
.
Suppose
$\Gamma $
is the graph of a holomorphic correspondence on
$\widehat {\mathbb {C}}$
. Let
$g:\Omega \to \widehat {\mathbb {C}}$
be a holomorphic function defined on a domain
$\Omega $
of
$\widehat {\mathbb {C}}$
, whose graph
$\operatorname {Gr}(g)$
is contained in one of the irreducible components
$\Gamma (i)$
of
$\Gamma $
. If
$a\in \Omega $
is a critical point for g, then
$(a,g(a))\in A_2(\Gamma (i))\subset A_2(\Gamma )$
and therefore
$g(a)\in B_2(\Gamma )$
, i.e., the critical value
of the function g is a critical value for the holomorphic correspondence associated to
$\Gamma $
, as well.
On the other hand, if
$\alpha \notin A_1(\Gamma )$
, then there exists a holomorphic function
$g:\Omega \to \widehat {\mathbb {C}}$
defined on a neighborhood
$\Omega $
of
$a=\pi (\alpha )$
, such that
$(a,g(a))=\alpha $
, and
$\operatorname {Gr}(g)\subset \Gamma (i)$
for some i. If in addition
$\alpha \in A_2(\Gamma )$
, then g is not locally injective at a, and therefore
$g'(a)=0$
. Therefore, a is a critical point of g and
$\pi _2(\alpha )=g(a)$
is a critical value of g.
If we denote by
$\operatorname {CritPt}(g)$
the set of critical points of g, and by
the set of critical values of g, then we get a motivation for the name ‘critical values’ in Definition 2.3 by the containment
$$ \begin{align*}B_2(\Gamma)\setminus B_1(\Gamma)\subset\bigcup\limits_{i}\bigcup\limits_{\operatorname{Gr}(g)\subset\Gamma(i)}\operatorname{CritVal}(g),\end{align*} $$
where the first union runs over the irreducible components of
$\Gamma $
and the second union runs over all the holomorphic functions
$g:\Omega \to \widehat {\mathbb {C}}$
whose graph
$\operatorname {Gr}(g)$
is contained in
$\Gamma (i)$
.
Proposition 2.4. For every
$a\in \mathbb {C}\setminus \lbrace 1\rbrace $
, we have that
$$ \begin{align} A_1(\Gamma_a)=\bigg\lbrace \bigg(\infty,\frac{a+1}{2}\bigg),\bigg( -2,1\bigg),\bigg(2,\frac{3a+1}{3+a}\bigg)\bigg\rbrace, \end{align} $$
and
$$ \begin{align} A_2(\Gamma_a)=\bigg\lbrace \bigg(\infty,\frac{a+1}{2}\bigg),\bigg(1,\frac{4a+2}{a+5}\bigg), \bigg(-1,\frac{2}{3-a}\bigg)\bigg\rbrace. \end{align} $$
As a consequence,
$B_1(\Gamma _a)=\lbrace \infty ,-2,2\rbrace $
and
$B_2(\Gamma _a)=\lbrace ({a+1})/{2},({4a+2})/({a+5}), {2}/({3-a})\rbrace $
. Moreover,
$\Gamma _a$
is smooth at all points except
$(\infty ,({a+1})/{2})$
.
To prove this proposition, we first prove the following lemma.
Lemma 2.5. For each
$a\in \mathbb {C}\setminus \lbrace 1\rbrace $
,
$$ \begin{align*}A_1(\Gamma_{\operatorname{Cov^Q_0}})=\lbrace(\infty,\infty),(-2,1),(2,-1)\rbrace\end{align*} $$
and
$$ \begin{align*}A_2(\Gamma_{\operatorname{Cov^Q_0}})=\lbrace(\infty,\infty),(1,-2),(-1,2)\rbrace.\end{align*} $$
Thus,
$B_1(\Gamma _{\operatorname {Cov^Q_0}})=\lbrace \infty , -2,2\rbrace $
and
$B_2(\Gamma _{\operatorname {Cov^Q_0}})=\lbrace \infty , 1,-1\rbrace $
.
Proof. Differentiating the equation
$P_Q(z,w)=0$
with respect to w, we get that
$\partial _w P_Q(z,w)=z+2w$
vanishes if and only if
$w={-z}/{2}$
. Also,
$P_Q(z,-{z}/{2})=0$
if and only if
$z=\pm 2$
. Therefore,
${dw}/{dz}$
exists on
$\mathbb {C}\setminus \lbrace -2, 2\rbrace $
. Thus, by the implicit function theorem, for every
$(z,w)\in \Gamma _{\operatorname {Cov^Q_0}}$
such that
$z\in \mathbb {C}\setminus \lbrace -2,2\rbrace $
, there exists a domain
$\Omega $
containing z and a holomorphic function
$g:\Omega \to \widehat {\mathbb {C}}$
such that
$g(z)=w$
and
$\operatorname {Gr}(g)=\Gamma _{\operatorname {Cov^Q_0}}\cap U$
, for some open neighborhood U of
$(z,w)$
. In addition, the function g will be locally injective at z if
$\partial _z P_Q(z,w)=2z+w$
is non-zero. Therefore, if
$(z,w)\in \Gamma _{\operatorname {Cov^Q_0}}$
satisfies that both z and w are different from
$\pm 2$
, then
$(z,w)\notin A_2(\Gamma _{\operatorname {Cov^Q_0}})$
.
On the other hand, observe that the only points
$(z,w)\in \Gamma _{\operatorname {Cov^Q_0}}$
with
$z=\pm 2$
are
$(-2,1)$
and
$(2,-1)$
. In particular,
$w\neq \pm 2$
, and by the symmetry of the above argument, we can use the implicit function theorem to obtain a neighborhood U of w and a function
$g:U\to \widehat {\mathbb {C}}$
satisfying
$\operatorname {Gr}(g)\subset \Gamma _{\operatorname {Cov^Q_0}}$
and
$g(w)=z$
, and such that
is injective in the neighborhood
$(g(U)\times U)\cap \Gamma _{\operatorname {Cov^Q_0}}$
of
$(z,w)$
. This proves that neither
$(-2,1)$
or
$(2,-1)$
belong to
$A_2(\Gamma _{\operatorname {Cov^Q_0}})$
. Since the only points
$(z,w)\in \Gamma _{\operatorname {Cov^Q_0}}$
with
$z=\pm 1$
are
$(1,-2)$
and
$(-1,2)$
, and since
$\Gamma _{\operatorname {Cov^Q_0}}\setminus (\mathbb {C}\times \mathbb {C})=\lbrace (\infty ,\infty )\rbrace $
, we have that
$A_2(\Gamma _{\operatorname {Cov^Q_0}})$
is contained in
$\lbrace (\infty ,\infty ),(1,-2),(-1,2)\rbrace $
.
We will check that for every neighborhood W of
$(\infty ,\infty )$
,
$(1,-2)$
, and
$(-1,2)$
, we have that
is not injective. Let
$W_1,W_2$
, and
$W_3$
be open neighborhoods of
$(\infty ,\infty )$
,
$(1,-2)$
, and
$(-1,2)$
, respectively. Then there exists
$T>0$
such that for every
$0<t<T$
,
$$ \begin{align*}\bigg(\frac{1}{2}\bigg(\!\pm\sqrt{3}\sqrt{-\frac{1}{t^2}-\frac{4}{t}}+\frac{1}{t}+2\bigg),-2-\frac{1}{t}\bigg)\in\Gamma_{\operatorname{Cov^Q_0}}\cap W_1,\end{align*} $$
$$ \begin{align*}\bigg(\frac{1}{2}\big(\!\pm\sqrt{3}\sqrt{-t^2-4t}+t+2\big),-2-t\bigg)\in\Gamma_{\operatorname{Cov^Q_0}}\cap W_2,\end{align*} $$
and
$$ \begin{align*}\bigg(\frac{1}{2}\big(\!\pm\sqrt{3}\sqrt{4t-t^2}+t-2\big),2-t\bigg)\in\Gamma_{\operatorname{Cov^Q_0}}\cap W_3.\end{align*} $$
We conclude that
$A_2(\Gamma _{\operatorname {Cov^Q_0}})=\lbrace (\infty ,\infty ),(1,-2),(-1,2)\rbrace $
, and by the symmetry of
$\operatorname {Cov^Q_0}$
,
$A_1(\Gamma _{\operatorname {Cov^Q_0}})=\lbrace (\infty ,\infty ),(-2,1),(2,-1)\rbrace $
. We conclude that
$$ \begin{align*}B_2(\Gamma_{\operatorname{Cov^Q_0}})=\lbrace \infty, -2,2\rbrace=B_1(\Gamma_{\operatorname{Cov^Q_0}}).\\[-40pt]\end{align*} $$
Proof of Proposition 2.4.
Since
$\mathcal {F}_a=\operatorname {J}_a\circ \operatorname {Cov^Q_0}$
and
$\operatorname {J}_a$
is an involution, we have that
$$ \begin{align*}A_j(\Gamma_a)=\lbrace (z,\operatorname{J}_a(w)):(z,w)\in A_j(\Gamma_{\operatorname{Cov^Q_0}})\rbrace,\end{align*} $$
for
$j=1,2$
. Using Lemma 2.5, this gives us equations (3) and (4), and thus
$B_1(\Gamma _a)=\lbrace \infty , -2,2\rbrace $
and
$$ \begin{align*}B_2(\Gamma_a)=\lbrace \operatorname{J}_a(\infty),\operatorname{J}_a(-2),\operatorname{J}_a(2)\rbrace=\bigg\lbrace \frac{a+1}{2},\frac{4a+2}{a+5},\frac{2}{3-a}\bigg\rbrace.\end{align*} $$
In addition, observe that locally,
$\Gamma _a$
is either a function on z or on w for all
$(z,w)\in \Gamma _a$
with
$z\neq \infty $
, then the only point that can be irregular is
$(\infty ,({a+1})/{2})$
. Indeed, this point is irregular, as the curve given by the points
$$ \begin{align*}\bigg(\frac{1}{2}\bigg(\pm\sqrt{3}\sqrt{-\frac{1}{t^2}-\frac{4}{t}}+\frac{1}{t}+2\bigg),-2-\frac{1}{t}\bigg)\in\Gamma_{\operatorname{Cov^Q_0}}\end{align*} $$
self-intersects at
$(\infty ,\infty )$
with an angle of
${2\pi }/{3}$
. In other words, there are two functions
$z(w)$
which intersect with different derivatives, which makes
$(\infty ,\infty )$
an irregular point of
$\Gamma _{\operatorname {Cov^Q_0}}$
. Thus, passing through the involution
$\operatorname {J}_a$
, we get that
$(\infty ,({a+1})/{2})$
is an irregular point of
$\Gamma _a$
.
Remark 2.6. The correspondence
$\operatorname {Cov^Q_0}$
, and hence
$\mathcal {F}_a$
, sends open sets to open sets. Indeed, let
$U\subset \widehat {\mathbb {C}}$
be open and take
$w_0\in \operatorname {Cov^Q_0}(U)$
. Then there exists
$z_0\in U$
for which
$(z_0,w_0)\in \Gamma _{\operatorname {Cov^Q_0}}$
. We will prove that
$\operatorname {Cov^Q_0}(U)$
is open by showing that in all the cases,
$w_0\in \operatorname {int}(\operatorname {Cov^Q_0}(U))$
.
-
• Suppose
$(z_0,w_0)\notin A_1(\Gamma _{\operatorname {Cov^Q_0}})$
. By Lemma 2.5 and since
$(\operatorname {Cov^Q_0})^{-1}(\infty )=\lbrace \infty \rbrace $
, we have that
$w_0\neq \infty $
. Moreover, there exists a holomorphic function
$g:\Omega \to \mathbb {C}$
on an open subset
$\Omega \subset U$
, and
$(z_0,w_0)\in \operatorname {Gr}(g)\subset \Gamma _{\operatorname {Cov^Q_0}}$
. Furthermore,
$\Gamma _{\operatorname {Cov^Q_0}}$
is irreducible and
$\operatorname {Cov^Q_0}$
is not constant, so g is not constant. Thus, g is open and then
$w_0\in g(\Omega )\subset \operatorname {int}(\operatorname {Cov^Q_0}(U))$
. -
• Now suppose
$(z_0,w_0)\notin A_2(\Gamma _{\operatorname {Cov^Q_0}})$
. By Lemma 2.5 and since
$\operatorname {Cov^Q_0}(\infty )=\lbrace \infty \rbrace $
, then
$z_0\neq \infty $
and there exists a holomorphic function
$\tilde {g}:\widetilde {\Omega }\to \mathbb {C}$
on an open subset
$\widetilde {\Omega }\subset \mathbb {C}$
so that
$(z_0,w_0)\in \iota (\operatorname {Gr}(\tilde {g}))\subset \Gamma _{\operatorname {Cov^Q_0}}$
. Since
$\tilde {g}$
is continuous, then
$\tilde {g}^{-1}(U)$
is open and
$w_0\in \tilde {g}^{-1}(U)\subset \operatorname {int}(\operatorname {Cov^Q_0}(U))$
. -
• Finally, if
$(z_0,w_0)\in A_1(\Gamma _{\operatorname {Cov^Q_0}})\cap A_2(\Gamma _{\operatorname {Cov^Q_0}})$
, then Lemma 2.5 implies that
$(z_0,w_0)=(\infty ,\infty )$
. For each
$r>0$
, put
. To show that
$\infty \in \operatorname {int}(\operatorname {Cov^Q_0}(U))$
, we will show that for every
$R>\sqrt {3}$
,
$U_{2R}\subset \operatorname {Cov^Q_0}(U_R)$
. We proceed by the contrapositive. If
$P_Q(z,w)=0$
and
$|w|\leq R$
, then Hence,
$$ \begin{align*} |z|^2=|3-zw-w^2|\leq 3+|z||w|+|w|^2\leq 2R^2+|z|R. \end{align*} $$
$|z|^2-R|z|-2R^2\leq 0$
and
$|z|\leq 2R$
. This implies that for every
$R>\sqrt {3}$
, we have that
$\operatorname {Cov^Q_0}(U_{2R})\subset U_R$
. By the symmetry of
$\operatorname {Cov^Q_0}$
, Moreover, if
$$ \begin{align*}U_{2R}\subset\operatorname{Cov^Q_0}(\operatorname{Cov^Q_0}(U_{2R}))\subset\operatorname{Cov^Q_0}(U_R).\end{align*} $$
$R>\sqrt {3}$
is large enough so that
$U_R\subset U$
, then
$$ \begin{align*} \infty\in U_{2R}\subset \operatorname{int}(\operatorname{Cov^Q_0}(U)).\end{align*} $$
Therefore,
$\operatorname {Cov^Q_0}$
sends open sets to open sets. Since the involution
$\operatorname {J}_a$
also sends open sets to open sets, then so does
$\mathcal {F}_a$
.
2.4 Klein combination pairs
In this section, we will define the set
$\mathcal {K}$
of parameters we will consider for our family.
Definition 2.7. A fundamental domain for an irreducible holomorphic correspondence F is an open set
$\Delta _F$
that is maximal with the property that
$\Delta _F\cap F(\Delta _F)=\emptyset $
.
Definition 2.8. We say that a pair
$(\Delta _{\operatorname {Cov^Q_0}},\Delta _{\operatorname {J}_a})$
of fundamental domains for
$\operatorname {Cov^Q_0}$
and
$\operatorname {J}_a$
, respectively, is a Klein combination pair for
$\mathcal {F}_a$
if both
$\Delta _{\operatorname {Cov^Q_0}}$
and
$\Delta _{\operatorname {J}_a}$
are simply connected domains, bounded by Jordan curves, and satisfy
$$ \begin{align*} \Delta_{\operatorname{Cov^Q_0}}\cup\Delta_{\operatorname{J}_a}=\widehat{\mathbb{C}}\setminus\lbrace 1\rbrace. \end{align*} $$
We define as well the Klein combination locus
$\mathcal {K}$
to be the set of parameters
$a\in \mathbb {C}\setminus \lbrace 1\rbrace $
for which there exist a Klein combination pair.
In [Reference Bullett and Lomonaco4], for
$|a-4|\leq 3$
,
$a\neq 1$
, the authors found a Klein combination pair for
$\mathcal {F}_a$
, where
$\Delta _{\operatorname {Cov^Q_0}}$
is given by the right side of the curve
and
$\Delta _{\operatorname {J}_a}$
is given by the exterior of the circle passing through
$z=1$
and
$z=a$
with diameter contained in the real line.
This pair
$(\Delta _{\operatorname {Cov^Q_0}},\Delta _{\operatorname {J}_a})$
is composed by simply connected fundamental domains for
$\operatorname {Cov^Q_0}$
and
$\operatorname {J}_a$
, respectively, whose boundaries are Jordan curves, smooth except from
$\partial \Delta _{\operatorname {Cov^Q_0}}$
at
$(\infty ,\infty )$
(see Figure 1 and [Reference Bullett and Lomonaco4, Proposition 3.3]). In particular,
$$ \begin{align*}\lbrace a\in\mathbb{C}|\mbox{ } |a-4|\leq 3\rbrace\setminus\lbrace 1\rbrace\subset\mathcal{K}.\end{align*} $$
Figure 1 Klein combination pair for
$|a-4|\leq 3$
.
From now on, whenever
$a\in \mathcal {K}$
, we denote by
$(\Delta _{\operatorname {Cov^Q_0}},\Delta _{\operatorname {J}_a})$
a Klein combination pair for
$\mathcal {F}_a$
.
The following remark will be useful to prove that
$\mathcal {F}_a$
is not modular, and later to analyze the asymptotic behavior of
$\mathcal {F}_a$
.
Remark 2.9. Let
$a\in \mathcal {K}$
.
-
(1) Note that
$\mathcal {F}_a(\widehat {\mathbb {C}}\setminus \Delta _{\operatorname {J}_a})\subset \widehat {\mathbb {C}}\setminus \Delta _{\operatorname {J}_a}$
and
${\mathcal {F}_a((\widehat {\mathbb {C}}\setminus \Delta _{\operatorname {J}_a})\setminus \lbrace 1\rbrace )\subset \widehat {\mathbb {C}}\setminus \overline {\Delta _{\operatorname {J}_a}}}$
. Indeed,
$$ \begin{align*}\mathcal{F}_a(1)=\operatorname{J}_a(\operatorname{Cov^Q_0} (1))=\operatorname{J}_a(\lbrace 1,-2\rbrace)=\bigg\lbrace 1,\frac{4a+2}{a+5}\bigg\rbrace.\end{align*} $$
From Remark 2.6, we have that
$\operatorname {Cov^Q_0}$
sends open sets to open sets. In particular,
$-$
2 cannot belong to
$\Delta _{\operatorname {Cov^Q_0}}$
, as
$1\notin \operatorname {Cov^Q_0}(\Delta _{\operatorname {Cov^Q_0}})=\widehat {\mathbb {C}}\setminus \overline {\Delta _{\operatorname {Cov^Q_0}}}$
. By the Klein combination pair condition, this implies that
$-2\in \Delta _{\operatorname {J}_a}$
, and thus
$\operatorname {J}_a(-2)=({4a+2})/({a+5})\notin \Delta _{\operatorname {J}_a}$
. Since we also have that
$1\notin \Delta _{\operatorname {J}_a}$
, we have that
$$ \begin{align*}\mathcal{F}_a(1)\subset\widehat{\mathbb{C}}\setminus\Delta_{\operatorname{J}_a}.\end{align*} $$
However, for
$z\in \widehat {\mathbb {C}}\setminus \Delta _{\operatorname {J}_a}$
,
$z\neq 1$
, we have that
$z\in \Delta _{\operatorname {Cov^Q_0}}$
. Therefore,
$$ \begin{align*}\operatorname{Cov^Q_0}(z)\subset\widehat{\mathbb{C}}\setminus\overline{\Delta_{\operatorname{Cov^Q_0}}}\subset\Delta_{\operatorname{J}_a},\end{align*} $$
and thus, since
$\operatorname {J}_a$
is an involution fixing
$\partial \Delta _{\operatorname {J}_a}$
,
$$ \begin{align*}\mathcal{F}_a(z)=\operatorname{J}_a\circ\operatorname{Cov^Q_0}(z)\subset\operatorname{J}_a(\Delta_{\operatorname{J}_a})=\widehat{\mathbb{C}}\setminus\overline{\Delta_{\operatorname{J}_a}}.\end{align*} $$
In particular,
$\Delta _{\operatorname {J}_a}\setminus \mathcal {F}_a(\widehat {\mathbb {C}}\setminus \Delta _{\operatorname {J}_a})\neq \emptyset $
. -
(2) Observe that
$\mathcal {F}_a^{-1}(\Delta _{\operatorname {J}_a})\subset \Delta _{\operatorname {J}_a}$
and
$\mathcal {F}_a^{-1}(\overline {\Delta _{\operatorname {J}_a}})\subset \Delta _{\operatorname {J}_a}\cup \lbrace 1\rbrace $
. Indeed, for every
$z\in \Delta _{\operatorname {J}_a}$
, we have that
$\operatorname {J}_a(z)\in \widehat {\mathbb {C}}\setminus \overline {\Delta _{\operatorname {J}_a}}\subset \Delta _{\operatorname {Cov^Q_0}}$
. From Remark 2.6 and since
$(\Delta_{\operatorname {Cov^Q_0}},\Delta _{\operatorname {J}_a})$
is a Klein combination pair, then
$$ \begin{align*}\mathcal{F}_a^{-1}(z)=\operatorname{Cov^Q_0}(\widehat{\mathbb{C}}\setminus\overline{\Delta_{\operatorname{J}_a}})\subset\operatorname{Cov^Q_0}(\Delta_{\operatorname{Cov^Q_0}})\subset\widehat{\mathbb{C}}\setminus\overline{\Delta_{\operatorname{Cov^Q_0}}}\subset\Delta_{\operatorname{J}_a}.\end{align*} $$
Furthermore, for
$w\in \overline {\Delta _{\operatorname {J}_a}}$
, we have that
3 Clasification of the family
$\lbrace \mathcal {F}_a\rbrace _a$
In this section, we prove that
$\mathcal {F}_a$
is weakly modular but not modular, for every
$a\in \mathcal {K}$
. In addition, we prove that it does not satisfy the required conditions for the equidistribution result [Reference Bharali and Sridharan1, Theorem 3.5]. All together proving Theorem 1.1.
3.1 Modularity and weak modularity
Definition 3.1. Let G be a connected Lie group,
$\Lambda $
a torsion free lattice, and K a compact Lie subgroup. Let
$g\in G$
be such that
$g\Lambda g^{-1}\cap \Lambda $
has finite index in
$\Lambda $
. The irreducible modular correspondence induced by g is the multivalued map
$F_g$
on
$X=\Lambda \setminus G/K$
corresponding to the projection to X of the map
$x\mapsto (x,gx)$
on
$G\to G\times G$
. Denote by
$\Gamma _g$
the graph of
$F_g$
. A modular correspondence F is a correspondence whose graph is of the form
$\sum _jn_j\Gamma _{g_j}$
, for
$\Gamma _{g_j}$
as before.
The following definition was introduced by Dinh, Kaufmann, and Wu in [Reference Dinh, Kaufmann and Wu15].
Definition 3.2. Let X be a compact Riemann surface and let F be a holomorphic correspondence on X with graph
$\Gamma $
such that
$d(F)=d(F^{-1})$
. We say that F is a weakly modular correspondence if there exist Borel probability measures
$\mu _1$
and
$\mu _2$
on X, such that
Remark 3.3
-
(1) Let F be a modular correspondence that is also a holomorphic correspondence. Then it is always the case that
$$ \begin{align*} d(F)=\sum_j n_j[\Lambda: g_j\Lambda g_j^{-1}\cap\Lambda]=\sum_j n_j[\Lambda:g_j^{-1}\Lambda g_j\cap\Lambda]=d(F^{-1}). \end{align*} $$
-
(2) With the notation in Definition 3.1, let
$\unicode{x3bb} $
be the direct image on X of the finite Haar measure on
$\Lambda \setminus G$
. Then,
$(1/d){(F_g)}F_g^*\unicode{x3bb} =\unicode{x3bb} $
and if we put
$\mu _1=\mu _2=\unicode{x3bb} $
, we get that Therefore, modular correspondences are weakly modular.
-
(3) The measure
$\unicode{x3bb} $
above is Borel, invariant under
$F_g$
, and assigns positive measure to non-empty open sets.
Proof of Theorem 1.1 part 1.
Observe that the graph
$\Gamma _{\operatorname {Cov^Q_0}}$
of the correspondence
$\operatorname {Cov^Q_0}$
is symmetric with respect to the diagonal
$\mathfrak {D}_{\widehat {\mathbb {C}}}=\lbrace (z,z) | z\in \widehat {\mathbb {C}}\rbrace $
, as
$z\in \operatorname {Cov^Q_0}(w)$
if and only if
$w\in \operatorname {Cov^Q_0}(z)$
. Let m be any positive and finite measure on
$\Gamma _{\operatorname {Cov^Q_0}}$
, and
$\iota :\Gamma _{\operatorname {Cov^Q_0}}\to \Gamma _{\operatorname {Cov^Q_0}}$
the involution
. Take
The measure
$m_0$
is symmetric in
$\Gamma _{\operatorname {Cov^Q_0}}$
in the sense that
$\iota ^*m'=m'$
and moreover
After normalizing if necessary, this proves that
$\operatorname {Cov^Q_0}$
is weakly modular with measures
and
. Our goal is to show there are probability measures
$\mu _1$
and
$\mu _2$
on
$\widehat {\mathbb {C}}$
such that
where
$\Gamma _a$
is the graph of the correspondence
$\mathcal {F}_a=\mathcal {F}_a\circ \operatorname {Cov^Q_0}$
.
Put
and observe that by symmetry of
$\operatorname {J}_a$
, we have that
Now let
$\widehat {\operatorname {J}_a}:\widehat {\mathbb {C}}\times \widehat {\mathbb {C}}\to \widehat {\mathbb {C}}\times \widehat {\mathbb {C}}$
be given by
. Observe that whenever
$(z,w)\in \Gamma _{\operatorname {Cov^Q_0}}$
, then
$(z,\operatorname {J}_a(w))\in \Gamma _a$
and
On the other hand,
with multiplicity
$2$
. This, together with equations (5), (7), and (8) yield
Observe that
and
$\mu _2$
both have
$2$
times the mass of
$\mu _1$
and
$\mu _2$
. After normalizing, equation (9) proves that
$\mathcal {F}_a$
is weakly modular, as desired.
To check
$\mathcal {F}_a$
is not modular, we will prove that no Borel measure
$\unicode{x3bb} $
on
$\widehat {\mathbb {C}}$
that gives positive measure to non-empty open sets can be invariant under
$\mathcal {F}_a$
. Suppose by contradiction that
$\unicode{x3bb} $
is such a measure satisfying
$\tfrac 12\mathcal {F}_a^*\unicode{x3bb} =\unicode{x3bb} $
. In particular, we have that
$\unicode{x3bb} ((\widehat {\mathbb {C}}\setminus \Delta _{\operatorname {J}_a})\setminus \mathcal {F}_a(\widehat {\mathbb {C}}\setminus \Delta _{J}))=0$
. On the other hand, note that
is closed in
$\widehat {\mathbb {C}}$
. By Remark 2.9 part (1),
$(\widehat {\mathbb {C}}\setminus \overline {\Delta _{\operatorname {J}_a}})\setminus \mathcal {F}_a(\widehat {\mathbb {C}}\setminus \Delta _{\operatorname {J}_a})$
is open and non-empty, contained in
$(\widehat {\mathbb {C}}\setminus \Delta _{\operatorname {J}_a})\setminus \mathcal {F}_a(\widehat {\mathbb {C}}\setminus \Delta _{J})$
. This contradicts part
$(3)$
of Remark 3.3, as
$\unicode{x3bb} $
cannot assign 0 measure to open sets.
Remark 3.4. For a
$(d,d)$
holomorphic correspondence F on compact Riemann surface X, the operator
$({1}/{d})F^*$
acts on the space
$L^2_{(1,0)}$
of
$(1,0)$
-forms with
$L^2$
coefficients. In [Reference Dinh, Kaufmann and Wu15], the authors showed that the operator norm satisfies
$\Vert ({1}/{d})F^*\Vert \leq 1$
, with strict inequality for non weakly modular correspondences. This strict inequality is a key factor of their equidistribution result. However, this is never the case for
$F=\mathcal {F}_a$
,
$a\neq 1$
. We claim that
$$ \begin{align*}\big\Vert\tfrac{1}{2}\mathcal{F}_a^*\big\Vert=\sup\big\lbrace\big\Vert\tfrac{1}{2}\mathcal{F}_a^*\phi\big\Vert_{L^2}\big|\phi\in L^2_{(1,0)},\Vert\phi\Vert_{L^2}=1\big\rbrace=1,\end{align*} $$
and furthermore that the supremum is attained. To prove this, we use that
$\|({1}/{d})F^*\phi \|_{L^2}=\|\phi \|_{L^2}$
for
$\phi \in L^2_{(1,0)}$
if and only if for every
$U\subset X\setminus B_1(\Gamma )$
and for every pair of local branches
$f_1$
and
$f_2$
of F on U, the equality
$f_1^*\phi =f_2^*\phi $
holds on U (see [Reference Dinh, Kaufmann and Wu15, Proposition 2.1]).
Observe that the form
$\phi (z)=e^{-|Q(z)|}\,dz$
belongs to
$L^2_{(1,0)}$
for Q as in §2.2. Let
$U\subset \widehat {\mathbb {C}}\setminus B_1(\Gamma _{\operatorname {Cov^Q_0}})$
. Then the deleted covering correspondence
$\operatorname {Cov^Q_0}$
sends z to the values w for which
$({Q(z)-Q(w)})/({z-w})=0$
. Hence, any two local branches
$f_1$
and
$f_2$
of
$\operatorname {Cov^Q_0}$
satisfy
$f_1^*\phi (z)=Q(z)=f_2^*\phi (z)$
, and thus
$\Vert \tfrac 12\operatorname {Cov^Q_0}^*\!\Vert =1$
. Now note that
$\operatorname {J}_a$
is an involution, and hence
$\operatorname {J}_a^*$
has operator norm
$1$
. Thus we can conclude that
$\Vert \tfrac 12\mathcal {F}_a^*\Vert =1$
as well, with supremum attained at
$\phi $
.
3.2 Limit sets
In this section, we define limit sets and give some properties. We will also prove that the application listed in [Reference Bharali and Sridharan1, §7] does not hold for our family of correspondences, and hence this is a new case to study equidistribution.
Remark 3.5. From Proposition 2.4, observe that
$1\notin B_1(\Gamma _a)$
, and hence there is a holomorphic function g whose graph contains
$(1,1)$
and is contained in
$\Gamma _a$
. After the change of coordinates
$\psi (z)=z-1$
, the function g has Taylor series expansion
$$ \begin{align*}g^{\psi}(z)=z+\frac{a-7}{3(a-1)}z^2+\cdots\end{align*} $$
whenever
$a\neq 7$
, and
$$ \begin{align*}g^{\psi}(z)=z+\frac{1}{27}z^4+\cdots\end{align*} $$
for
$a=7$
(see [Reference Bullett and Lomonaco4, Proposition 3.5]). In particular,
$g^{\psi }$
has a parabolic fixed point at
$0$
with multiplier
$1$
.
In [Reference Bullett and Lomonaco4, Proposition 3.8], the authors showed that for each
$a\in \mathcal {K}$
, after a small perturbation of
$\partial \Delta _{\operatorname {Cov^Q_0}}$
and
$\partial \Delta _{\operatorname {J}_a}$
around
$z=1$
, we can choose a Klein combination pair
$(\Delta ^{\prime }_{\operatorname {Cov^Q_0}},\Delta ^{\prime }_{\operatorname {J}_a})$
so that
$\partial \Delta ^{\prime }_{\operatorname {Cov^Q_0}}$
and
$\partial \Delta ^{\prime }_{\operatorname {J}_a}$
are both smooth at
$1$
, and transverse at
$1$
to the line generated by the repelling direction at
$z=1$
for
$a\neq 7$
, and to the real axis in the case
$a=7$
.
For
$a\in \mathcal {K}$
and
$(\Delta _{\operatorname {Cov^Q_0}},\Delta _{\operatorname {J}_a})$
as above, we define
to be the forward and backward limit set of
$\mathcal {F}_a$
, respectively. These sets do not depend on the choice of the Klein combination pair
$(\Delta ^{\prime }_{\operatorname {Cov^Q_0}},\Delta ^{\prime }_{\operatorname {J}_a})$
as above.
Lemma 3.6. Let
$a\in \mathcal {K}$
. We have that:
-
(1)
$\operatorname {J}_a(\Lambda _{a,\pm })=\Lambda _{a,\mp }$
and
$\operatorname {J}_a(\partial \Lambda _{a,\pm })=\partial \Lambda _{a,\mp }$
; -
(2)
$\Lambda _{a,-}\cap \Lambda _{a,+}=\lbrace 1\rbrace ;$
-
(3) if
$z\notin \Lambda _{a,-}$
, then there exists
$n\geq 1$
so that
$\mathcal {F}_a^{n}(z)\subset \widehat {\mathbb {C}}\setminus \Delta ^{\prime }_{\operatorname {J}_a}$
, and if
$z\notin \Lambda _{a,+}$
, then there exists
$n\geq 1$
such that
$\mathcal {F}_a^{-n}(z)\subset \Delta ^{\prime }_{\operatorname {J}_a}$
; -
(4)
$\mathcal {F}_a^{-1}(\Lambda _{a,-})=\Lambda _{a,-}$
and
$\mathcal {F}_a^{-1}(\partial \Lambda _{a,-})=\partial \Lambda _{a,-}$
; and -
(5)
$\mathcal {F}_a(\Lambda _{a,+})=\Lambda _{a,+}$
and
$\mathcal {F}_a(\partial \Lambda _{a,+})=\partial \Lambda _{a,+}$
.
Proof. Since
$\operatorname {J}_a$
is an involution sending
$\overline {\Delta ^{\prime }_{\operatorname {J}_a}}$
and
$\widehat {\mathbb {C}}\setminus \Delta ^{\prime }_{\operatorname {J}_a}$
to each other, and since
$\operatorname {J}_a\circ \mathcal {F}_a^n=\mathcal {F}_a^{-n}\circ \operatorname {J}_a$
, then
$$ \begin{align*}\operatorname{J}_a(\Lambda_{a,+})=\bigcap\limits_{n=0}^{\infty}\operatorname{J}_a\circ\mathcal{F}_a^n(\widehat{\mathbb{C}}\setminus\Delta^{\prime}_{\operatorname{J}_a})=\bigcap\limits_{n=0}^{\infty}\mathcal{F}_a^{-n}\circ\operatorname{J}_a(\widehat{\mathbb{C}}\setminus\Delta^{\prime}_{\operatorname{J}_a})=\Lambda_{a,-},\end{align*} $$
and applying
$\operatorname {J}_a$
to both sides, we also get
$\operatorname {J}_a(\Lambda _{a,-})=\Lambda _{a,+}$
. Moreover, since
$\operatorname {J}_a$
is continuous, note that
$$ \begin{align*}\Lambda_{a,-}\cap\Lambda_{a,+}\subset\overline{\Delta^{\prime}_{\operatorname{J}_a}}\cap(\widehat{\mathbb{C}}\setminus\Delta^{\prime}_{\operatorname{J}_a})=\partial\Delta^{\prime}_{\operatorname{J}_a}.\end{align*} $$
Since
$(\Delta ^{\prime }_{\operatorname {Cov^Q_0}},\Delta ^{\prime }_{\operatorname {J}_a})$
is a Klein combination pair,
$\widehat {\mathbb {C}}\setminus \Delta ^{\prime }_{\operatorname {J}_a}\subset \Delta ^{\prime }_{\operatorname {Cov^Q_0}}$
, so
$\partial \Delta ^{\prime }_{\operatorname {J}_a}\subset \overline {\Delta ^{\prime }_{\operatorname {Cov^Q_0}}}$
.
If
$z\in \Delta ^{\prime }_{\operatorname {Cov^Q_0}}$
, then
$\operatorname {Cov^Q_0}(z)\subset \widehat {\mathbb {C}}\setminus \Delta ^{\prime }_{\operatorname {J}_a}$
, and
$\mathcal {F}_a(z)=\operatorname {J}_a\circ \operatorname {Cov^Q_0}(z)\subset \Delta ^{\prime }_{\operatorname {J}_a}$
. This is a contradiction, as
$\mathcal {F}_a(z)$
must belong to
$\Lambda _{a,+}\subset \widehat {\mathbb {C}}\setminus \Delta ^{\prime }_{\operatorname {J}_a}$
as well. Again, since
$(\Delta ^{\prime }_{\operatorname {Cov^Q_0}},\Delta ^{\prime }_{\operatorname {J}_a})$
is a Klein combination pair, we have that
$z\in \partial \Delta ^{\prime }_{\operatorname {Cov^Q_0}}\cap \partial \Delta ^{\prime }_{\operatorname {J}_a}=\lbrace 1\rbrace $
and we conclude that
$$ \begin{align*}\Lambda_{a,-}\cap\Lambda_{a,+}=\lbrace 1\rbrace.\end{align*} $$
We prove part
$(3)$
by the contrapositive. Suppose that for all n, there exists
$w\in \mathcal {F}_a^{n}(z)\cap (\widehat {\mathbb {C}}\setminus \Delta ^{\prime }_{\operatorname {J}_a})$
, then
$z\in \mathcal {F}_a^{n}(w)\subset \mathcal {F}_a^{n}(\widehat {\mathbb {C}}\setminus \Delta ^{\prime }_{\operatorname {J}_a})$
for all n. This implies that z must belong to
$\Lambda _{a,-}$
. The other case is analogous.
It is immediate from the definition of
$\Lambda _{a,-}$
and from part
$(2)$
of Remark 2.9 that
$$ \begin{align} \mathcal{F}_a^{-1}(\Lambda_{a,-})=\Lambda_{a,-}. \end{align} $$
From this and Remark 2.6,
$$ \begin{align} \mathcal{F}_a^{-1}(\operatorname{int}(\Lambda_{a,-}))\subset\operatorname{int}(\mathcal{F}_a^{-1}(\Lambda_{a,-}))=\operatorname{int}(\Lambda_{a,-}). \end{align} $$
Observe that if
$z\in \partial \Lambda _{a,-}\setminus \lbrace 1\rbrace \subset \Delta ^{\prime }_{\operatorname {Cov^Q_0}}$
, then
$$ \begin{align*}\mathcal{F}_a(z)=\operatorname{J}_a(\operatorname{Cov^Q_0}(z))\subset\operatorname{J}_a(\widehat{\mathbb{C}})\subset\operatorname{J}_a(\Delta^{\prime}_{\operatorname{J}_a})\subset\widehat{\mathbb{C}}\setminus\overline{\Delta^{\prime}_{\operatorname{J}_a}}.\end{align*} $$
Since
$\Lambda _{a,-}\subset \overline {\Delta ^{\prime }_{\operatorname {J}_a}}$
, then we conclude that
$$ \begin{align} \Lambda_{a,-}\cap\partial\Delta^{\prime}_{\operatorname{J}_a}=\lbrace 1\rbrace. \end{align} $$
Put
$w\in \partial \Lambda _{a,-}$
and
$z\in \mathcal {F}_a^{-1}(w)\subset \Lambda _{a,-}$
. We will show that
$z\in \partial \Lambda _{a,-}$
by the contrapositive. Suppose
$w\neq 1$
and
$z\in \operatorname {int}(\Lambda _{a,-})$
. Then equation (12) implies that
$$ \begin{align*}w\in\mathcal{F}_a(\operatorname{int}(\Lambda_{a,-}))\cap\Delta^{\prime}_{\operatorname{J}_a}.\end{align*} $$
From Remark 2.6, and the fact that
$\Delta ^{\prime }_{\operatorname {J}_a}$
is open, we have that
$\mathcal {F}_a(\operatorname {int}(\Lambda _{a,-}))\cap \Delta ^{\prime }_{\operatorname {J}_a}$
is open. Moreover, for each
$z'\in \operatorname {int}(\Lambda _{a,-})$
, the set
$\mathcal {F}_a(z')$
consists of a point in
$\Delta ^{\prime }_{\operatorname {J}_a}$
and one in
${\widehat {\mathbb {C}}\setminus \Delta ^{\prime }_{\operatorname {J}_a}}$
. Since the one in
$\Delta ^{\prime }_{\operatorname {J}_a}$
is actually in
$\Lambda _{a,-}$
by definition of
$\Lambda _{a,-}$
, then
$\mathcal {F}_a(\operatorname {int}(\Lambda _{a,-}))\cap \Delta ^{\prime }_{\operatorname {J}_a}\subset \Lambda _{a,-}$
. Then
$w\in \operatorname {int}(\Lambda _{a,-})$
. This proves that
$$ \begin{align} \mathcal{F}_a^{-1}(\partial\Lambda_{a,-})\subset\Lambda_{a,-}. \end{align} $$
As for
$w=1$
, we have that
$z\in \mathcal {F}_a^{-1}(1)=\lbrace -2,1\rbrace $
. We know
$1\in \partial \Lambda _{a,-}$
, so it suffices to show that
$-2\in \partial \Lambda _{a,-}$
as well. Let
$U\subset \widehat {\mathbb {C}}$
be an open neighborhood of
$-2$
. From Remark 2.6, we have that
$\mathcal {F}_a(U)$
is an open neighborhood of
$1$
. Since
$1\in \partial \Delta ^{\prime }_{\operatorname {J}_a}$
, and
$\partial \Delta ^{\prime }_{\operatorname {J}_a}$
is a Jordan curve, then there exists a point
$w'\in U\cap \partial \Delta ^{\prime }_{\operatorname {J}_a}$
,
$w'\neq 1$
. We have that
$\mathcal {F}_a(\partial \Delta ^{\prime }_{\operatorname {J}_a}\setminus \lbrace 1\rbrace )\subset \widehat {\mathbb {C}}\setminus \overline {\Delta ^{\prime }_{\operatorname {Cov^Q_0}}}$
, so
$\mathcal {F}_a^{-1}(w')$
consists of two points,
$z',z"\in \widehat {\mathbb {C}}\setminus \overline {\Delta ^{\prime }_{\operatorname {Cov^Q_0}}}$
, with
$z'\in U$
. We will prove that
$z'\notin \Lambda _{a,-}$
by showing that
$\mathcal {F}_a(z')\cap \Lambda _{a,-}=\emptyset $
, and thus
$-2\in \Lambda _{a,-}\setminus \operatorname {int}(\Lambda _{a,-})=\partial \Lambda _{a,-}$
. Indeed,
$$ \begin{align*}\mathcal{F}_a(z')=\operatorname{J}_a(\lbrace z",\operatorname{J}_a(w')\rbrace)=\lbrace\operatorname{J}_a(z"),w'\rbrace\subset\widehat{\mathbb{C}}\setminus\Delta^{\prime}_{\operatorname{J}_a}.\end{align*} $$
From part
$(2)$
of Remark 2.9, we have that
$z'\notin \Lambda _{a,-}$
, and
$-2\in \partial \Lambda _{a,-}$
as desired. This, together with equations (10), (11), and (13) prove part
$(4)$
.
We have that part
$(1)$
of Remark 2.9 together with the definition of
$\Lambda _{a,+}$
prove that
$\mathcal {F}_a(\Lambda _{a,+})=\Lambda _{a,+}$
. To prove the rest of part
$(5)$
, we use part
$(4)$
together with the fact that
$\mathcal {F}_a=\operatorname {J}_a\circ \mathcal {F}_a^{-1}\circ \operatorname {J}_a$
and
$\operatorname {J}_a(\partial \Lambda _{a,\pm })=\partial \Lambda _{a,\mp }$
, as
$\operatorname {J}_a$
is a continuous involution. Thus,
$$ \begin{align*}\mathcal{F}_a(\partial\Lambda_{a,+})=\operatorname{J}_a\circ\mathcal{F}_a^{-1}\circ\operatorname{J}_a(\partial\Lambda_{a,+})=\operatorname{J}_a(\mathcal{F}_a^{-1}(\partial\Lambda_{a,-}))=\operatorname{J}_a(\partial\Lambda_{a,-})=\partial\Lambda_{a,+}.\qquad\\[-35pt]\end{align*} $$
The following definition is from [Reference Bharali and Sridharan1, Reference McGehee26].
Definition 3.7. Let F be a holomorphic correspondence on X. We say that
$\mathcal {R}\subset X$
is a repeller for F if there exists a set U such that
$\mathcal {R}$
is contained in the interior of U, and
$$ \begin{align*}\mathcal{R}=\bigcap_{K\in\mathfrak{K}(U,F^{-1})} K,\end{align*} $$
where
Bharali and Sridharan proved an equidistribution result similar to that in this paper [Reference Bharali and Sridharan1, Theorem 3.5] for correspondences having a repeller. Moreover, in [Reference Bharali and Sridharan1, §7.2], they showed that there is a set of pairs
$(a,k)$
for which their result can be applied to the correspondence
$$ \begin{align*}\bigg(\frac{az+1}{z+1}\bigg)^2+\bigg(\frac{az+1}{z+1}\bigg)\bigg(\frac{aw-1}{w-1}\bigg)+\bigg(\frac{aw-1}{w-1}\bigg)^2=3k.\end{align*} $$
For these correspondences (studied by Bullett and Harvey in [Reference Bullett and Harvey8]), there is a set
$\Lambda _{a,-}$
analogous to that presented here (see [Reference Bullett and Penrose7] for the general definition of limit sets). For the pair
$(a,k)$
to work for their theorem, it is crucial for
$\partial \Lambda _{a,-}$
to be a repeller for the correspondence. Nevertheless, part
$(2)$
of Theorem 1.1 says this never happens for
$k=1$
and
$|a-4|\leq 3$
.
Proof of Theorem 1.1 part (2).
Let
$U\subset \widehat {\mathbb {C}}$
contain
$\partial \Lambda _{a,-}$
in its interior. We have that
$1\in \partial \Lambda _{a,-}$
and is a parabolic fixed point of the function g whose graph is contained in
$\Gamma _a$
, described in Remark 3.5. Take an attracting petal
$\mathcal {P}$
at
$1$
so that
$\mathcal {P}\subset U$
. We first show that every
$K\in \mathfrak {K}(U,\mathcal {F}_a^{-1})$
contains
$\partial \Lambda _{a,-}\cup \mathcal {P}$
. Indeed, for every
$K\in \mathfrak {K}(U,\mathcal {F}_a^{-1})$
and some integer
$n\geq 0$
,
$$ \begin{align} \mathcal{P}\subset g^{-n}(\mathcal{P})\subset\mathcal{F}_a^{-n}(\mathcal{P})\subset\mathcal{F}_a^{-n}(U)\subset K. \end{align} $$
Moreover, from part (4) of Lemma 3.6, we have that
$$ \begin{align} \partial\Lambda_{a,-}\subset\mathcal{F}_a^{-n}(U)\subset K. \end{align} $$
Putting equations (14) and (15) together, we get that every
$K\in \mathfrak {K}(U,\mathcal {F}_a^{-1})$
contains the union
$\partial \Lambda _{a,-}\cup \mathcal {P}$
, and therefore so does the intersection over all K. Since
$\operatorname {int}(\partial \Lambda _{a,-})=\emptyset $
and
$\operatorname {int}(\mathcal {P})\neq \emptyset $
, we have that
$$ \begin{align*}\partial\Lambda_{a,-}\subsetneq\partial\Lambda_{a,-}\cup\mathcal{P}\subset\bigcap_{K\in\mathfrak{K}(U,\mathcal{F}_a^{-1})} K.\end{align*} $$
Since U is arbitrary, we conclude that
$\partial \Lambda _{a,-}$
is not a repeller for
$\mathcal {F}_a$
.
4 Exceptional set and periodic points
In this section, we will define a two-sided restriction of
$\mathcal {F}_a$
and prove it is a proper holomorphic map of degree 2. We will find its exceptional set and that of
$\mathcal {F}_a$
. This will be important for the next section, as it is the set of all points that may escape from the equidistribution property given in Theorem 1.2.
The following definition is classical.
Definition 4.1. Let
$f:U\to V$
be a holomorphic proper map, with
$U,V$
open,
$U\subset V$
. For
$z\in U$
, we denote by
$[ z]$
the equivalence class of z by the equivalence relation
$$ \begin{align*} w\sim z\Leftrightarrow\text{ there exist } n,m\in\mathbb{Z}^+\cup\lbrace 0\rbrace, f^n(w)=f^n(z). \end{align*} $$
We say that z is exceptional for f if
$[ z]$
is finite, and we call exceptional set the set
$\mathcal {E}$
of all points that are exceptional for f.
Put
$a\in \mathcal {K}$
and denote by
$f_a$
the two-sided restriction
, meaning
$f_a$
sends each
$z\in \mathcal {F}_a^{-1}(\Delta ^{\prime }_{\operatorname {J}_a})$
to the unique point in
$\mathcal {F}_a(z)\cap \Delta ^{\prime }_{\operatorname {J}_a}$
. This it is a single-valued, continuous, and holomorphic 2-to-1 map (see [Reference Bullett and Lomonaco4, Proposition 3.4] and Theorem 4.2 below) that extends on a neighborhood of every point
$z\in \partial \mathcal {F}_a^{-1}(\Delta _{\operatorname {J}_a})\setminus \lbrace -2\rbrace $
. In particular,
$f_a$
extends around
$z=1$
and
$f_a(1)=1$
. Since
$\Delta ^{\prime }_{\operatorname {J}_a}$
is open and
$f_a$
is continuous, then
$\mathcal {F}_a^{-1}(\Delta ^{\prime }_{\operatorname {J}_a})(=f_a^{-1}(\Delta ^{\prime }_{\operatorname {J}_a}))$
is open as well. Note that the analogous of part (2) of Remark 2.9 holds for
$\Delta ^{\prime }_{\operatorname {J}_a}$
and
$\Delta ^{\prime }_{\operatorname {Cov^Q_0}}$
instead, using the definition of Klein pair and the fact that
$\operatorname {J}_a$
is open. Thus,
$\mathcal {F}_a^{-1}(\Delta ^{\prime }_{\operatorname {J}_a})\subset \Delta ^{\prime }_{\operatorname {J}_a}$
.
The following theorem is the main result of this section.
Theorem 4.2. For each
$a\in \mathcal {K}$
, we have the following.
-
(1) The two-sided restriction
$f_a:\mathcal {F}_a^{-1}(\Delta ^{\prime }_{\operatorname {J}_a})\to \Delta ^{\prime }_{\operatorname {J}_a}$
of
$\mathcal {F}_a$
is holomorphic and proper, of degree
$2$
. -
(2) The map
$f_a$
has a critical point if and only if
$2\in \widehat {\mathbb {C}}\setminus \overline {\Delta ^{\prime }_{\operatorname {J}_a}}$
. Furthermore, in that case, we have that the critical point is
$-$
1. -
(3) The exceptional set
$\mathcal {E}_{a,-}$
of
$f_a$
is non-empty if and only if
$a=5$
. In that case,
${\mathcal {E}_{a,-}=\lbrace -1\rbrace }$
.
Computing images and preimages under
$\mathcal {F}_a$
, we see that when
$a=5$
, we have that
$\circlearrowright -1\mapsto 2\circlearrowleft $
. In the following section, it will be useful to use the full orbit of
$\mathcal {E}_{a,-}$
under
$\mathcal {F}_a$
, meaning
Proof of Theorem 4.2.
We first show part (1). Observe that
$\infty $
and
$\operatorname {J}_a(\infty )$
lie on opposite sides of
$\partial\Delta^{\prime }_{{\operatorname{J}_a}}$
. Therefore,
$(\infty ,({a+1})/{2})\notin (\mathcal {F}_a^{-1}(\Delta ^{\prime }_{\operatorname {J}_a})\times \Delta ^{\prime }_{\operatorname {J}_a})$
. In view of Proposition 2.4, this implies that for every
$$ \begin{align*}(z_0,w_0)\in\operatorname{Gr}(f_a)=(\mathcal{F}_a^{-1}(\Delta^{\prime}_{\operatorname{J}_a})\times \Delta^{\prime}_{\operatorname{J}_a})\cap\Gamma_a,\end{align*} $$
there there exists a neighborhood U of
$(z_0,w_0)$
such that
$U\cap \Gamma _a$
is the graph of a holomorphic function either in z or in w. Therefore,
$\operatorname {Gr}(f_a)$
is an open subset of
$\Gamma _a\setminus \lbrace (\infty ,({a+1})/{2})\rbrace $
, which has no singularities. Thus,
$\operatorname {Gr}(f_a)$
is a Riemann surface and
$f_a$
is holomorphic.
It is clear that
$f_a^{-1}(w)$
is compact for all
$w\in \Delta _{\operatorname {J}_a}$
, since
$$ \begin{align*}1\leq|f_a^{-1}(w)|\leq|\mathcal{F}_a^{-1}(w)|\leq 2.\end{align*} $$
Moreover,
$f_a$
is a closed map, and therefore a proper map. Indeed, let
$C\subset \mathcal {F}_a^{-1}(\Delta ^{\prime }_{\operatorname {J}_a})$
be closed. Then
$C=C'\cap \mathcal {F}_a^{-1}(\Delta ^{\prime }_{\operatorname {J}_a})$
for some closed subset
$C'\subset \widehat {\mathbb {C}}$
. Observe that
${f_a(C)=\mathcal {F}_a(C')\cap \Delta ^{\prime }_{\operatorname {J}_a}}$
and that
$$ \begin{align*}\mathcal{F}_a(C')=\pi_2(\Gamma_a\cap(C'\times\widehat{\mathbb{C}})).\end{align*} $$
By compactness of
$\widehat {\mathbb {C}}$
,
$\pi _2$
is closed, and since both
$\Gamma _a$
and
$C'\times \widehat {\mathbb {C}}$
are closed subsets of
$\widehat {\mathbb {C}}\times \widehat {\mathbb {C}}$
, then
$\mathcal {F}_a(C')$
is closed. Therefore,
$f_a(C)$
is closed in
$\Delta ^{\prime }_{\operatorname {J}_a}$
and
$f_a$
is a closed map. We conclude
$f_a$
is proper. Moreover, by definition of
$f_a$
, every preimage z of a point
$w\in \Delta ^{\prime }_{\operatorname {J}_a}$
belongs to the domain of
$f_a$
, and
$f_a(z)=w$
. Therefore,
$f_a$
has degree
$2$
, since
$\mathcal {F}_a$
has two preimages of a generic point in
$\Delta ^{\prime }_{\operatorname {J}_a}$
.
We proceed to show part (2). Let
$z_0$
be a critical point of
$f_a$
. Then
$(z_0,f_a(z_0))$
belongs to
$$ \begin{align*}A_2(\Gamma_a)\cap(\mathcal{F}_a^{-1}(\Delta^{\prime}_{\operatorname{J}_a})\times\Delta^{\prime}_{\operatorname{J}_a}).\end{align*} $$
Observe that
$\mathcal {F}_a^{-1}(\Delta ^{\prime }_{\operatorname {J}_a})\subset \Delta ^{\prime }_{\operatorname {J}_a}$
and
$1\notin \Delta ^{\prime }_{\operatorname {J}_a}$
, so
$(1,\operatorname {J}_a(-2))\notin \mathcal {F}_a^{-1}(\Delta ^{\prime }_{\operatorname {J}_a})\times \Delta ^{\prime }_{\operatorname {J}_a}$
. Since we also have that
$\infty $
and
$\operatorname {J}_a(\infty )$
lie on opposite sides of
$\partial\Delta^{\prime }_{\operatorname {J}_a}$
and from Proposition 2.4, it must be the case that
$(z_0,f_a(z_0))=(-1,\operatorname {J}_a(2))$
. Thus,
$z_0=-1$
and
${\operatorname {J}_a(2)\in \Delta ^{\prime }_{\operatorname {J}_a}}$
, and therefore
$2\in \widehat {\mathbb {C}}\setminus \overline {\Delta ^{\prime }_{\operatorname {J}_a}}$
. To prove the reverse implication, note that whenever
${2\in \widehat {\mathbb {C}}\setminus \overline {\Delta ^{\prime }_{\operatorname {J}_a}}}$
, we have that
$\operatorname {J}_a(2)={2}/({3-a})\in \Delta ^{\prime }_{\operatorname {J}_a}$
, so there exists
$z_0\in \mathcal {F}_a^{-1}(\Delta ^{\prime }_{\operatorname {J}_a})$
such that
${f_a(z_0)={2}/({3-a})}$
, by definition of
$f_a$
. Then
$z_0=-1\in \mathcal {F}_a^{-1}(\Delta ^{\prime }_{\operatorname {J}_a})$
is a critical point for
$f_a$
, as
$f_a$
has degree
$2$
and
$f_a^{-1}({2}/({3-a}))=\lbrace -1\rbrace $
. This proves that
$f_a$
has a critical point if and only if
$2\in \widehat {\mathbb {C}}\setminus \overline {\Delta ^{\prime }_{\operatorname {J}_a}}$
, and the critical point is
$z_0=-1$
.
Finally, we prove part (3). Observe that if
$w\in f_a^{-1}(z)$
, then
$[ w]=[ z]$
, so
${f_a^{-1}(\mathcal {E}_{a,-})\subset \mathcal {E}_{a,-}}$
and thus,
$|f_a^{-1}(\mathcal {E}_{a,-})|\leq |\mathcal {E}_{a,-}|$
. In particular, all points of
$\mathcal {E}_{a,-}$
must have only one preimage under
$f_a$
, and therefore, be critical. By part (2), if
$\mathcal {E}_{a,-}\neq \emptyset $
, then
$-$
1 must be fixed. We know
$f_a(-1)={2}/({3-a})$
, and therefore if
$\mathcal {E}_{a,-}\neq \emptyset $
, then
${2}/({3-a})=-1$
. Solving the equation, we get that
$a=5$
. On the other hand, if
$a=5$
, then
$2\in \widehat {\mathbb {C}}\setminus \Delta ^{\prime }_{\operatorname {J}_a}$
and therefore
$z_0=-1$
is a critical point for
$f_a$
that is fixed.
Remark 4.3. Put
$a\neq 1$
,
$|a-4|\leq 3$
, and
$(\Delta _{\operatorname {Cov^Q_0}},\Delta _{\operatorname {J}_a})$
the Klein combination pair from [Reference Bullett and Lomonaco4] described in §2.2. Away from
$z=1$
,
$\Delta ^{\prime }_{\operatorname {J}_a}$
agrees with
$\Delta _{\operatorname {J}_a}$
. Let r be the radius of the circle
$\partial \Delta _{\operatorname {J}_a}$
. Since the circle passes through a and has center
$(1+r)$
, then
$2\in \widehat {\mathbb {C}}\setminus \overline {\Delta _{\operatorname {J}_a}}$
if and only if
$r>\tfrac 12$
. Equivalently,
$a\notin \overline {B}(\tfrac 32,\tfrac 12)$
. Since
$2$
is away from
$z=1$
,
$2\in \widehat {\mathbb {C}}\setminus \overline {\Delta ^{\prime }_{\operatorname {J}_a}}$
if and only if
$a\notin \overline {B}(\tfrac 32,\tfrac 12)$
as well.
-
• If
$0<r\leq \tfrac 12$
, then
$f_a:\mathcal {F}_a^{-1}(\Delta ^{\prime }_{\operatorname {J}_a})\to \Delta ^{\prime }_{\operatorname {J}_a}$
has no critical points and hence is an unramified covering map. Since
$f_a$
is a 2-to-1 map, the Riemann–Hurwitz formula yields where
$$ \begin{align*}\chi(\mathcal{F}_a^{-1}(\Delta^{\prime}_{\operatorname{J}_a}))=2\chi(\Delta^{\prime}_{\operatorname{J}_a})=2,\end{align*} $$
$\chi $
denotes the Euler characteristic. Since
$f_a$
has degree 2,
$\mathcal {F}_a^{-1}(\Delta ^{\prime }_{\operatorname {J}_a})$
has at most two connected components, each of them with Euler characteristic at most
$1$
. It follows that
$\mathcal {F}_a^{-1}(\Delta ^{\prime }_{\operatorname {J}_a})$
has exactly two connected components, each of them homeomorphic to a disk.
-
• If
$\tfrac 12<r<3$
, then
$f_a$
is a ramified covering of degree
$2$
with one critical point at
${z_0=-1}$
, whose ramification index equals 2. Therefore, again by the Riemann–Hurwitz formula, we get that Let
$$ \begin{align*}\chi(\mathcal{F}_a^{-1}(\Delta^{\prime}_{\operatorname{J}_a}))=2\chi(\Delta^{\prime}_{\operatorname{J}_a})-(2-1)=1.\end{align*} $$
$\Omega $
be the component of
$\mathcal {F}_a^{-1}(\Delta ^{\prime }_{\operatorname {J}_a})$
that contains
$-$
1. Then
is not locally injective at
$-$
1, and therefore of degree
$2$
. Therefore,
$\mathcal {F}_a^{-1}(\Delta ^{\prime }_{\operatorname {J}_a})=\Omega $
has one connected component, which is homeomorphic to a circle, and mapped 2-to-1 onto
$\Delta ^{\prime }_{\operatorname {J}_a}$
.
We finish this section by listing some properties of the periodic points of
$\mathcal {F}_a$
. To do so, recall that for a holomorphic correspondence
$F$
on
$X$
,
$$ \begin{align*}\operatorname{Per}_n(F)=\pi_1(\Gamma^{(n)}\cap\mathfrak{D}_{X}),\end{align*} $$
where
$\Gamma ^{(n)}$
is the graph of
$F^n$
and
$\mathfrak {D}_{X}$
is the diagonal in
$X\times X$
. Note that
$$ \begin{align*}\operatorname{Per}_n(F)=\lbrace z\in X|z\in F^n(z)\rbrace=\lbrace z\in X|z\in F^{-n}(z)\rbrace.\end{align*} $$
Lemma 4.4. For every
$a\in \mathcal {K}$
, we have that:
-
(1)
$\operatorname {Per}_n(\mathcal {F}_a)\subset \Lambda _{a,-}\cup \Lambda _{a,+}$
; -
(2)
$\operatorname {J}_a(\operatorname {Per}_n(\mathcal {F}_a))=\operatorname {Per}_n(\mathcal {F}_a)$
; -
(3)
$\operatorname {J}_a(\operatorname {Per}_n(\mathcal {F}_a)\cap \Lambda _{a,\pm })=\operatorname {Per}_n(\mathcal {F}_a)\cap \Lambda _{a,\mp }$
; and -
(4)
$\operatorname {Per}_n(\mathcal {F}_a)\cap \Lambda _{a,-}=\operatorname {Per}_n(f_a)$
.
Proof. To prove part
$(1)$
, suppose
$z\in \operatorname {Per}_n(\mathcal {F}_a)$
. If
$z\in \overline {\Delta ^{\prime }_{\operatorname {J}_a}}$
, then
$z\in \mathcal {F}_a^{-kn}(z)\subset \mathcal {F}_a^{-kn}(\overline {\Delta ^{\prime }_{\operatorname {J}_a}})$
for all
$k\geq 1$
. Since the sets
$\mathcal {F}_a^{-k}(\overline {\Delta ^{\prime }_{\operatorname {J}_a}})$
are nested and their intersection is
$\Lambda _{a,-}$
, then
$z\in \Lambda _{a,-}$
. On the other hand, if
$z\notin \Lambda _{a,-}$
, by Lemma 3.6 part
$(3)$
, there exists
$m\geq 1$
so that
$\mathcal {F}_a^m(z)\subset \widehat {\mathbb {C}}\setminus \Delta ^{\prime }_{\operatorname {J}_a}$
. A similar argument as above shows that for all k sufficiently large,
$z\in \mathcal {F}_a^k(\widehat {\mathbb {C}}\setminus \overline {\Delta ^{\prime }_{\operatorname {J}_a}})$
, which implies that
$z\in \Lambda _{a,+}$
.
Next, note that if
$z\in \operatorname {Per}_n(\mathcal {F}_a)$
, then
$z\in \mathcal {F}_a^{-n}(z)$
and
$\operatorname {J}_a(z)\in \operatorname {J}_a(\mathcal {F}_a^{-n}(z))=\mathcal {F}_a^n(\operatorname {J}_a(z))$
. Therefore,
$\operatorname {J}_a(z)\in \operatorname {Per}_n(\mathcal {F}_a)$
as well. Since
$\operatorname {J}_a$
is an involution, this shows part
$(2)$
.
Recall from Remark 3.6 that
$\operatorname {J}_a$
sends the limits sets to each other. Therefore,
$\operatorname {J}_a(\operatorname {Per}_n(\mathcal {F}_a)\cap \Lambda _{a,-})=\operatorname {Per}_n(\mathcal {F}_a)\cap \Lambda _{a,+}$
, and again since
$\operatorname {J}_a$
is an involution, we also have that
$$ \begin{align*}\operatorname{J}_a(\operatorname{Per}_n(\mathcal{F}_a)\cap\Lambda_{a,+})=\operatorname{Per}_n(\mathcal{F}_a)\cap\Lambda_{a,-}.\end{align*} $$
This proves part
$(3)$
.
Finally, we prove part
$(4)$
. We have that for each
$z\in \mathcal {F}_a^{-1}(\Delta ^{\prime }_{\operatorname {J}_a})\cup \lbrace 1\rbrace $
,
$\mathcal {F}_a^{-n}(z)=f_a^{-n}(z)$
and part
$(1)$
implies that all periodic points of
$\mathcal {F}_a$
not in
$\Lambda _{a,+}$
must belong to
$\Lambda _{a,-}$
. Since
$z\in \operatorname {Per}_n(f_a)$
if and only if
$z\in f_a^{-n}(z)=\mathcal {F}_a^{-1}(z)$
, then
$$ \begin{align*}\operatorname{Per}_n(\mathcal{F}_a)\cap\Lambda_{a,-}=\operatorname{Per}_n(\mathcal{F}_a)\cap(\mathcal{F}_a^{-1}(\Delta^{\prime}_{\operatorname{J}_a})\cup\lbrace 1\rbrace)=\operatorname{Per}_n(f_a).\\[-38pt]\end{align*} $$
5 Equidistribution of
$\lbrace \mathcal {F}_a^n\rbrace _n$
In this section, we prove Theorems 1.2 and 1.3 using the results from [Reference Freire, Lopes and Mañé20, Reference Ljubich24], together with [Reference Bullett and Lomonaco4, Reference Bullett and Lomonaco5].
Recall every rational map
$P_A$
of the form
$$ \begin{align} P_A(z)=z+1/z+A, \end{align} $$
with
$A\in \mathbb {C}$
, has critical points
$\pm 1$
and a parabolic fixed point at
$\infty $
with multiplier equal to
$1$
. Now note that in the coordinate
$\phi (z)=1/z$
, we have that
$$ \begin{align*}P_A^{\phi}(z)=\frac{z}{z^2+Az+1}=z-Az^2+(A^2-1)z^3-\cdots\end{align*} $$
near
$0$
. Thus,
$z=0$
is a fixed point of
$P_A^{\phi }$
with multiplicity
$2$
if
$A\neq 0$
, and
$3$
if
$A=0$
. We conclude that for
$A\neq 0$
, the parabolic fixed point has multiplicity
$1$
, and
$3$
for
$A=0$
.
For
$A\in \mathbb {C}\setminus \lbrace 0\rbrace $
, let
$\Omega _A$
be the basin of attraction of
$\infty $
, and for
$A=0$
, we make the choice
$\Omega _0=\lbrace x+iy| x,y\in \mathbb {R},x>0\rbrace $
. We define the filled Julia set of
$P_A$
as the set
$$ \begin{align*}K_{P_A}=\widehat{\mathbb{C}}\setminus\Omega_A.\end{align*} $$
Remark 5.1. No periodic points of
$P_A$
live in
$\Omega _A$
. Indeed, it is clear that points in
$\Omega _A$
for
$A\neq 0$
cannot be periodic, as their iterates converge to
$\infty $
. Moreover, it is easy to check that if
$\Re (z)$
denotes the real part of
$z\neq 0$
, then
$\Re (P_A(z))=\Re (z)({|z|^2+1})/{|z|^2}$
. Then
$\Omega _0$
is completely invariant and has no periodic points. Therefore, for all
$A\in \mathbb {C}$
, we have that
$\operatorname {Per}_n(P_A)\subset K_{P_A}$
, for all
$n\geq 1$
.
Lemma 5.2. For every
$a\in \mathcal {K}$
, there exists a measure
$\mu _-$
with
$\operatorname {supp}(\mu _-)=\partial \Lambda _{a,-}$
such that
$$ \begin{align*}\frac{1}{2^n}(f_a^n)^*\delta_{z_0}\to\mu_-\end{align*} $$
weakly, for all
$z_0\in \Delta _{\operatorname {J}_a}\setminus \mathcal {E}_{a,-}$
.
Here,
$\mathcal {E}_{a,-}$
is the exceptional set of the two-sided restriction
$f_a$
of
$\mathcal {F}_a$
that leaves
$\Lambda _{a,-}$
invariant, described in §4.
Proof. From the previous section, we can see that the two-sided restriction
$f_a$
can extend around
$1$
. In [Reference Bullett and Lomonaco4, Proposition 5.2], the authors proved the existence of closed topological disks
$V^{\prime }_a$
and
$V_a$
containing
$\Lambda _{a,-}$
with
$V^{\prime }_a=\mathcal {F}_a^{-1}(V_a)\subset V_a$
and satisfying most properties for
$f_a$
to be a parabolic-like map from
$V^{\prime }_a$
and
$V_a$
. Moreover, from the proof of [Reference Bullett and Lomonaco4, Theorem B], there exists a neighborhood U of
$\Lambda _{a,-}$
,
$A\in\mathbb{C}$
and a quasiconformal map
${h:U\cap V^{\prime }_a\cap \to h(U)\cap V_a}$
such that
$h\circ f=P_A\circ h$
, where
$P_A$
is as in equation (16). Such conjugacy sends
$\Lambda _{a,-}$
onto the filled Julia set
$K_{P_A}$
of
$P_A$
.
From [Reference Freire, Lopes and Mañé20, Reference Ljubich24], there exists a measure
$\tilde {\mu }_A$
on
$\widehat {\mathbb {C}}$
supported on the Julia set
$\mathcal {J}_{P_A}$
of
$P_A$
, such that for all
$z_0\in \widehat {\mathbb {C}}$
not in the exceptional set E of
$P_A$
,
$(1/2^n)(P_A^n)^*\delta _{z_0}$
is weakly convergent to
$\tilde {\mu }_A$
.
Recall
$\Lambda _{a,-}$
is the intersection of the nested compact sets
$\mathcal {F}_a^{-1}(\overline {\Delta ^{\prime }_{\operatorname {J}_a}})$
. Thus, there exists
$N\in \mathbb {N}$
such that
$\mathcal {F}_a^n(\overline {\Delta ^{\prime }_{\operatorname {J}_a}})\subset U\cap V^{\prime }_a$
, for all
$n\geq N$
. Observe as well that
$h^{-1}(E)=\mathcal {E}_{a,-}$
as elements in
$\mathcal {E}_{a,-}$
must have only one preimage and h is a homeomorphism, and the analogous holds for E and
$h^{-1}$
.
Now take
$z_0\in \overline {\Delta ^{\prime }_{\operatorname {J}_a}}\setminus \mathcal {E}_{a,-}$
. Then,
$f_a^{-N}(z_0)=\mathcal {F}_a^{-N}(z_0)\subset U\cap V^{\prime }_a$
. Then for each
${\zeta \in f_a^{-N}(z_0)}$
, we have that
$h(\zeta )\notin E$
and
$(1/2^n)(P_A^n)^*\delta _{h(\zeta )}$
is weakly convergent to
$\tilde {\mu }_A$
. Note that
$\delta _{h(\zeta )}=h_*\delta _{\zeta }$
, and therefore for
$n\geq N$
,
$$ \begin{align*}\frac{1}{2^n}(P_A^n)^*\delta_{h(\zeta)}=\frac{1}{2^n}(h^{-1}\circ P_A^n)^*\delta_{\zeta}=\frac{1}{2^n}(f_a^n\circ h^{-1})^*\delta_{\zeta}=h_*\bigg(\frac{1}{2^n}(f_a^n)^*\delta_{\zeta}\bigg).\end{align*} $$
Since the left-hand side is weakly convergent to
$\tilde {\mu }_A$
, then
$(1/2^n)(f_a^n)^*\delta _{\zeta }$
is weakly convergent to
, which is supported on
$h^{-1}(\mathcal {J}_{P_A})=\partial \Lambda _{a,-}$
. Thus,
$$ \begin{align*}\frac{1}{2^n}(f_a^n)^*\delta_{z_0}=\frac{1}{2^N}\sum\limits_{\zeta\in f_a^{-N}(z_0)}\nu_{f_a^N}(\zeta)\frac{1}{2^{n-N}}(f_a^{n-N})^*\delta_{\zeta}\end{align*} $$
is weakly convergent to
$\mu _-$
as desired.
Proof of Theorem 1.2.
Let
$\mu _-$
be as in Lemma 5.2 and put
. Clearly,
$\operatorname {supp}(\mu _+)=\partial \Lambda _{a,+}$
. Denote by
$\delta _{z_0}$
the Dirac measure at
$z_0$
. If
$z_0\in \widehat {\mathbb {C}}\setminus (\Lambda _{a,-}\cup \mathcal {E}_a)$
, there exists
$n_0\in \mathbb {Z}^+\cup \lbrace 0\rbrace $
such that
${\mathcal {F}_a^{n_0}(z_0)\cap \overline {\Delta ^{\prime }_{\operatorname {J}_a}}=\emptyset }$
by part
$(3)$
of Lemma 3.6. In particular, this gives us that
$\mathcal {F}_a^{n_0}(z_0)$
is contained in
$\widehat {\mathbb {C}}\setminus \Delta ^{\prime }_{\operatorname {J}_a}$
, and therefore
${\mathcal {F}_a^n(z_0)\subset \widehat {\mathbb {C}}\setminus \Delta ^{\prime }_{\operatorname {J}_a}}$
, for all
${n\geq n_0}$
. In addition, for every
$z_j\in \mathcal {F}_a^{n_0}(z_0)$
,
$\operatorname {J}_a(z_j)\notin \mathcal {E}_{a,-}$
. Thus, for such a
$z_0$
, we get that
weakly, as
$n\to \infty $
, where
$\Gamma ^{(n_0)}$
is the graph of
$\mathcal {F}_a^{n_0}$
.
Now observe that the two-sided restriction
$f_a$
of
$\mathcal {F}_a$
sends
$\Lambda _{a,-}$
to itself. Indeed, note that the sets
$\mathcal {F}_a^{-n}(\overline {\Delta ^{\prime }_{\operatorname {J}_a}})$
are nested and if
$f_a(z)\notin \mathcal {F}_a^{-n}(\overline {\Delta ^{\prime }_{\operatorname {J}_a}})$
for some
$n\in \mathbb {Z}^+$
, then
${z\notin \mathcal {F}_a^{n-1}(\overline {\Delta ^{\prime }_{\operatorname {J}_a}})\supset \Lambda _{a,-}}$
. Note as well that
$-2\in \mathcal {F}_a^{-1}(1)$
and
$1\in \Lambda _{a,-}$
, so
${-2\in \Lambda _{a,-}}$
. Define
$\tilde {f_a}:\Lambda _{a,-}\to (\widehat {\mathbb {C}}\setminus \overline {\Delta ^{\prime }_{\operatorname {J}_a}})\cup \lbrace 1\rbrace $
by
$\tilde {f_a}(z)=w$
, where
$\mathcal {F}_a(z)\setminus \lbrace f_a(z)\rbrace =\lbrace w\rbrace $
for
${z\neq -2}$
, and
$\tilde {f_a}(-2)=f_a(-2)=1$
for
$z\in \mathcal {F}_a^{-1}(1)\setminus \lbrace 1\rbrace $
. Then for
$z_0\in \Lambda _{a,-}\setminus \mathcal {E}_a$
, we have that
$\mathcal {F}_a(z_0)=\lbrace f_a(z_0),\tilde {f_a}(z_0)\rbrace $
, and
$$ \begin{align*} \frac{1}{2}(\mathcal{F}_a)_*\delta_{z_0} &= \frac{1}{2}\delta_{f_a(z_0)}+\frac{1}{2}\delta_{\tilde{f_a}(z_0)},\\ \frac{1}{4}(\mathcal{F}_a^2)_*\delta_{z_0} &= \frac{1}{4}\delta_{{f_a}^2(z_0)}+\frac{1}{4}\delta_{\tilde{f_a}\circ f_a(z_0)}+\frac{1}{2}(\mathcal{F}_a)_*\delta_{\tilde{f_a}(z_0)},\\ \frac{1}{8}(\mathcal{F}_a^3)_*\delta_{z_0} &= \frac{1}{8}\delta_{{f_a}^3(z_0)}+\frac{1}{8}\delta_{\tilde{f_a}\circ {f_a}^2(z_0)}+\frac{1}{4}(\mathcal{F}_a)_*\delta_{\tilde{f_a}\circ f_a(z_0)}+\frac{1}{2}(\mathcal{F}_a^2)_*\delta_{\tilde{f_a}(z_0)},\\ &\ \ \vdots \\ \frac{1}{2^n}(\mathcal{F}_a^n)_*\delta_{z_0} &= \frac{1}{2^n}\delta_{{f_a}^n(z_0)}+\sum\limits_{j=1}^n \frac{1}{2^j}(\mathcal{F}_a^{n-j})_*\delta_{\tilde{f_a}\circ {f_a}^{j-1}(z_0)}. \end{align*} $$
Since
$(1/2^n)\delta _{{f_a}^n(z_0)}$
has mass
${1}/{2^n}$
, then it is weakly convergent to a measure with zero mass. Put
If we truncate the sum defining
$\mu _n$
at
$N<n$
, we obtain a sequence
$\mu _n^{(N)}=\sum \nolimits _{j=1}^N\mu _{n,j}$
satisfying
$\mu _n^{(N)}\to \sum \nolimits _{j=1}^N(1/2^j)\mu _+=(1-2^{-N})\mu _+$
weakly. Let
$\varphi :\widehat {\mathbb {C}}\to \mathbb {R}$
be continuous and
$\varepsilon>0$
. Choose
$N\in \mathbb {Z}^+$
big so that
$(3/2^N)\sup |\varphi |<\varepsilon $
, and
$n>N$
such that
$$ \begin{align*}\bigg|\int\varphi \,d\mu_n^{(N)}-(1-2^{-N})\int\varphi \,d\mu_+\bigg|<\frac{1}{2^N}\sup|\varphi|\end{align*} $$
by the convergence
$\mu _n^{(N)}\to (1-2^{-N})\mu _+$
. Then,
$$ \begin{align*} \bigg|\int\varphi \,d\mu_n^{(N)}-\int\varphi \,d\mu_+\bigg|&\leq \bigg|\int\varphi \,d\mu_n^{(N)}-\bigg(1-\frac{1}{2^N}\bigg)\int\varphi \,d\mu_+\bigg|+\frac{1}{2^N}\bigg|\int\varphi \,d\mu_+\bigg|\\ &\leq \frac{1}{2^{N-1}}\sup|\varphi|. \end{align*} $$
Since
$\mu _n-\mu _n^{(N)}=\sum \nolimits _{j=N+1}^n\mu _{n,j}$
has mass at most
${1}/{2^N}$
, we get
$$ \begin{align*} \bigg|\int\varphi \,d\mu_n-\int\varphi \,d\mu_+\bigg|&\leq \bigg|\int\varphi \,d\mu_n-\int\varphi \,d\mu_n^{(N)}\bigg|+\bigg|\int\varphi \,d\mu_n^{(N)}-\int\varphi \,d\mu_+\bigg|\\ &\leq \frac{3}{2^N}\sup|\varphi|<\varepsilon. \end{align*} $$
This proves that for all
$\varphi :\widehat {\mathbb {C}}\to \mathbb {R}$
, we have that
$\int \varphi \,d\mu _n\to \int \varphi \,d\mu _+$
as
$n\to \infty $
and hence
$\mu _n\to \mu _+$
. Since
$(1/2^n)(\mathcal {F}_a^n)_*\delta _{z_0}=(1/2^n)\delta _{{f_a}^n(z_0)}+\mu _n$
, we obtain that
$(1/2^n)(\mathcal {F}_a^n)_*\delta _{z_0}$
is weakly convergent to
$\mu _+$
, as desired.
Applying again the push-forward by
$\operatorname {J}_a$
, we get that for every
$z_0\notin \mathcal {E}_a$
,
$(1/2^n)(\mathcal {F}_a^n)^*\delta _{z_0}$
is weakly convergent to
$\mu _-$
.
Now, we show the asymptotic equidistribution of periodic points of
$\mathcal {F}_a$
of order n with respect to the probability measure
$\tfrac 12(\mu _-+\mu _+)$
.
Proof of Theorem 1.3.
From Lemma 4.4, we have that all periodic points lie in
${\Lambda _{a,-}\cup \Lambda _{a,+}}$
and
Denote this number by
$d_n$
. Since
$1\in \mathcal {F}_a(1)$
and Lemma 4.4 says
$\Lambda _{a,-}\cap \Lambda _{a,+}=\lbrace 1\rbrace $
, then
$$ \begin{align*}(\operatorname{Per}_n(\mathcal{F}_a)\cap\Lambda_{a,-})\cap(\operatorname{Per}_n(\mathcal{F}_a)\cap\Lambda_{a,+})=\lbrace 1\rbrace.\end{align*} $$
Thus,
. Since the conjugacy h between
$f_a$
and the quadratic rational map
$P_A$
sends
$\Lambda _{a,-}$
onto the filled Julia set
$K_{P_A}$
of
$P_A$
, which contains all periodic points of
$P_A$
by Remark 5.1,
$\operatorname {Per}_n(P_A)=h(\operatorname {Per}_n(\mathcal {F}_a)\cap \Lambda _{a,-})$
and
as well. We have that
$\lim \nolimits _{n\to \infty }d_n=\infty $
(see in [Reference Ljubich24, p. 363]). Then,
$$ \begin{align*} \frac{1}{2d_n-1}\sum\limits_{z\in\operatorname{Per}_n(\mathcal{F}_a)\cap\Lambda_{a,-}}\delta_z&= \frac{1}{2d_n-1}\sum\limits_{z\in\operatorname{Per}_n(f_a)}\delta_z\\ &= h^*\bigg(\frac{1}{2d_n-1}\sum\limits_{z\in\operatorname{Per}_n(f_a)}\delta_{h(z)}\bigg)\\ &= h^*\bigg(\frac{d_n}{2d_n-1}\frac{1}{d_n}\sum\limits_{\zeta\in\operatorname{Per}_n(P_A)}\delta_{\zeta}\bigg), \end{align*} $$
which is weakly convergent to
$\tfrac 12h^*\widetilde {\mu }_A=\tfrac 12\mu _-$
by the corollary to Theorem 3 in [Reference Ljubich24]. Thus,
$$ \begin{align*} \frac{1}{2d_n-1}\sum\limits_{z\in\operatorname{Per}_n(\mathcal{F}_a)\cap\Lambda_{a,+}}\delta_z= \operatorname{J}_a^*\bigg(\frac{1}{2d_n-1}\sum\limits_{\operatorname{J}_a(z)\in \operatorname{Per}_n(\mathcal{F}_a)\cap\Lambda_{a,-}}\delta_{\operatorname{J}_a(z)}\bigg) \end{align*} $$
is weakly convergent to
$\tfrac 12\operatorname {J}_a^*\mu _-=\tfrac 12\mu _+$
, and
converges weakly to
$\tfrac 12(\mu _-+\mu _+)$
, as desired.
Finally, we proceed to show that the equidistribution still holds when counting with multiplicity. Observe that the only points of
$\Lambda _{a,-}$
that are not in the interior of the topological conjugacy h are
$-$
2 and
$1$
, and we have that
$-$
2 is not a periodic point for
$\mathcal {F}_a$
. We claim that for every
$z_0\in \operatorname {Per}_n({f_a})\setminus \lbrace 1\rbrace $
, we have that
Indeed, the topological conjugacy h sends attracting periodic points to attracting periodic points, so if
$|(f_a^n)'(z_0)|<1$
, then
$|(P^n_A)'(h(z_0))|<1$
as well. Thus, both
$z_0$
and
$h(z_0)$
have multiplicity
$1$
as a periodic point in this case. The same happens for
$|(f_a^n)'(z_0)|>1$
. Finally, if
$|(f_a^n)'(z_0)|=|(P^n_A)'(h(z_0))|=1$
, Naishul’s theorem [Reference Naishul27] (later re-proven by Pérez-Marco in [Reference Pérez-Marco28]) shows that
$(f_a^n)'(z_0)=(P^n_A)'(h(z_0))$
. Moreover, attracting directions are preserved under topological conjugacy, so the parabolic fixed point
$z_0$
of
$\mathcal {F}_a$
and the parabolic fixed point
$h(z_0)$
of
$P_A$
have the same number of attracting directions. Therefore, both
$z_0$
and
$h(z_0)$
have the same multiplicity as periodic points of order n of
$f_a$
and
$P_A$
, respectively. Therefore,
From Lemma 4.4 part
$(2)$
and given that
$\operatorname {J}_a$
is a Mobius transformation, we have that
as well.
Since the multiplicity of a periodic point is the multiplicity as a periodic point of its minimal period and since
$\phi ^{-1}(0)=\infty $
, we have that
Similarly, from the equations for
$g^{\psi }$
listed in Remark 3.5, we have that
In [Reference Bullett and Lomonaco5, Corollary 4.3], the authors showed that for
$a=7$
, the member of the family of quadratic rational maps
$$ \begin{align*}\lbrace P_A(z)=z+1/z+A|A\in\mathbb{C}\rbrace\end{align*} $$
that is conjugate to
$f_a$
is
$P_0$
. Therefore, for all
$a\in \mathbb {C}\setminus \lbrace 1\rbrace $
,
$|a-4|\leq 3$
,
and we have that
which also converges weakly to
$\mu _-$
by [Reference Ljubich24, Theorem 3]. Using that
$\operatorname {J}_a$
preserves multiplicities and that
$\operatorname {J}_a^*\mu _-=\mu _+$
, we have that
is weakly convergent to
$\mu _+$
. Since
has total mass
${1}/{2^n}$
and
$$ \begin{align*}\operatorname{Per}_n(\mathcal{F}_a)=\lbrace 1\rbrace\cup(\operatorname{Per}_n(\mathcal{F}_a)\cap\Lambda_{a,-}\setminus\lbrace 1\rbrace)\cup(\operatorname{Per}_n(\mathcal{F}_a)\cap\Lambda_{a,+}\setminus\lbrace 1\rbrace)\end{align*} $$
is a disjoint union, then
is weakly convergent to
$\tfrac 12(\mu _-+\mu _+)$
.
In what follows, we will introduce terminology and prove Theorem 1.4.
Definition 5.3. A holomorphic correspondence F with graph
$\Gamma $
is said to be postcritically finite if for all
$w\in B_2(\Gamma )$
, there exist
$0\leq m<n$
so that
$$ \begin{align} F^m(w)\cap F^n(w)\neq\emptyset. \end{align} $$
Equivalently, for every
$w\in B_2(\Gamma )$
, there exists
$m\geq 0$
and
$z\in F^m(w)$
so that
${z\in \operatorname {Per}_n(F)}$
for some
$n\geq 1$
. This definition generalizes that of postcritically finite rational maps. Indeed, if F is a rational map that is postcritically finite as a correspondence, then we have that every
$w\in B_2(\Gamma )$
is pre-periodic. Since
$B_2(\Gamma )=F(\operatorname {CritPt}(F))$
, then every critical point is pre-periodic as well. Hence, F is postcritically finite as a rational map.
If F is a holomorphic correspondence and
$z_0\in \operatorname {Per}_n(F)$
, there exists a cycle
$$ \begin{align} (z_0,z_1,z_2,\ldots,z_{n-1}) \end{align} $$
so that
$z_i\in F(z_{i-1})$
and
$z_0\in F(z_{n-1})$
.
Definition 5.4. We say that a holomorphic correspondence with graph
$\Gamma $
is superstable if there exists
$\alpha \in A_2(\Gamma )$
and a cycle in equation (18) satisfying that
$z_0=\pi _1(\alpha )$
and
${z_1=\pi _2(\alpha )}$
.
Observe that if F is a rational map, then
$\pi _1(\alpha )$
corresponds to a critical point and
$\pi _2(\alpha )$
corresponds to its critical value. Therefore, the definition of superstable correspondences reduces to F having a superstable cycle, meaning a cycle containing a critical point.
In the context of the family
$\lbrace \mathcal {F}_a\rbrace _{a\in \mathcal {K}}$
, Proposition 2.4 says that
$$ \begin{align*}B_2(\Gamma_a)=\bigg\lbrace \frac{a+1}{2},\frac{4a+2}{a+5},\frac{2}{3-a}\bigg\rbrace.\end{align*} $$
Observe that
$({a+1})/{2}=\operatorname {J}_a(\infty )\notin \Lambda _{a,-}\cup \Lambda _{a,+}$
. Indeed, since
$\infty $
is fixed by
$\operatorname {Cov^Q_0}$
and
$(\Delta ^{\prime }_{\operatorname {Cov^Q_0}},\Delta ^{\prime }_{\operatorname {J}_a})$
is a Klein combination pair, then
$\infty \in (\widehat {\mathbb {C}}\setminus \Delta ^{\prime }_{\operatorname {Cov^Q_0}})\setminus \lbrace 1\rbrace \subset \Delta ^{\prime }_{\operatorname {J}_a}$
, so
${\operatorname {J}_a(\infty )\notin \Lambda _{a,-}\subset \overline {\Delta ^{\prime }_{\operatorname {J}_a}}}$
. On the other hand,
$\mathcal {F}_a^{-1}(\operatorname {J}_a(\infty ))=\lbrace \infty \rbrace $
does not intersect
$\Lambda _{a,+}$
, so
${\operatorname {J}_a(\infty )\notin \Lambda _{a,+}}$
. By Lemma (4.4),
$({a+1})/{2}$
cannot satisfy equation (17). Therefore, there are no parameters
$a\in \mathcal {K}$
for which
$\mathcal {F}_a$
is postcritically finite.
However,
and
, so
$({4a+2})/({a+5})$
is not periodic. Therefore,
$\mathcal {F}_a$
is superstable if and only if
${2}/({3-a})$
is periodic. Since
$\mathcal {F}_a^{-1}({2}/({3-a}))=\lbrace -1\rbrace $
and
$\mathcal {F}_a^{-1}=f_a^{-1}$
, then
$\mathcal {F}_a$
is superstable if and only if
$-1$
is a critical point of
$f_a$
that is periodic. We say that the parameter
$a\in \mathcal {K}$
is superstable whenever
$\mathcal {F}_a$
is superstable.
We consider the quadratic family
$$ \begin{align*}\lbrace p_c(z)=z^2+c|c\in\mathbb{C}\rbrace\end{align*} $$
and let
be the set of superstable parameters of order n. Note that
$\hat {P}_n$
has
$2^{n-1}$
points, counted with multiplicity, and that it is contained in the Mandelbrot set
$\mathcal {M}$
. Levin proved in [Reference Levin23] that
$$ \begin{align*}\lim\limits_{n\to\infty}\frac{1}{2^{n-1}}\sum\limits_{c\in\hat{P}_n}\delta_{c}\end{align*} $$
converges to a measure
$m_{\operatorname {BIF}}$
on
$\mathcal {M}$
, which we call the bifurcation measure.
Let
$\mathcal {M}_{\Gamma }$
denote the connectedness locus of the family
$\lbrace \mathcal {F}_a\rbrace _{a\in \mathcal {K}}$
and
$\mathcal {M}_1$
denote the parabolic Mandelbrot set. The conjugacy shown in [Reference Bullett and Lomonaco4] between
$f_a$
and
$P_A$
induces a map
$a\mapsto 1-A^2$
from
$\mathcal {M}_{\Gamma }$
and
$\mathcal {M}_1$
. From [Reference Bullett and Lomonaco5, Main Theorem], that map is a homeomorphism. On the other hand, [Reference Petersen and Roesch29, Main Theorem] says that
$\mathcal {M}_1$
is homeomorphic to
$\mathcal {M}$
. Moreover, both homeomorphisms constructed preserve the type of dynamics associated to the parameter (see [Reference Petersen and Roesch29, §1]). Therefore, there is a homeomorphism
$\Psi :\mathcal {M}\to \mathcal {M}_{\Gamma }$
that gives a one-to-one correspondence between superstable parameters of
$\mathcal {M}_{\Gamma }$
and superstable parameters of
$\mathcal {M}$
. Pushing the bifurcation measure forward through
$\Psi $
, and writing
$$ \begin{align*}\hat{P}^{\Gamma}_n=\lbrace a\in\mathcal{K}\mbox{ superstable }|f_a^n(-1)=-1\rbrace,\end{align*} $$
we obtain the statement in Theorem 1.4.
Acknowledgements
The author would like to thank Shaun Bullett and Luna Lomonaco for a useful conversation in Santiago de Chile, and especially thank Juan Rivera-Letelier for his great patience and guidance.
Open access
