Article contents
Endperiodic automorphisms of surfaces and foliations
Published online by Cambridge University Press: 07 October 2019
Abstract
We extend the unpublished work of Handel and Miller on the classification, up to isotopy, of endperiodic automorphisms of surfaces. We give the Handel–Miller construction of the geodesic laminations, give an axiomatic theory for pseudo-geodesic laminations, show that the geodesic laminations satisfy the axioms, and prove that pseudo-geodesic laminations satisfying our axioms are ambiently isotopic to the geodesic laminations. The axiomatic approach allows us to show that the given endperiodic automorphism is isotopic to a smooth endperiodic automorphism preserving smooth laminations ambiently isotopic to the original ones. Using the axioms, we also prove the ‘transfer theorem’ for foliations of 3-manifolds, namely that, if two depth-one foliations 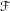 ${\mathcal{F}}$ and
${\mathcal{F}}$ and 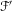 ${\mathcal{F}}^{\prime }$ are transverse to a common one-dimensional foliation
${\mathcal{F}}^{\prime }$ are transverse to a common one-dimensional foliation  ${\mathcal{L}}$ whose monodromy on the non-compact leaves of
${\mathcal{L}}$ whose monodromy on the non-compact leaves of 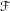 ${\mathcal{F}}$ exhibits the nice dynamics of Handel–Miller theory, then
${\mathcal{F}}$ exhibits the nice dynamics of Handel–Miller theory, then 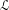 ${\mathcal{L}}$ also induces monodromy on the non-compact leaves of
${\mathcal{L}}$ also induces monodromy on the non-compact leaves of 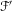 ${\mathcal{F}}^{\prime }$ exhibiting the same nice dynamics. Our theory also applies to surfaces with infinitely many ends.
${\mathcal{F}}^{\prime }$ exhibiting the same nice dynamics. Our theory also applies to surfaces with infinitely many ends.
Keywords
MSC classification
- Type
- Original Article
- Information
- Copyright
- © Cambridge University Press, 2019
References
- 3
- Cited by



