Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-04T19:22:23.703Z Has data issue: false hasContentIssue false
  • English
  • Français

Dynamics of homeomorphisms of the torus homotopic to Dehn twists

Published online by Cambridge University Press:  15 January 2013

SALVADOR ADDAS-ZANATA ,
FÁBIO A. TAL  and
BRÁULIO A. GARCIA
SALVADOR ADDAS-ZANATA
Affiliation:
Instituto de Matemática e Estatística, Universidade de São Paulo, Rua do Matão 1010, Cidade Universitária, 05508-090 São Paulo, SP, Brazil (email: [email protected], [email protected], [email protected])
FÁBIO A. TAL
Affiliation:
Instituto de Matemática e Estatística, Universidade de São Paulo, Rua do Matão 1010, Cidade Universitária, 05508-090 São Paulo, SP, Brazil (email: [email protected], [email protected], [email protected])
BRÁULIO A. GARCIA
Affiliation:
Instituto de Matemática e Estatística, Universidade de São Paulo, Rua do Matão 1010, Cidade Universitária, 05508-090 São Paulo, SP, Brazil (email: [email protected], [email protected], [email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper, we consider torus homeomorphisms 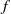$f$ homotopic to Dehn twists. We prove that if the vertical rotation set of 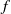$f$ is reduced to zero, then there exists a compact connected essential ‘horizontal’ set 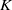$K$, invariant under $f$. In other words, if we consider the lift 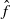$\hat {f}$ of 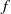$f$ to the cylinder, which has zero vertical rotation number, then all points have uniformly bounded motion under iterates of 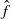$\hat {f}$. Also, we give a simple explicit condition which, when satisfied, implies that the vertical rotation set contains an interval and thus also implies positive topological entropy. As a corollary of the above results, we prove a version of Boyland’s conjecture to this setting: if 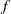$f$ is area preserving and has a lift 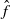$\hat {f}$ to the cylinder with zero Lebesgue measure vertical rotation number, then either the orbits of all points are uniformly bounded under $\hat {f}$, or there are points in the cylinder with positive vertical velocity and others with negative vertical velocity.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 34 , Issue 2 , April 2014 , pp. 409 - 422
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1]Addas-Zanata, S.. On the existence of a new type of periodic and quasi-periodic orbits for twist maps of the torus. Nonlinearity 15 (2002), 1399–1416.CrossRefGoogle Scholar
[2]Addas-Zanata, S.. On properties of the vertical rotation interval for twist mappings. Ergod. Th. & Dynam. Sys. 25 (2005), 641–660.Google Scholar
[3]Addas-Zanata, S.. Some extensions of the Poincaré–Birkhoff theorem to the cylinder and a remark on mappings of the torus homotopic to Dehn twists. Nonlinearity 18 (2005), 2243–2260.Google Scholar
[4]Addas-Zanata, S. and Tal, F. A.. Homeomorphisms of the annulus with a transitive lift. Math. Z. 267 (2009), 971–980.Google Scholar
[5]Birkhoff, G.. Nouvelles recherches sur les systèmes dynamiques. Collec. Math. Pap. 2 (1935), 530–661.Google Scholar
[6]Doeff, E.. Rotation measures for homeomorphisms of the torus homotopic to a Dehn twist. Ergod. Th. & Dynam. Sys. 17 (1997), 1–17.Google Scholar
[7]Doeff, E. and Misiurewicz, M.. Shear rotation numbers. Nonlinearity 10 (1997), 1755–1762.CrossRefGoogle Scholar
[8]Franks, J.. Generalizations of the Poincaré–Birkhoff theorem. Ann. of Math. (2) 128 (1988), 139–151.Google Scholar
[9]Le Roux, F.. Homéomorphismes de surfaces: théorèmes de la fleur de Leau-Fatou et de la variété stable. Astérisque 292 (2004), 120 pp.Google Scholar
[10]Le Calvez, P.. Propriétés dynamiques des régions d’instabilité. Ann. Sci. Éc. Norm. Supér. 20 (1987), 443–464.Google Scholar
[11]Llibre, J. and Mackay, R.. Rotation vectors and entropy for homeomorphisms of the torus isotopic to the identity. Ergod. Th. & Dynam. Sys. 11 (1991), 115–128.CrossRefGoogle Scholar
[12]Misiurewicz, M. and Ziemian, K.. Rotation sets for maps of tori. J. Lond. Math. Soc. 40 (1989), 490–506.Google Scholar